A simple Factorial Diophantine equation (17x^2=9y!+2003)

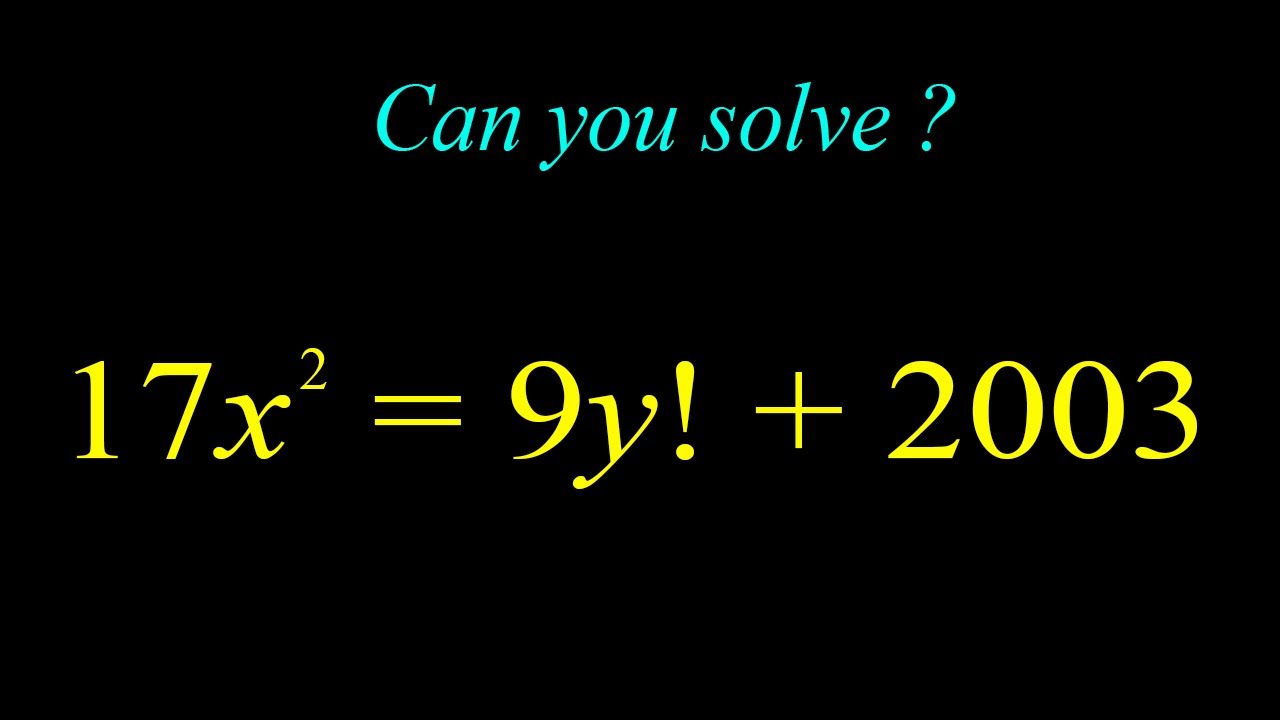
preview_player
Показать описание
A simple Factorial Diophantine equation (17x^2=9y!+2003)

Factorial Equation | Factorial Diophantine equation | Algebra | A simple Factorial Diophantine equation
  1. Mathematics MI
  2. A simple Factorial Diophantine equation
  3. factorial equation
  4. diophantine equations
  5. challenging math problems
  6. math
Рекомендации по теме
A simple Factorial 0:05:13

A simple Factorial Diophantine equation (17x^2=9y!+2003)

A Factorial Diophantine 0:05:04

A Factorial Diophantine Equation (n^2+19n-n!=0)

5 simple unsolvable 0:00:50

5 simple unsolvable equations

Learn How To 0:09:52

Learn How To Solve|Diophantine Factorial Equation|Math Olympiads

A Linear Diophantine 0:00:47

A Linear Diophantine Equation | 3x+4y=17

Diophantine Equation on 0:08:07

Diophantine Equation on Powers and Factorials | Singapore Mathematical Olympiad 2008

A Factorial Expression 0:00:32

A Factorial Expression

Solve Diophantine Equation 0:00:35

Solve Diophantine Equation by Factoring

A Diophantine Equation 0:09:40

A Diophantine Equation With Factorials

A Factorial Equation 0:00:48

A Factorial Equation | Math Olympiads

A Quick and 0:02:20

A Quick and Easy Diophantine Equation

A Quick and 0:08:01

A Quick and Easy Factorial Equation from Romania

A Factorial Equation 0:08:20

A Factorial Equation | Can you solve?

Factorial Equations Thank 0:00:05

Factorial Equations Thank You!!! 🤩

Solving an Intriguing 0:08:22

Solving an Intriguing Diophantine Equation with Factorials | Number Theory Challenge

When mathematicians get 0:00:37

When mathematicians get bored (ep1)

A Series on 0:00:31

A Series on Diophantine Equations - Please Subscribe to the Channel

A Linear Diophantine 0:00:27

A Linear Diophantine Equation

A Diophantine Equation 0:00:56

A Diophantine Equation | x^y=y^x

A Factorial Diophantine 0:05:04

A Factorial Diophantine Equation (n^2+19n-n!=0)

A Factorial Equation 0:00:44

A Factorial Equation | n! = n^2 + 19n

A Diophantine Equation 0:00:55

A Diophantine Equation | x^2-y^2=23

A Quick and 0:05:43

A Quick and Easy Factorial Equation

One fairly easy 0:03:30

One fairly easy diophantine equation anyone can solve | mathematica

Комментарии
Автор

Similar logic shows that the solution set's finite for mx^n = py! + q if m doesn't divide q, where m, n, p & q are all integers.

tedbagg
Автор

Great, actually looking at mod 4 for y>= 4 is already enough, since we have the nice fact that a^2 is congruent to 0, 1 mod 4 for all natural a.
Taking mod 4 reduces this to x^2 is congruent to y! +3, but y >= 4, this means y! is 0 mod 4, that means x^2 is 3 mod 4 which clearly means theres no positive integer solutions for x.

The above solution is motivated by the fact perfect squares mod 4 have a nice residue. Also y=3 works, and by testing 4, 5, 6…, motivates mod 4.
Nonetheless, great vid.

beautyofmath
Автор

How do you test for divisibility by 17 without using a calculator? I wouldn't have put it past Gauss, but if you have a rule, I would like to have it explained.

zh