Class 04 - Positive Definite Functions and Feature Maps

Показать описание

Lorenzo Rosasco, MIT, University of Genoa, IIT

Рекомендации по теме

Комментарии

I am repeating the calculations and it seems to me that the definitions of inner products up to minute 35 are slightly wrong.

For the spaces defined by a finite integral with a weighting factor in the measure, it is the factor itself (and not its inverse) that should appear in the associated inner product - this works fine by Cauchy-Schwarz.

Furthermore, since even the Fourier transforms of real functions can be complex, your inner product needs to be sesquilinear - you need to add a complex conjugate to the second entry in the integrals.

Finally - he discloses it - there is an overall disregard for constants when calculating Fourier transforms here, so take every expression with a grain of salt .

alemorita

The example of the RKHS of bandlimited functions, has an error in the definition. The sinc function, which is the kernel is not in L1(R), whereas it needs to be in the Hilbert space H. The L1(R) constraint needs to be replaced by continuity and appropriately interpreted.

GauravSharma

Hello MIT. Thank you for the great content !! Any chance the problem sets and/or the lecture notes could be made public ?

apoorvreddy

Class 04 - Positive Definite Functions and Feature Maps

Class 04 - Positive Definite Functions and Feature Maps

Is BᵀB Always Positive Definite? (Also, Messi makes a comeback!)

Theoretical Test on Positive Definite Matrices. Energy-based definition of Positive Definite Matrix.

Positive Definite Correlation Matrices | FRM Part 1 (Quantitative Analysis)

CSIR NET MATHEMATICS NOV 2020 | Linear Algebra | Positive Definite Quadratic Form

Positive Definite and Quadratic Forms(old)

POSITIVE DEFINITE SYMMETRIC MATRIX/VTU/4THSEM/ECE/ENGINEERING STATISTICS AND LINEAR ALGEBRA(ES&L...

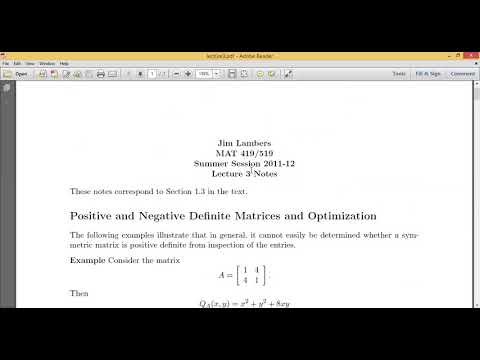
Positive Definite and Quadratic Forms

Msc-4th-lecture#12(What is positive definite matrix?)

Positive Definite Matrix - 2(SS)

Advanced Linear Algebra - Lecture 31: Sylvester's Criterion for Positive Definiteness

Problem on Positive definiteness||Sylvester's Condition

Pillai 'Cauchy Matrix and One of its Applications'

Advanced Linear Algebra - Lecture 30: Introduction to Positive (Semi)Definite Matrices

Uri Bader: Positive definite functions and characters 1 part b

NL 13 [A few basic Topics, Positive Definite Function, Class K Function]

Spectral Thm: Symmetric Matrices are Orthogonally Diagonalizable, Positive Definite Quadratic Form

Linear System Theory - 10 Hermitian matrices and positive definiteness

Sublinear Time Low-rank Approximation of Positive Semidefinite Matrices

Convex Functions and Positive Definite matrices. An Introduction

Positive Semidefinite Matrices

CLASS 03 POSITIVE AND SEMI POSITIVE DEFINITE MATRIX, TRACE, INVERSE, TRANSPOSE, IDEMPOTENT MATRICES

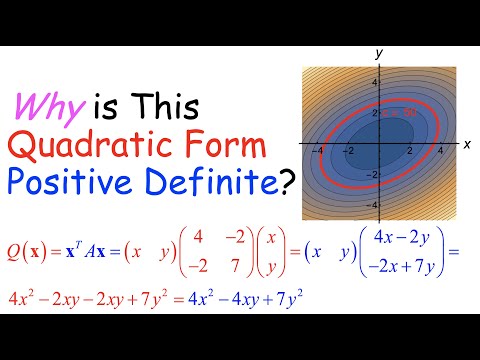
Quadratic form with a matrix

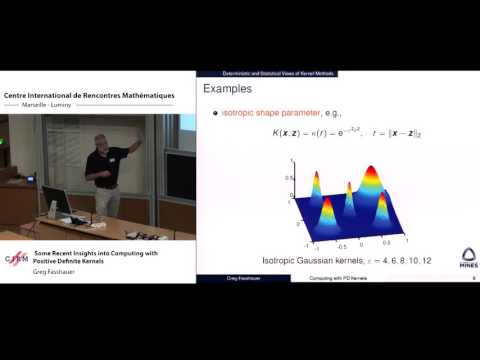
Greg Fasshauer: Some recent insights into computing with positive definite kernels