filmov
tv
Analytic Function and Singularity of a Function
Показать описание
Analytic Function and Singularity of a Function
Analytic Function
A mathematical function is said to be analytic at a point in a plane, if its value and its derivative has finite existence at that point.
Singularity of a Function
If at a point in plane, the value of function or its derivative is infinite, the function is said to be non-analytic at that point and such a point is called Singularity of the function.
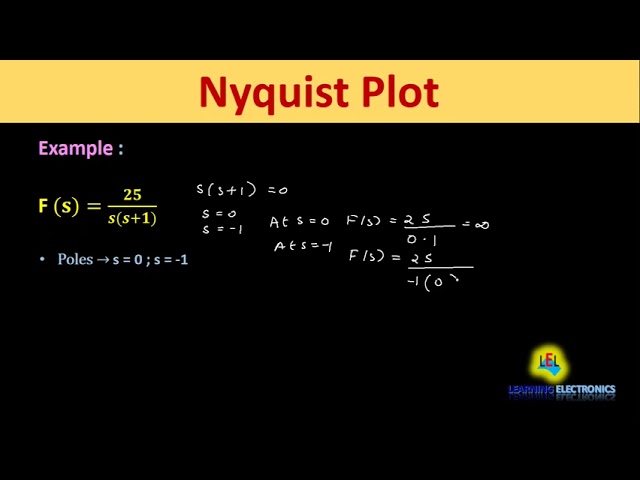
Example : F (𝐬)=𝟐𝟓/(𝒔(𝒔+𝟏))
This function is analytic at all points except s = 0 and s= -1 which are its poles.
Generally we can say that , Poles of a function are its singularities (∵ F(S) = ꚙ)
Single valued Function
A function F(s) is said to be single valued function if it has one and only one value for each separate values of ‘s’.
Consider : F(s) = √25
Such a function is not said to be single valued.
In control system we assume that transfer function of system or the functions G(s) and H(s) are single valued
Analytic Function
A mathematical function is said to be analytic at a point in a plane, if its value and its derivative has finite existence at that point.
Singularity of a Function
If at a point in plane, the value of function or its derivative is infinite, the function is said to be non-analytic at that point and such a point is called Singularity of the function.
Example : F (𝐬)=𝟐𝟓/(𝒔(𝒔+𝟏))
This function is analytic at all points except s = 0 and s= -1 which are its poles.
Generally we can say that , Poles of a function are its singularities (∵ F(S) = ꚙ)
Single valued Function
A function F(s) is said to be single valued function if it has one and only one value for each separate values of ‘s’.
Consider : F(s) = √25
Such a function is not said to be single valued.
In control system we assume that transfer function of system or the functions G(s) and H(s) are single valued
 0:11:25
0:11:25
 0:05:56
0:05:56
 0:08:22
0:08:22
 0:24:14
0:24:14
 0:10:04
0:10:04
 0:08:56
0:08:56
 0:05:11
0:05:11
 0:20:41
0:20:41
 0:25:38
0:25:38
 0:12:25
0:12:25
 0:27:37
0:27:37
 0:00:52
0:00:52
 0:37:01
0:37:01
 0:30:53
0:30:53
 0:27:14
0:27:14
 0:01:01
0:01:01
 0:01:00
0:01:00
 0:05:07
0:05:07
 0:18:34
0:18:34
 0:05:50
0:05:50
 0:10:04
0:10:04
 0:38:57
0:38:57
 0:16:05
0:16:05
 0:02:19
0:02:19