Proof Theory: From Arithmetic to Set theory

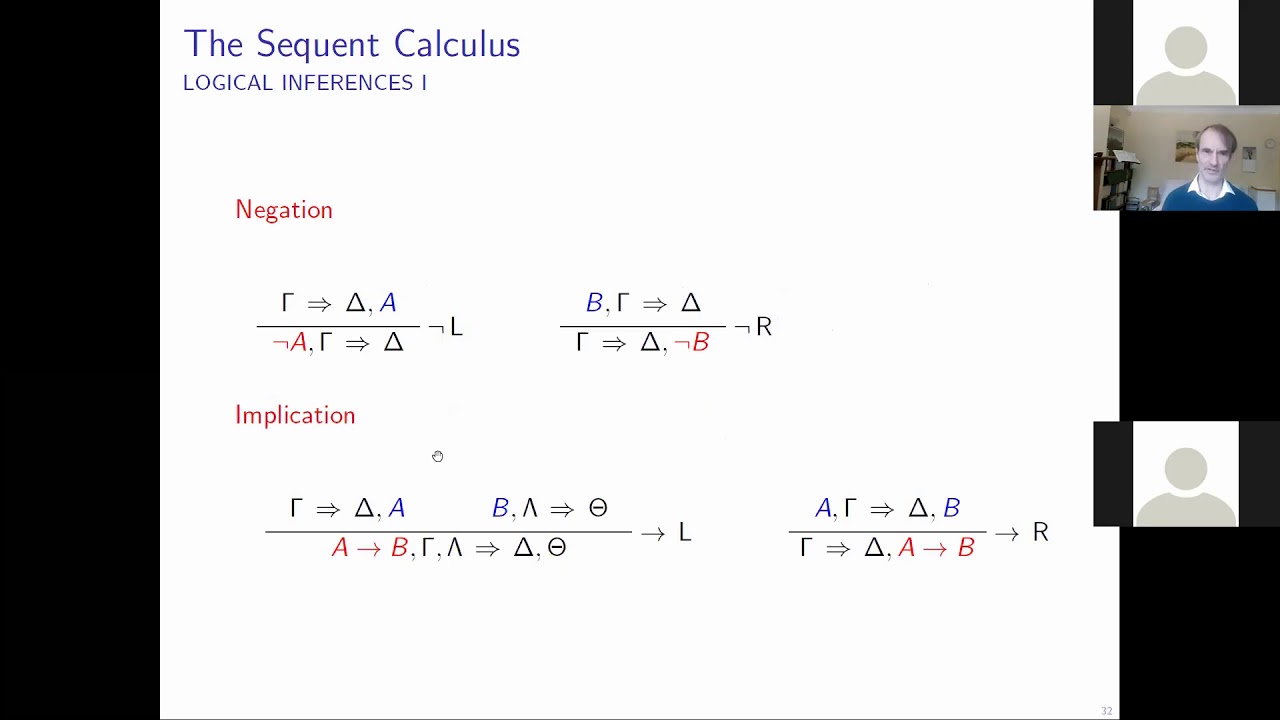
Abstract: A central theme running through all the main areas of Mathematical Logic is the classification of sets, functions or theories, by means of transfinite hierarchies whose ordinal levels measure their ‘rank’ or ‘complexity’ in some sense appropriate to the underlying context. In Proof Theory this is manifest in the assignment of ‘proof theoretic ordinals’ to theories, gauging their ‘consistency strength’ and ‘computational power’. This area of mathematical logic has is roots in Hilbert's “Beweistheorie”, the aim of which was to lay to rest all worries about the foundations of mathematics once and for all by securing mathematics via an absolute proof of consistency. In the main, modern proof theory came into existence in the 1930s, springing forth from Gentzen's work, especially his consistency proofs of arithmetic. The intent of the talk is to introduce the main notions and results of proof theory and to explain the rationale of ordinal-theoretic proof theory, advancing from arithmetic to set theory.

This is a video recording of a talk by Michael Rathjen in the

Online logic seminar on Foundations of Mathematics

on Oct. 13, 2020 presented via Zoom.

Interlocutor: Dr. Sam Sanders (TU Darmstadt, DE)
Organizer: School of Philosophy, Wuhan University (Wuhan, PRC)