JEE Advanced Physics 2014 Paper 1 #2 (#35) Wave, Speed, Frequency

To donate:

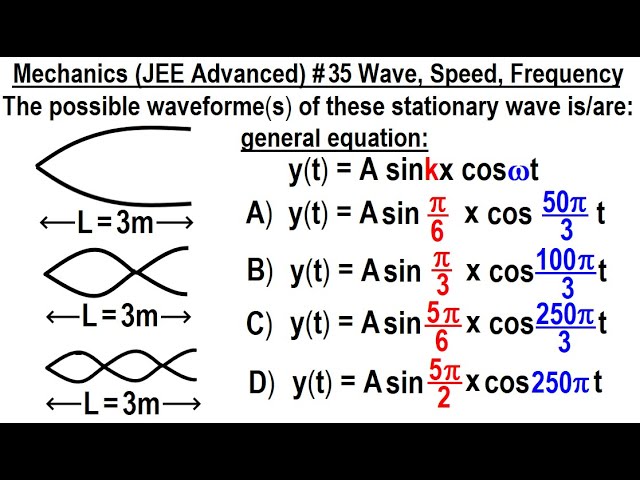
One end of a taut string of length 3m along the x-axis is fixed at x=0. The speed of the waves in the string is 100m/s. The other end of the string is vibrating in the y-direction so that stationary waves are set up in the string. The possible waveform(s) of these stationary waves is (are):
A) y(t)=A sin[ (pi)x/6] cos[ 50(pi)t/3)]
B) y(t)=A sin[5(pi)x/3] cos[100(pi)t/3)]
C) y(t)=A sin[5(pi)x/6] cos[250(pi)t/3)]
D) y(t)=A sin[5(pi)x/3] cos[250(pi)t)]

Previous video in this series can be seen at:
Next video in this series can be seen at:

[rbkstudios]

We are seriously gonna need a lot more of them
Thank you so much

[michaelduke]

Might have finished < 3min if it wasn't multiple choice.

[loughlan]

Dr. van Biezen, I enjoyed watching this video; it is a great exploration into string waves and harmonics. However, if you will excuse my forwardness, I would like to offer a possibly more expedient method for finding the values of k and omega. Instead of looking at the different harmonics and testing to see if they fit as a potential k or omega, why not change the equation around and solve for lambda, in both cases. A further explanation:
Observe that k = 2pi/lambda therefore, using two cancellations, lambda = 2 pi/k. Furthermore we can observe that lambda = s (3.0m) and that s must be an element of {4/(2n-1); n is an element of Z+}.
Therefore we can see that lambda = 2 pi/k can be expressed as (3) s = 2 pi/k or s = 2 pi/3 * 1/k.
Testing our new formula for pi / 6 results in:
answer A. s = 2 pi/3 * 6/pi => pi's cancel and 6 reduces to 2 => s = 4 or 4/1
ans B. s = 2 pi/3 * 3/pi => s = 2 or s = 4/2 which is not an element of the restricted set
ans C. s = 2 pi/3 * 6/5 pi => s = 4/5
ans D. s = 2 pi/3 * 2/5 pi => s = 4/15

A similar formula can be developed for the omegas using lambda = 200 pi/ w or using the above relation between lambda and s
s = 200 pi/3 * 1/w. This formula will yield the same s values as the wavenumber formula above. Thank you for your time, consideration, and awesome mathematical content.

[ImPresSiveXD]

how do you know that lambda is 4L, 4L/3, 4L/5 etc ?

[UniversaLLearningWithProshant]

Hi sir
Because your focus area is on electrical engineering and since I am also a electrical engineer student. So I request you to please make videos on
Question from previous year of
"GATE, ISRO, Indian Engineering Services, and UPSC EE Optional videos".
These are the top exams of Electrical student in India.