Higher Coleman theory. Vanishing theorems, slope estimates, control theorem I

Atelier: Théorie de Coleman supérieure et applications / Workshop: Higher Coleman theory and applications (8 déc. 2020 / Dec. 8, 2020)

George Boxer (ENS de Lyon, France)

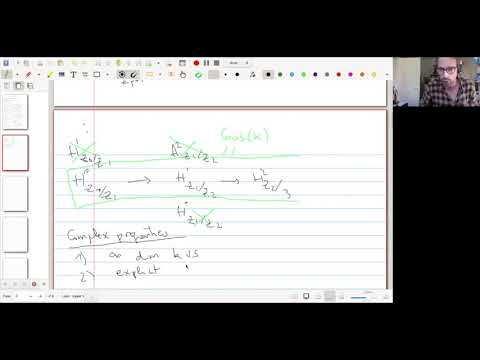
Abstract: Higher Coleman theory studies the finite slope part of coherent cohomology on Shimura varieties. We introduce a spectral sequence expressing the finite slope part of coherent cohomology in terms of certain overconvergent cohomologies. We prove a number of results on these overconvergent cohomologies : vanishing theorems, slope bounds, p-adic interpolation in the weight. As a corollary we deduce vanishing theorems for the small slope part of classical cohomology, prove classicality theorems, and construct (equidimensional) eigenvarieties. The goal of the course is to explain all this, with a particular emphasis on Siegel varieties (and even more specifically on modular curves and Siegel threefolds).