filmov
tv
A Nice Olympiad Trigonometric Exponential Problem | Find the value of X
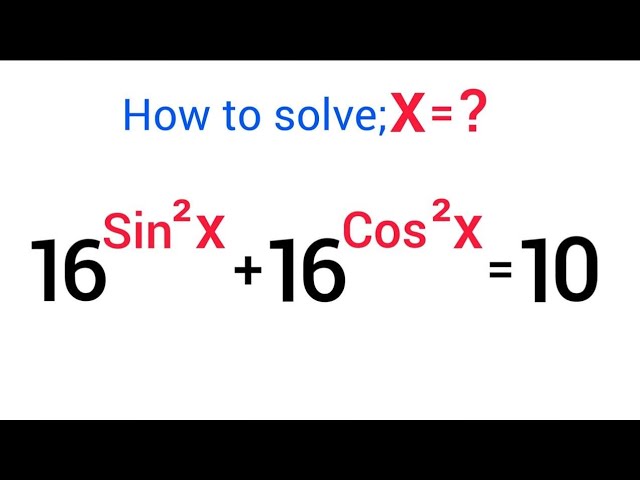
Показать описание
A Nice Olympiad Trigonometric Exponential Problem | Find the value of X
This is an interesting question with amazing concepts!
Hope you are well
#maths #algebra #matholympiads #matholympiad #olympiadexponentialproblem #olympiadmathexponentialproblems #aniceolympiadexponentialproblem #germanymatholympiadexponentialproblem #anicechineseolympiadexponentialproblem #averyniceolympiadexponentialproblem
Related Search:-
math problems, square root, simplify square root, math challenge, math puzzles, math tricks, educational video, math for kids, math tutorial, algebra, mathematics, solving square roots, square root examples, math exercises, brain teasers, critical thinking, problem-solving skills, learning math, math games, math skills, fun with math, math is fun, study math, math concepts, math techniques, square root rules, math education, math help, interactive math, math enthusiasts,
square root simplification, math solutions, algebraic expressions, challenge yourself, math quizzes, educational content, math exploration, square root problems, math learning, quick math, math strategies, rationalizing square roots, simplifying expressions, math fundamentals, study tips, math practice, problem-solving, engaging math, math community, learn algebra, mathematical concepts, arithmetic, mental math, critical reasoning, math mastery, high school math, middle school math, math homework, math for beginners, solve this puzzle, fun math problems
Playlist to watch all videos on
Mathisfun
GET IN TOUCH
FOLLOW US ON SOCIAL
Get updates or reach out to Get updates on our Social Media Profiles
This is an interesting question with amazing concepts!
Hope you are well
#maths #algebra #matholympiads #matholympiad #olympiadexponentialproblem #olympiadmathexponentialproblems #aniceolympiadexponentialproblem #germanymatholympiadexponentialproblem #anicechineseolympiadexponentialproblem #averyniceolympiadexponentialproblem
Related Search:-
math problems, square root, simplify square root, math challenge, math puzzles, math tricks, educational video, math for kids, math tutorial, algebra, mathematics, solving square roots, square root examples, math exercises, brain teasers, critical thinking, problem-solving skills, learning math, math games, math skills, fun with math, math is fun, study math, math concepts, math techniques, square root rules, math education, math help, interactive math, math enthusiasts,
square root simplification, math solutions, algebraic expressions, challenge yourself, math quizzes, educational content, math exploration, square root problems, math learning, quick math, math strategies, rationalizing square roots, simplifying expressions, math fundamentals, study tips, math practice, problem-solving, engaging math, math community, learn algebra, mathematical concepts, arithmetic, mental math, critical reasoning, math mastery, high school math, middle school math, math homework, math for beginners, solve this puzzle, fun math problems
Playlist to watch all videos on
Mathisfun
GET IN TOUCH
FOLLOW US ON SOCIAL
Get updates or reach out to Get updates on our Social Media Profiles
Комментарии
 0:18:54
0:18:54
 0:11:30
0:11:30
 0:08:57
0:08:57
 0:08:21
0:08:21
 0:07:43
0:07:43
 0:09:44
0:09:44
 0:11:43
0:11:43
 0:12:22
0:12:22
 0:09:56
0:09:56
 0:30:00
0:30:00
 0:09:48
0:09:48
 0:07:05
0:07:05
 0:09:00
0:09:00
 0:09:07
0:09:07
 0:15:13
0:15:13
 0:05:50
0:05:50
 0:07:39
0:07:39
 0:13:21
0:13:21
 0:06:12
0:06:12
 0:10:55
0:10:55
 0:13:13
0:13:13
 0:07:37
0:07:37
 0:06:39
0:06:39
 0:06:49
0:06:49