filmov
tv
Calculus 2, Lec 27B, Taylor Series on Mathematica, Taylor Series Calculation Tricks

Показать описание
Calculus 2, Lecture 27B.
(0:00) Fix the Mathematica code from Lecture 27A and make an animation of Taylor polynomial approximations to 1/(1+x^2).
(1:41) Check the other Taylor series from Lecture 27A.
(3:16) Taylor series for 1/((1+x^2)^2) centered at a = 0 found in two ways, one of which is by squaring the series for 1/(1+x^2).
(8:24) Write the Taylor series for sin(x) and cos(x) centered at a = 0.
(11:42) Find the first three nonzero terms of the Taylor series for tan(x) (tangent) by using long division to divide the series for sin(x) by the series for cos(x) and check with Series on Mathematica.
(17:34) Use substitution and multiplication to find the Taylor series for x^2*sin(x^3) centered at a = 0.
(19:47) Taylor series for e^(x) and a hint that exponential and trigonometric functions are related via complex numbers.
(21:43) Mathematica animation of Taylor polynomials for cosine function converging to the cosine function.
(23:57) Binomial series Taylor series expansion about a = 0 and application to finding the Taylor series for 1/((8+x)^(1/3)).
(0:00) Fix the Mathematica code from Lecture 27A and make an animation of Taylor polynomial approximations to 1/(1+x^2).
(1:41) Check the other Taylor series from Lecture 27A.
(3:16) Taylor series for 1/((1+x^2)^2) centered at a = 0 found in two ways, one of which is by squaring the series for 1/(1+x^2).
(8:24) Write the Taylor series for sin(x) and cos(x) centered at a = 0.
(11:42) Find the first three nonzero terms of the Taylor series for tan(x) (tangent) by using long division to divide the series for sin(x) by the series for cos(x) and check with Series on Mathematica.
(17:34) Use substitution and multiplication to find the Taylor series for x^2*sin(x^3) centered at a = 0.
(19:47) Taylor series for e^(x) and a hint that exponential and trigonometric functions are related via complex numbers.
(21:43) Mathematica animation of Taylor polynomials for cosine function converging to the cosine function.
(23:57) Binomial series Taylor series expansion about a = 0 and application to finding the Taylor series for 1/((8+x)^(1/3)).
 0:32:27
0:32:27
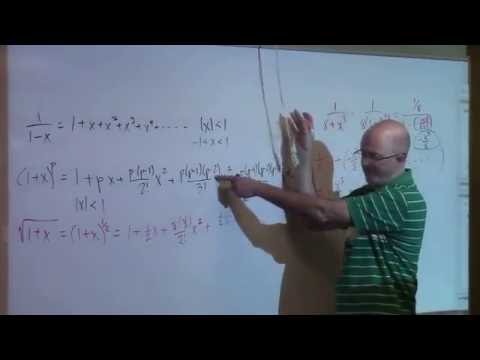 0:19:30
0:19:30
 0:33:28
0:33:28
 0:35:59
0:35:59
 0:20:25
0:20:25
 0:02:10
0:02:10
 0:07:35
0:07:35
 0:12:32
0:12:32
 0:33:02
0:33:02
 0:56:44
0:56:44
 0:35:34
0:35:34
 0:30:47
0:30:47
 0:01:25
0:01:25
 0:08:02
0:08:02
 0:30:31
0:30:31
 0:32:47
0:32:47
 0:06:01
0:06:01
 0:21:51
0:21:51
 0:30:52
0:30:52
 0:06:46
0:06:46
 0:32:40
0:32:40
 0:59:51
0:59:51
 0:28:08
0:28:08
 0:55:19
0:55:19