filmov
tv
Algebra I STAAR 2022: Question 44: Matching Exponential Function Equations to a Given Table
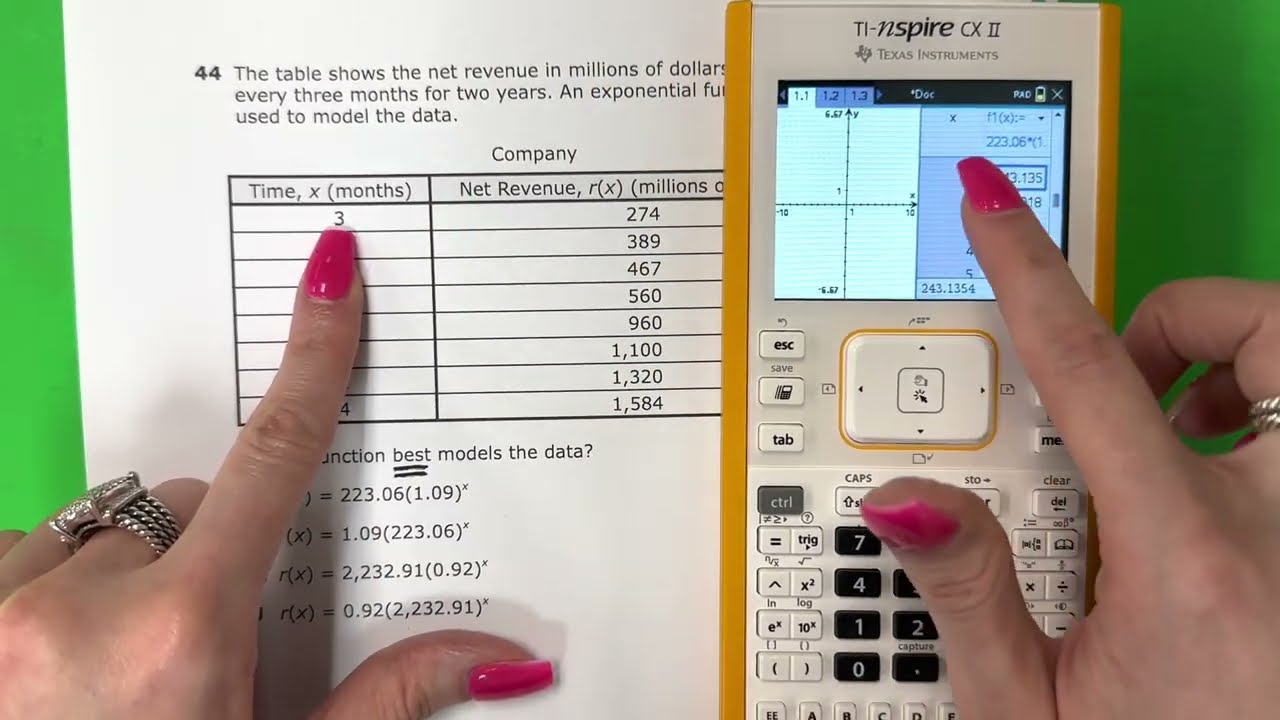
Показать описание
In this video, I will use my TI-Nspire calculator to find which exponential function best models the data shown in a table. Remember: the BEST does not always mean PERFECT!
How should you study? Watch the videos, follow along with me using your calculator, copy the notes at the end.
How should you study? Watch the videos, follow along with me using your calculator, copy the notes at the end.
STAAR Algebra 1 Test May 2022 (questions 1 - 54)
Algebra I STAAR 2022: Question 1: Finding Intercepts From a Graph
Algebra I STAAR 2022: Question 19: Rate of Change / Slope
Algebra I STAAR 2022: Question 2: Finding Slope & Rate of Change From a Table
Algebra I STAAR 2022: Question 5: Solving Systems of Equations by Hand and with a TI-Nspire
Algebra I STAAR 2022: Question 45: Which Graph Represents the System of Equations
Algebra I STAAR 2022: Question 17: Solution to a Quadratic Equation / Using the Quadratic Formula
Algebra I STAAR 2022: Question 10: Multiplying Binomials
2022 STAAR Algebra 1 EOC | Questions 21-25
2022 STAAR Algebra 1 EOC: Questions 1-5
Algebra I STAAR 2022: Question 24: Solutions of a Quadratic Function
Algebra I STAAR 2022: Question 12: Creating an Equation in Slope-Intercept Form from Table & Gra...
Algebra I STAAR 2022: Question 40: Matching an Equation to a Graph
Algebra I STAAR 2022: Question 42: Solving for a Variable / Solving Multi-Step Equations
Algebra I STAAR 2022: Question 22: Matching a Quadratic Function to a Table
2022 STAAR Algebra 1 EOC: Questions 6-10
Algebra I STAAR 2022: Question 43: Factoring in the Form x^2+bx+c (36m^2 - 100)
Algebra I STAAR 2022: Question 23: Adding Polynomials
Algebra I STAAR 2022: Question 44: Matching Exponential Function Equations to a Given Table
2022 STAAR Algebra 1 EOC: Questions 11-15
Algebra I STAAR 2022: Question 25: Slope-Intercept Form from Two Points on a Graph
Algebra I STAAR 2022: Question 41: Geometric Sequence
Algebra I STAAR 2022: Question 14: Factoring in the Form x^2+bx+c (d^2 - d - 6)
Algebra I STAAR 2022: Question 33: Factoring in the Form ax^2+bx+c
Комментарии
 1:46:02
1:46:02
 0:03:34
0:03:34
 0:05:05
0:05:05
 0:05:43
0:05:43
 0:07:45
0:07:45
 0:14:45
0:14:45
 0:07:07
0:07:07
 0:03:27
0:03:27
 0:03:54
0:03:54
 0:06:10
0:06:10
 0:06:40
0:06:40
 0:11:18
0:11:18
 0:02:42
0:02:42
 0:05:55
0:05:55
 0:04:34
0:04:34
 0:05:40
0:05:40
 0:13:43
0:13:43
 0:05:06
0:05:06
 0:07:36
0:07:36
 0:04:21
0:04:21
 0:06:45
0:06:45
 0:05:50
0:05:50
 0:05:15
0:05:15
 0:11:32
0:11:32