filmov
tv
Algebra - Ch. 7: Factoring Practice Problems (5 of 21) Example 4
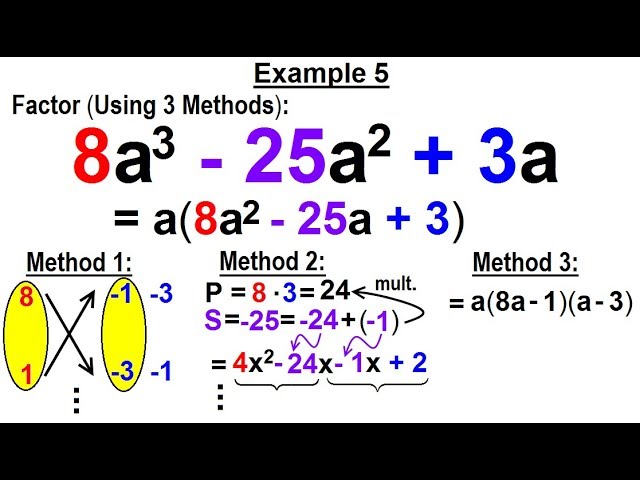
Показать описание
In this video I will use 3 different methods (trial/error/guessing, product-sum, and foil method) to factor 8a^3-25a^2+3a. Example 4
To donate:
.
Next video in this series can be seen at:
Algebra - Ch. 7: Factoring Practice Problems (1 of 21) How to Determine Which Technique to Use?
Algebra - Ch. 7: Factoring Practice Problems (2 of 21) Example 1
Algebra - Ch. 7: Factoring Practice Problems (4 of 21) Example 3
Algebra - Ch. 7: Factoring Practice Problems (17 of 21) Example 16
Algebra - Ch. 7: Factoring Practice Problems (10 of 21) Example 9
How To Factor Polynomials The Easy Way!
Algebra - Ch. 7: Factoring Practice Problems (16 of 21) Example 15
Algebra - Ch. 7: Factoring Practice Problems (20 of 21) Example 18
Algebraic Identities - Concepts & Examples (Part 1) Polynomials | Class 9 Maths Chapter 2 #cbse
Algebra - Ch. 7: Factoring Practice Problems (3 of 21) Example 2
Factoring Trinomials & Polynomials, Basic Introduction - Algebra
Algebra - Ch. 7: Factoring Practice Problems (14 of 21) Example 13
Algebra - Ch. 7: Factoring Practice Problems (9 of 21) Example 8
Algebra - Ch. 7: Factoring Practice Problems (15 of 21) Example 14
Algebra - Ch. 7: Factoring Practice Problems (6 of 21) Example 5
Algebra - Ch. 7: Factoring Practice Problems (5 of 21) Example 4
Algebra - Ch. 7: Factoring Practice Problems (19 of 21) Example 17
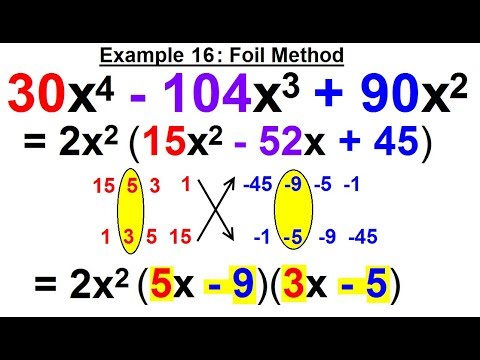
Algebra - Ch. 7: Factoring Practice Problems (18 of 21) Example 16: Foil Method
Algebra - Ch. 7: Factoring Practice Problems (7 of 21) Example 6
Algebra 1 Chapter 7 Factoring Quadratics
Algebra - Ch. 7: Factoring Practice Problems (8 of 21) Example 7
Algebra - Ch. 7: Factoring Practice Problems (13 of 21) Example 12
Algebra - Ch. 7: Factoring Practice Problems (21 of 21) Example 19
Algebra - Ch. 7: Factoring Practice Problems (12 of 21) Example 11
Комментарии
 0:04:00
0:04:00
 0:01:34
0:01:34
 0:06:24
0:06:24
 0:05:36
0:05:36
 0:02:39
0:02:39
 0:11:54
0:11:54
 0:06:10
0:06:10
 0:02:09
0:02:09
 0:50:50
0:50:50
 0:07:50
0:07:50
 0:34:46
0:34:46
 0:03:27
0:03:27
 0:02:21
0:02:21
 0:02:56
0:02:56
 0:01:36
0:01:36
 0:06:38
0:06:38
 0:03:01
0:03:01
 0:03:38
0:03:38
 0:01:34
0:01:34
 0:40:44
0:40:44
 0:03:15
0:03:15
 0:03:05
0:03:05
 0:03:56
0:03:56
 0:01:12
0:01:12