filmov
tv
Real Analysis Ep 20: Heine-Borel Theorem
Показать описание
Episode 20 of my videos for my undergraduate Real Analysis course at Fairfield University. This is a recording of a live class.
This episode is some more about the Heine-Borel Theorem.
This episode is some more about the Heine-Borel Theorem.
Real Analysis Ep 20: Heine-Borel Theorem
Real Analysis ep20: Heine-Borel Theorem (Oct 25, 2022)
Real Analysis 14 | Heine-Borel Theorem
Heine- Borel Theorem complete proof Of Real Analysis-1 bs math 5th semester
Real Analysis | The Heine-Borel Theorem
Real Analysis Lecture 5.3 Heine-Borel Theorem
Real Analysis 14 | Heine-Borel Theorem [dark version]
Real Analysis Lecture 7: Heine-Borel Theorem
The Heine-Borel Theorem on the Real Line
Heine Borel Theorem ( B.Sc. Level )
Heine - Borel theorem
402.3X5 Compact, Closed, and Bounded: The Heine-Borel Theorem
Heine Borel Theorem
MTH631Topic81 Real Analysis II Heine-Borel Theorem
Real Analysis | Heine Borel Theorem | Closed & Bounded set is Compact #HeineBoreltheorem #Compac...
Real Analysis: Heine Borel Theorem
The Heine-Borel Covering Theorem - Real Analysis | Lecture 8
Heine-Borel Theorem
L-8 Heine Borel Theorem || Real Analysis || B.Sc 2nd-year Maths
An Introduction to Compact Sets
Heine–Borel theorem | Real Analysis II
Another proof of Heine Borel theorem for the set of Real numbers
Visual Proof of The Heine-Borel Theorem and Compactness
More Compact set and Heine Borel theorem
Комментарии
 0:50:06
0:50:06
 0:49:09
0:49:09
 0:06:50
0:06:50
 0:01:04
0:01:04
 0:17:56
0:17:56
 0:26:45
0:26:45
 0:06:37
0:06:37
 0:13:32
0:13:32
 0:06:14
0:06:14
 0:57:12
0:57:12
 0:00:30
0:00:30
 0:16:38
0:16:38
 0:27:20
0:27:20
 0:10:52
0:10:52
 0:03:54
0:03:54
 0:22:20
0:22:20
 0:40:27
0:40:27
 0:31:59
0:31:59
 0:15:17
0:15:17
 0:11:13
0:11:13
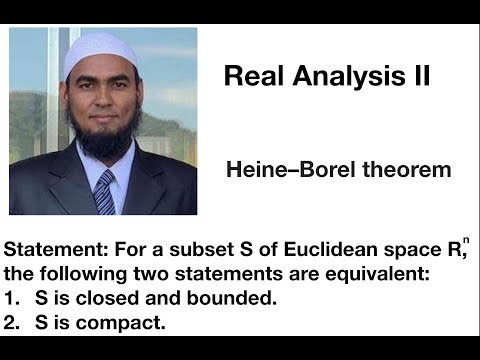 0:41:04
0:41:04
 0:24:25
0:24:25
 0:46:09
0:46:09
 0:32:03
0:32:03