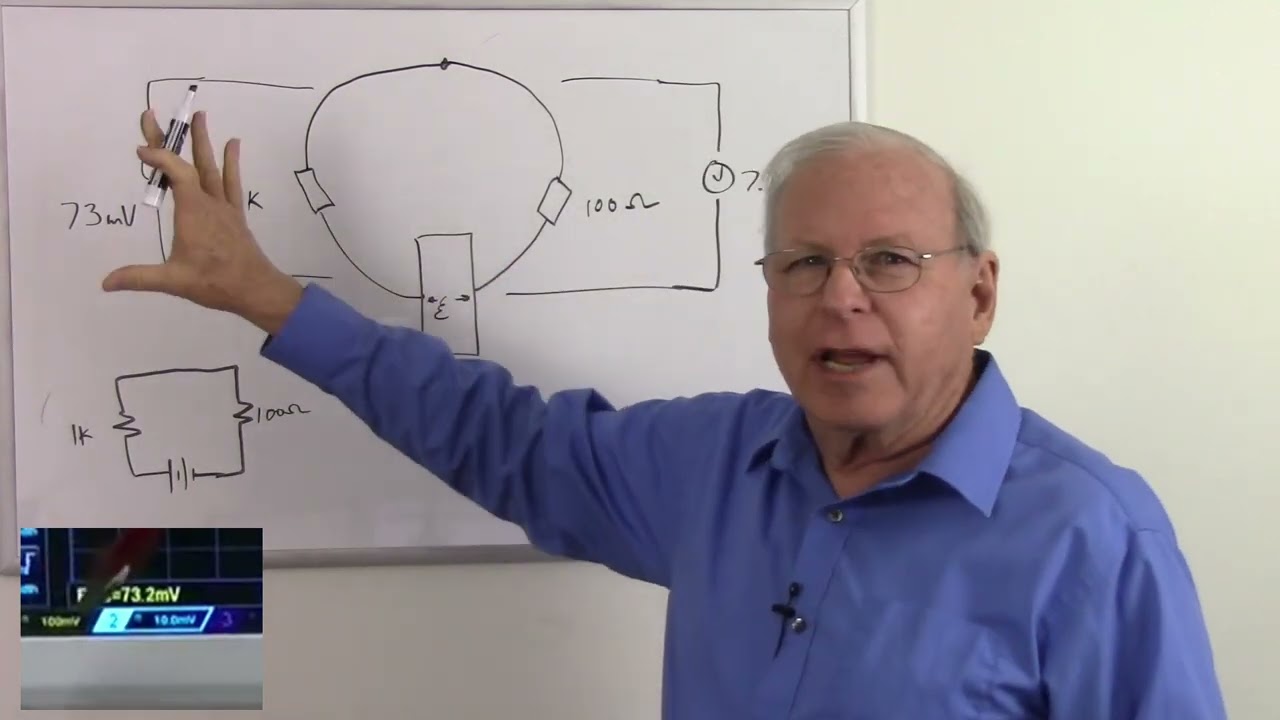
KVL and Induction One Last Lash at the Dead Horse

Vocademy - free vocational education

Here are the videos referred to in this video:

Vocademy is a free online vocational school, and we rely on free-will donations to operate. You can be a big help for as little as $12.00 per year. Please go to our support page to see how to make a monthly pledge or a one-time donation.

[SiliconSoup]

You are very sincere in pursuing the knowledge of science, and I really respect that. I hope this is not your last video on KVL. You have now accepted that voltage along the wire is zero, but not along that small segment inside the torus. This is like saying the general form of ohms law, J=sigma E does not apply to that segment, and that Maxwell equations are wrong. My video on KVL is to explain how the voltage can be zero along the wire, and yet non-zero across the terminals. For some reasons you didn't watch it carefully, and so you couldn't get around with voltage being path-dependent in changing magnetic field. I want to invite you to watch my video again. Everything I said in the video was not just based on intuition, but based on rigorous equations and calculation, as demonstrated with my numerical calculation.

[trevorkearney]

You were looking for some background information about the electric field distribution. One useful document for you to consider might be the following paper which can be downloaded from the internet.

Edwards, J. & Saha, Tapan. (2011). Power flow in transformers via the Poynting vector.
When referring to the mutual flux in a two-limb transformer linking primary and secondary windings Edwards & Saha state:
“Most of this flux is guided by the horizontal limbs to the secondary winding so that the voltage/turn (i.e. integral of E) around the secondary limb is only slightly less than that around the primary. The E fields due the changing flux in the upper and lower limbs tend to reinforce each other in the gap between the two limbs and cancel each other in the space beyond. Hence the E field is not uniformly distributed around the limbs, but concentrated into the space between them.”
They later state when referring to the power flow via the Poynting vector between the windings of a toroidal transformer:
“Most of the power (P) will flow in the inner space of the toroid where the E field is greatest, but a significant amount will also flow around the outer region of the windings particularly if the inner diameter of the toroid is greater than half that of the outer.”
So the authors only state that the E field is at its maximum within the core window, rather than being exclusively confined there and that as the core proportions change, the field distribution external to the core window alters in response. Imagine a toroidal core with proportions on the scale of a bicycle inner tube. The result would be very different to the case where the core has proportions more akin to that of a donut.
This can presumably be verified by calculation, but the outcome would presumably depend on the toroidal geometry and the routing of the conductor path through the toroid window.

[copernicofelinis]

There is no EMF localized somewhere in the part of loop that is inside the toroidal core. You can verify it by sliding the voltmeter's probe along the ring until they touch.
The reason you cannot find anything in literature that confirms your "theory" is because it is wrong.

[trevorkearney]

In the comments below LauncestonCastle asks an important question (objection) about the E field and asserts that the induced e.m.f can only occur in the core window as that is the only place where the magnetic field can interact with a conductor. 
Regarding the latter assertion about the presence of a magnetic field [B] confined to the core window, it is well established in the literature that in the case of an ideal toroidal winding carrying a DC current, the magnetic field is confined entirely within the toroidal internal volume and is absent in the space surrounding the toroid - including the core window. Even where the primary current producing the toroidal B field is time-varying, the external B field is present in the quasi-static situation but is orders of magnitude less than the B field within the toroidal volume proper. This can readily be established experimentally using a B field probe and applying a time-varying current to the toroidal primary winding. Perhaps LauncestonCastle is confusing the concept of flux cutting with that of flux linking. Flux cutting is typical of situations where there is relative motion between a conductor and a static magnetic field. Flux linking is typical of Faraday induction associated with a time-varying magnetic field - such as in a transformer.
Regarding LauncestonCastle's objection about the point of mentioning an E field. One is tempted to ask what is the origin of the motive force which compels electric charge to traverse a conductor path i.e. what causes an electric current to "flow"? Surely this must be an electric field. In the quasi-static case of the ideal toroid where there is a negligible external time-varying B field, the generally accepted approach is to attribute induced secondary current flow to the time-varying magnetic vector potential [denoted A]. Unlike the B field, the magnetic vector potential [A] external to the toroid volume is substantial and readily accounts for the induced E field giving rise to current flow in the secondary loop. The vector potential is not confined to the core window but rather exists everywhere in the space external to the toroid internal volume.
As a final note regarding the external B field, the essence of the Aranhov-Bohm effect is that it is the vector potential that is relevant and the use of a toroidal field to test the effect is desirable as it ensures the condition of there being a negligible external B field in the space external to the torus boundary.

[trevorkearney]

It is illogical (on at least two counts) to argue that there is a ”voltage gradient” along a portion of an open conductor “loop” partly enclosing an energised solenoid winding.
Let's adopt Romer's approach and assume for the purposes of analysis, that the solenoid flux is changing at a constant rate of alpha Webers per second - see Romer's paper equation (2). In that case, applying Faraday's Law would lead to the conclusion that the induced emf in a closed loop would be a constant value whilst the adopted flux condition persists.
Returning then to the case of an open conductor.
Firstly, were a voltage gradient to exist along a portion or all of the open path, there would have to be a non-zero electric field acting longitudinally throughout the loop. Maxwell's integral equation mandates this - voltage is defined by the line integral of an electric field. Using Romer's flux model, this non-zero electric field would have to equate to the superposition of the constant Faraday induced component and any surface charge gradient changes along the surface of the electrically neutral conductor forming the open loop. If there were a non-zero resultant electric field along the wire, the initial charge realignment would not have reached steady-state and would persist until the resultant field reduced to zero. The non-zero field would continue to push unbound electric charges unidirectionally along the wire surface. This would imply an indefinite or unbounded increase in the potential difference arising at the open loop end points - an impossible outcome, since only a finite value is possible based on Faraday's Law. Ask yourself - "What physical condition limits an unbounded increase in charge accumulation at the open loop end points?"
The second point to note is that whatever induction takes place along the partial loop segment being observed would also take place in the measuring instrument (e.g. a CRO) connected across that segment. This must be true if there is negligible magnetic flux external to the solenoid boundary. The induction along the loop segment and the measurement path segment would cancel one another. The measurement path together with the partial loop segment creates a closed loop in which any additional emf induction could only occur if that loop itself encloses time-varying magnetic flux external to the solenoid boundary. I suggest this very condition led to your supposed observation of a voltage gradient along the open loop adjacent to the "solenoid" you used in your experiment and detailed elsewhere.

[LauncestonCastle]

If we are talking about electromagnetic induction, why are we considering the E field? In addition there can only be an induced e.m.f, where a magnetic field interacts with a conductor i.e. within the toroid in this case. The wiring outside the toroid is just connecting wire.

[leonhardtkristensen]

I think you do have EMF on the outside too but just so small compared to what is inside that we can't measure it. A magnetic probe for the oscilloscope should be possible to confirm. I do have some and also a toroid transformer so I should be able to test it but it is a little difficult as I don't have a laboratory and I am walking with a walker only and everything is difficult - not just a 5 min job as it should be in a laboratory.