filmov
tv
mod12lec71 - Differentiation theorems: Almost everywhere differentiability for Monotone and Bounded

Показать описание
DIfferentiation theorems: Almost everywhere differentiability for Monotone and Bounded Variation functions - Part 1
Monotonically non-decreasing functions, Functions of bounded variation (BV), Second fundamental theorem of Calculus, Examples and Non-examples of BV functions, BV functions as a difference of monotonically non-decreasing functions
Monotonically non-decreasing functions, Functions of bounded variation (BV), Second fundamental theorem of Calculus, Examples and Non-examples of BV functions, BV functions as a difference of monotonically non-decreasing functions
mod12lec71 - Differentiation theorems: Almost everywhere differentiability for Monotone and Bounded
mod12lec72 - Differentiation theorems: Almost everywhere differentiability for Monotone and Bounded
DIfferentiation theorems: Almost everywhere differentiability for Monotone and Bounded Variation
Mod12lec71
Differentation theorem for general monotone functions and Second fundamental theorem of calculus
Differentiation theorem for monone continuous functions
Lebesgue theorem
If f is function of bounded variation then f is differential almost everywhere
Lec-42|Dini Derivatives|Prove left and right hand Derivative,If exists,is Measurable on an Interval|
mod11lec70 - Lebesgue's differentiation theorem: statement and proof - Part 2
Function of Bounded variation, Differentiation of an integral||Lebesgue theory ||maths||
Mod-14 Lec-42 Royden\'s Theorem on Normality Based On Growth Of Derivatives
Lec-3 f is function of bounded variation then F is differentiable and F`=f almost everywhere part -1
Dinis derivatives and LDT
Four Derivatives of Modulus function
Length of a curve formula, absolutely continuous curves
Real Analysis (MTH-RA) Lecture 15
Lec-2 if f is lebesgue Integrable then f =0 almost everywhere
Unit4 lebesque theorem and Jordan decomposition theorem
Lebesgue Integral || differential equations mathematics || Find Four Dini Derivatives at x=0.
Lebesgue Theorem - Measure and Integration
7.4 - Functions of bounded variation
UNIT 4 (part_1) PMA204
Every monotone functions are Riemann integrable and Sequential criterion for Riemann integration
Комментарии
 0:23:39
0:23:39
 0:17:12
0:17:12
 0:17:12
0:17:12
 0:27:24
0:27:24
 0:18:18
0:18:18
 0:31:52
0:31:52
 0:16:16
0:16:16
 0:02:22
0:02:22
 0:07:40
0:07:40
 0:31:43
0:31:43
 0:09:46
0:09:46
 0:36:04
0:36:04
 0:14:22
0:14:22
 0:30:32
0:30:32
 0:07:48
0:07:48
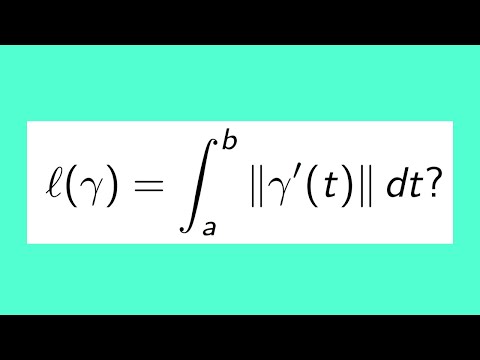 0:22:21
0:22:21
 1:57:03
1:57:03
 0:08:39
0:08:39
 0:30:34
0:30:34
 0:04:20
0:04:20
 0:23:08
0:23:08
 0:13:56
0:13:56
 0:19:49
0:19:49
 0:44:40
0:44:40