Calculus 1 Exam Review Part 2

In this live stream, we will review various problems likely to appear in your Final Exam.
1. Derivatives Rules: Sum, Difference, Product, Quotient, and Chain Rules
2. Tangent Line Equations
3. Logarithmic Differentiation
4. Implicit Differentiation
We will solve 25 problems during this review session.
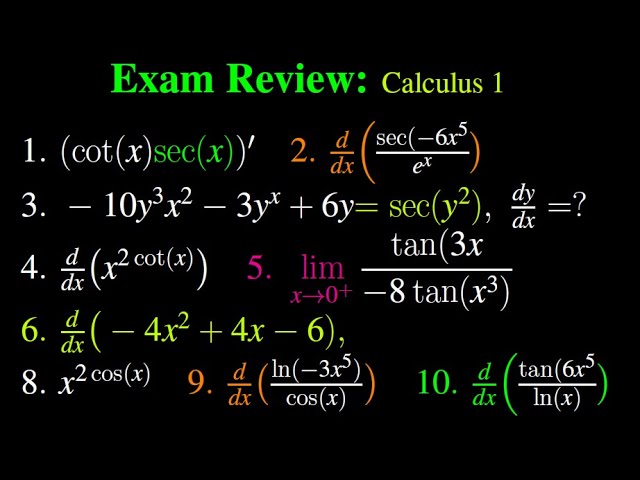
Problem 1: Find the derivative of f (x) = tan(x) log(x),
using derivative rules.
Problem 2: Find the derivative of f (x) =
log(−3x5)/cos(x).
Problem 3: y^3x^2+6y^2x-8y =tan(y^2), find dy/dx.
Problem 4: d/dx(-8x^5-3x^4-2x^3+x^2-9x-9). Find the tangent line at x=2.
Problem 5: Determine the derivative d/dx(tan(x)^cos(x))
Problem 6: Find the derivative of f (x) = csc(x) cot(x), using
derivative rules.
Problem 7: Find the derivative of f (x)= sec(5x^5)/e^x.
Problem 8: −4y^3x^2 − 5y^2x − 3y = tan(y^2). dy/dx=?
Problem 9: d/dx(− 7x^5 + 5x^4 − 10x^3 − x^2 + 8x − 7), Find the tangent line at x = 2.
Problem 10: Determine the derivative d/dx(tan(x)^cos(x))
Problem 11: Find the derivative of f (x) = log(x) cos(x), using
derivative rules.
Problem 12: Find the derivative of f (x) =log(7x^5)/sin(x)
Problem 13: −7y3x2 + 4y2x − 5y = cot(y2). Find dy/dx =?
Problem 14: d/dx(− 9x5 − 10x4 + x3 + 3x2 − 5x − 3).
Find the tangent line at x = 2.
Problem 15: Determine the derivative
d/dx(x^(2sin(x)))
Problem 16: Find the derivative of f (x) = tan(x) log(x),
using derivative rules.
Problem 17: Find the derivative of f (x) =tan(3x^5)/log(x)
Problem 18: y^3x^2 + 2y^2x − y = log(y^2). dy/dx=?
Problem 19: d/dx(6x5 − x4 − x3 + 4x2 − 8x + 7). Find the tangent line at x = 2.
Problem 20: Determine the derivative d/dx(tan(x)^cos(x))
Problem 21: Find the derivative of f (x) = e^x*tan(x), using
derivative rules.
Problem 22: Find the derivative of f (x) =log(-5x^5)/sin(x)
Problem 23: 7y3x2 + y2x + 9y = cot(y2). dy/dx=?
Problem 24: d/dx(− 10x^5 − 3x^4 + x^3 − 5x^2 − 9x − 1). Find the tangent line at x = 2.
Problem 25: Determine the derivative d/dx( sin(x)^cot(x)).