filmov
tv
Find the Constant Term In (x^2 - 2/x)^9 | Olympiad Math

Показать описание
We use the binomial formula to obtain the general term in the expansion of (x^2-2/x)^9. Then we find the correct k such that in the kth term, the power of the x term is zero.
Find The Constant Term in The Binomial Expansion Of (2x-7/x^2)^21
How to find Constant term in Binomial Expansion (x + 1/x)^6 using General Term
Binomial Expansion finding the constant term
Find the constant term in the expansion of (x^3- 2/x)^8
What Is a Trinomial With a Constant Term? : Algebra Help
Can you find the constant term?
How to Find the Constant Term in a Binomial Expansion | Polynomials | Algebra II | Mathacademy
Identifying the Constant Term in a Binomial Expansion
𝗗𝗲𝗳𝗶𝗻𝗶𝘁𝗲 𝗜𝗻𝘁𝗲𝗴𝗿𝗮𝘁𝗶𝗼𝗻 𝘄𝗶𝘁𝗵 𝗙𝗼𝘂𝗿𝘁𝗵 𝗣𝗼𝘄𝗲𝗿𝘀 𝗼𝗳 𝟮𝟬𝟮𝟰 𝗮𝗻𝗱 𝗹𝗶𝗺𝗶𝘁𝘀 -𝟮𝟬𝟮𝟱 𝘁𝗼 𝟮𝟬𝟮𝟱 ║ 𝗠𝗮𝘁𝗵𝟭𝟬𝟴𝟵...
Find value of k given constant term for expansion of (x^3/3+k/x)^12
Find the Constant Term In (x^2 - 2/x)^9 | Olympiad Math
Binomial Theorem (2 of 2: Finding the constant term in a binomial expansion)
FIND THE CONSTANT TERM - BINOMIAL EXPANSION TEASER #shorts #maths
Constant Term in Binomial Expansion (x/a + a^/x)^6 is 1280 Find a
Finding the constant term in an expansion (Part 1)
How to find the constant term in a binomial expansion?
Binomial expansions , finding the constant term Q6
How to find a constant term in binomial expansion.knec example.
Finding the constant term in an expansion (Part 2)
What Is a Trinomial With a Constant Term? : Algebra Help
Binomial Theorem Find Term independent of variable x
Binomial Theorem Example 5b - finding the constant term
Constant term in the expansion of `(x-1/x)^(10)` is a. `152` b. `-152` c. `-252` d. 252
In the expansion of (5x-3) (x + 2)^(2), find : constant term | 9 | EXPANSIONS | MATHS | ICSE | D...
Комментарии
 0:05:16
0:05:16
 0:02:54
0:02:54
 0:03:07
0:03:07
 0:04:34
0:04:34
 0:01:20
0:01:20
 0:01:29
0:01:29
 0:03:53
0:03:53
 0:07:37
0:07:37
 0:06:31
0:06:31
 0:05:52
0:05:52
 0:04:20
0:04:20
 0:06:09
0:06:09
 0:00:11
0:00:11
 0:05:38
0:05:38
 0:13:17
0:13:17
 0:05:02
0:05:02
 0:03:33
0:03:33
 0:04:28
0:04:28
 0:11:25
0:11:25
 0:01:19
0:01:19
 0:05:00
0:05:00
 0:06:31
0:06:31
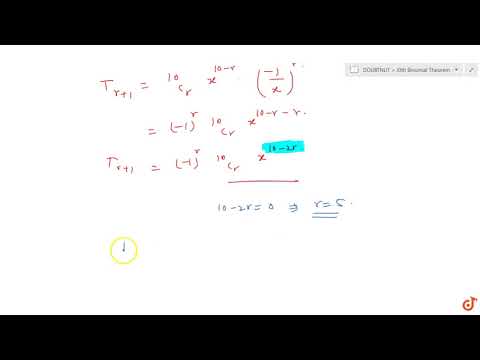 0:02:18
0:02:18
 0:01:39
0:01:39