filmov
tv
Equivalence Classes Partition a Set Proof

Показать описание
Equivalence Classes Partition a Set Proof. This video starts with the definition of an equivalence class and then proves that for a given set S and an equivalence relation R on S, we can write S as the disjoint union of it's equivalence classes. Every single detail is shown or explained in the proofs.
Equivalence Classes and Partitions
Equivalence Classes Partition a Set Proof
Important Math Proof: The Set of Equivalence Classes Partition a Set
Equivalence Classes and Partitions (Solved Problems)
Equivalence Classes
Partitions and Equivalence classes
Proof that equivalence classes form a partition
Equivalence Classes and Partitions
Abstract Algebra | Partitions and Equivalence Relations
Equivalence Classes and Partitions
Properties of Equivalence Classes (Partition)
11.4 equivalence classes and partitions.mp4
Equivalence Classes and Partitions (Preliminaries 03)
Prove with Partitions, Equivalence Classes
015 - Equivalence Classes and Partition of a Set
Discrete Math - 9.5.1 Equivalence Relations
(Abstract Algebra 1) Partitions and Equivalence Relations
Equivalence Classes and Partitions Intuition
Partitions and Equivalence Classes
Math 3000: Introduction to Partitions and Equivalence Classes
Proving the set of equivalence classes is a partition (ILIEKMATHPHYSICS)
Equivalence classes partition the set -- Proofs
Equivalence Classes and Partition
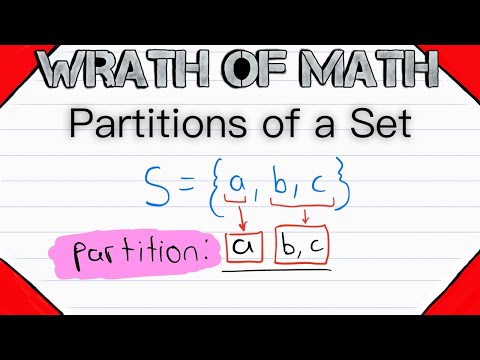
Partitions of a Set | Set Theory
Комментарии
 0:13:10
0:13:10
 0:15:26
0:15:26
 0:07:15
0:07:15
 0:08:45
0:08:45
 0:07:19
0:07:19
 0:07:29
0:07:29
 0:06:50
0:06:50
 0:15:26
0:15:26
 0:14:38
0:14:38
 0:14:35
0:14:35
 0:06:15
0:06:15
 0:14:59
0:14:59
 0:21:28
0:21:28
 0:10:28
0:10:28
 0:16:32
0:16:32
 0:22:30
0:22:30
 0:06:35
0:06:35
 0:11:49
0:11:49
 0:05:38
0:05:38
 0:26:19
0:26:19
 0:25:45
0:25:45
 0:49:16
0:49:16
 0:46:40
0:46:40
 0:07:59
0:07:59