filmov
tv
Stone's theorem & construction of observables - L12 - Frederic Schuller

Показать описание
This is from a series of lectures - "Lectures on Quantum Theory" delivered by Dr.Frederic P Schuller
Stone's theorem & construction of observables - L12 - Frederic Schuller
Stone's representation theorem for Boolean algebras
How Was Stonehenge Created? | Animated History
9.1 The Stone--Weierstrass theorem
Math of QM: 8.1. Stone-von Neumann Uniqueness Theorem: Formulation and Idea of Proof
The Ingenious Methods: Lever Theory and Casing Stones
Math of QM: 8.2. Stone-von Neumann Theorem: Properties of Operators W(s,t)
8. Retaining Walls
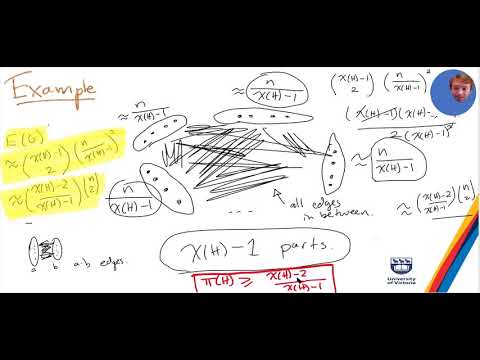
Extremal Combinatorics Lecture 14: Erdos Stone Theorem
Mystery of Inca's stone construction in Machu Picchu | Ed Barnhart and Lex Fridman
Lecture 20.1 - The Weierstrass Approximation Theorem
ACCL 2021 #10. Representation of Boolean algebras: finite & infinite Stone's theorem | Evge...
The Geopolymer Theory In Under 3 Minutes
'The Giza Power Plant' Christopher Dunn's Theory that The Great Pyramid Collected and...
Math 131 Spring 2022 042522 Stone Weierstrass Theorem. Introduction to analytic functions.
Eric Stachura - On Stone's Theorem for Strongly Continuous Semigroups
The Great Pyramid Power Plant Theory Actually Works | Billy Carson
Pyramid theory moving 50 ton stones #eypt #pyramids #shorts #interesting
Erdős–Stone Theorem. MATH 492/529 Extremal Combinatorics, University of Victoria.
MAST30026 Lecture 16: Stone-Weierstrass theorem (Part 2)
Stone–Weierstrass theorem | Wikipedia audio article
Stone–von Neumann theorem | Wikipedia audio article
Stone -von Neumann Theorem 1
Statue of Liberty Theory #scary #christianity #history #joerogan #theory
Комментарии
 1:52:25
1:52:25
 0:02:53
0:02:53
 0:01:45
0:01:45
 0:30:46
0:30:46
 0:16:30
0:16:30
 0:00:27
0:00:27
 0:21:32
0:21:32
 0:04:44
0:04:44
 0:53:10
0:53:10
 0:07:18
0:07:18
 0:15:04
0:15:04
 0:57:20
0:57:20
 0:02:49
0:02:49
 0:14:38
0:14:38
 1:03:19
1:03:19
 0:54:38
0:54:38
 0:08:20
0:08:20
 0:00:38
0:00:38
 1:19:35
1:19:35
 0:54:01
0:54:01
 0:21:25
0:21:25
 0:37:34
0:37:34
 0:37:24
0:37:24
 0:00:32
0:00:32