Hypothesis Testing for Two Population Means Dependent Samples

preview_player
Показать описание
This video shows how to perform a hypothesis test on a claim about the relationship between two population means when using data from a matched-pairs sample.
  1. FCMathGuy
Рекомендации по теме
Hypothesis Testing - 0:18:36

Hypothesis Testing - Difference of Two Means - Student's -Distribution & Normal Distributio...

Hypothesis test for 0:05:40

Hypothesis test for 2 Population Means using Excel’s Data Analysis

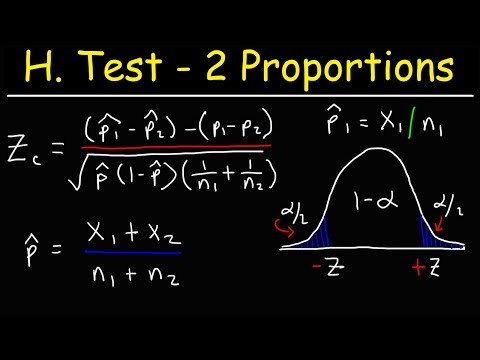Hypothesis Testing With 0:10:35

Hypothesis Testing With Two Proportions

Hypothesis Testing - 0:04:00

Hypothesis Testing - Introduction

Hypothesis Testing with 0:08:27

Hypothesis Testing with Two Proportions

Intro to Hypothesis 0:07:37

Intro to Hypothesis Tests for Two Population Means

Hypothesis Testing Problems 0:13:34

Hypothesis Testing Problems - Z Test & T Statistics - One & Two Tailed Tests 2

Hypothesis Test: Two 0:05:30

Hypothesis Test: Two Population Means with Unknown Standard Deviations (Right Tailed)

Interval Estimate for 0:03:39

Interval Estimate for Difference between Two Population Means | Biostatistics | BIO733_Topic120

Two-sample t test 0:06:56

Two-sample t test for difference of means | AP Statistics | Khan Academy

Hypothesis Test: Two 0:06:33

Hypothesis Test: Two Population Proportions

Hypothesis Testing Two 0:21:12

Hypothesis Testing Two Means Independent Samples

Hypothesis Testing - 0:09:58

Hypothesis Testing - Two Proportions

Hypothesis Test: Two 0:06:04

Hypothesis Test: Two Population Means with Unknown Standard Deviations (Data)

Chapter 10 Excel 0:05:54

Chapter 10 Excel Tutorial on Hypothesis Testing of Two Populations

Hypothesis test for 0:03:00

Hypothesis test for Equality of Two Variances | F-test

Hypothesis Testing 🔥 0:00:51

Hypothesis Testing 🔥 Explained in 60 Seconds

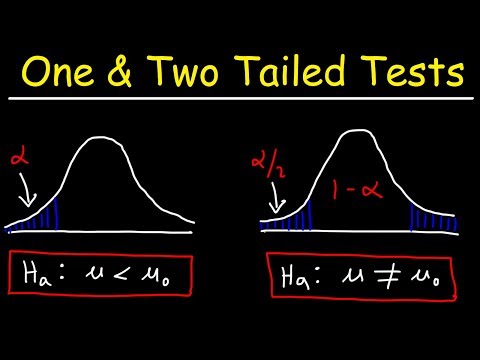One Tailed and 0:05:41

One Tailed and Two Tailed Tests, Critical Values, & Significance Level - Inferential Statistics

Inferences About Two 0:15:14

Inferences About Two Population Means, Sigma Known Part 1

Hypothesis Test: Two 0:05:18

Hypothesis Test: Two Population Means with Known Standard Deviations (Right Tailed)

Example- Confidence Interval 0:06:01

Example- Confidence Interval and Hypothesis Testing for Two Population Averages

Matched or Paired 0:12:56

Matched or Paired Samples T-Test - Hypothesis Testing

Lecture 55: Testing 0:18:53

Lecture 55: Testing hypothesis for differences between two population means with examples

Estimation and Hypothesis 0:16:50

Estimation and Hypothesis Testing for Two Population Means (Small Samples, Equal Variances)