Nested Hilbert schemes and DT theory of local threefolds

Artan Sheshmani (QGM Aarhus/Harvard CMSA) speaks on Nested Hilbert schemes and DT theory of local threefolds at the Center for Mathematical Sciences and Applications.

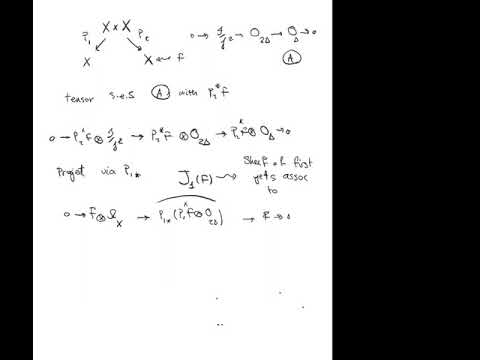
Abstract: Following talk 1 we study the geometry of S which is projective simply connected complex surface and we let L be a line bundle on S. We study moduli space of stable compactly supported 2-dimensional sheaves on the total spaces of L. The moduli space admits a \C^*-action induced by scaling the fibers of L. We identify certain components of the fixed locus of the moduli space with the moduli space of torsion free sheaves on S and the nested Hilbert schemes on S. We define reduced Donaldson-Thomas invariants by virtual localization. When L is the canonical bundle of S these reduced DT invariants recover Vafa-Witten invariants defined by Tanaka-Thomas via the moduli space of Higgs pairs on S. Vafa-Witten invariants are expected to have modular properties predicted by S-duality. We further show that in this case, in combination with Mochizuki's formulas, we are able to express these equivariant Donaldson-Thomas invariants on the total space of canonical bundle of S in terms of our invariants of nested Hilbert schemes in talk 1, the Seiberg-Witten invariants of S, and the integrals over the products of Hilbert schemes of points on S.