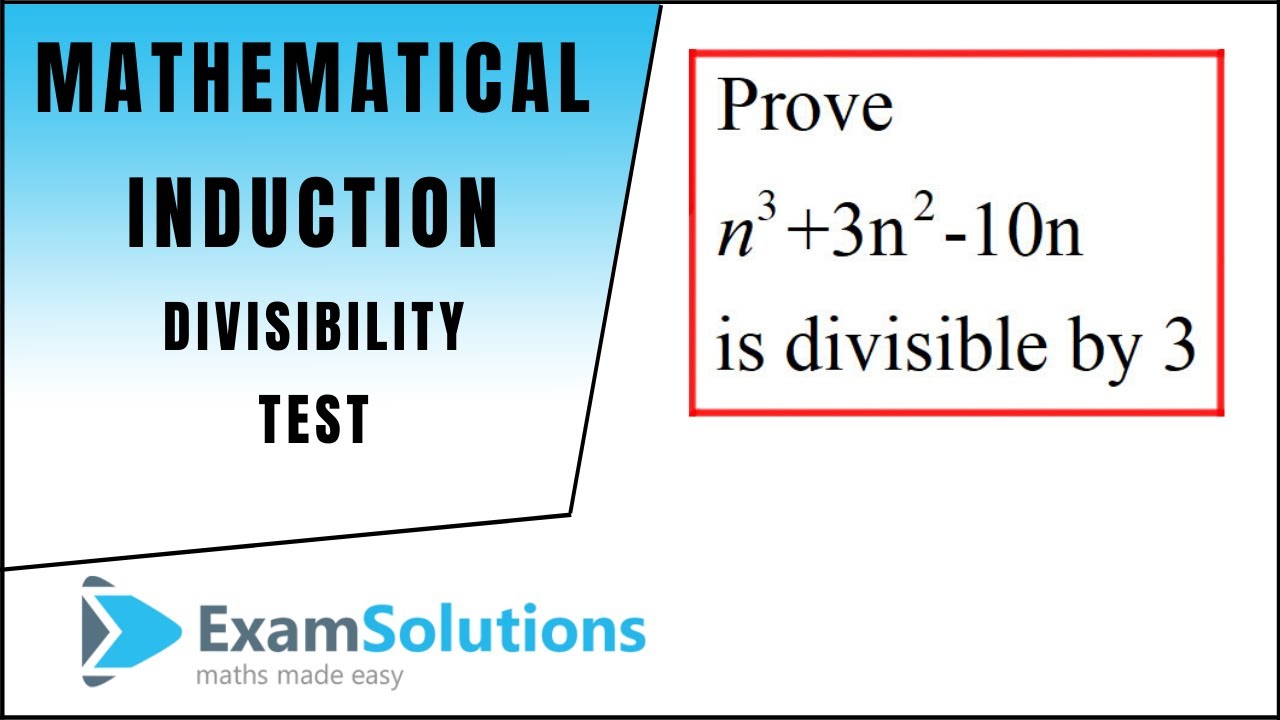
Mathematical Induction - Divisibility Tests (2) | ExamSolutions

Another example proof by mathematical induction for divisibility tests.

[emmansonessien]

Thanks alot. You made it all simple to understand❤❤.
I would also love you to make a video on finding all fourth root of 64i

[ExamSolutions_Maths]

Since it enables us to create the common factor (in this case 3) between the two terms when we add f(k) to both sides.

[DarkChild]

3:24 what is the logic behind that subtraction?

[IanSamirYepManzano]

If I have a problem whereby I end up with 12(12^k)+10(5^(k-1)) as my n=k+1 term and the n=k term is 12^k+2(5^(k-1)) and I am proving divisibility by 7, would it be legitimate to add f(k+1) twice since it would be faster to reach two terms that are divisible by 7 in this way rather than subtracting f(k+1) 5 times...Sorry for the wordy question...I hope it made some sense! :) thx in advance

[s.d]

why do you have to subtract f(k) from f(k+1) ?

[s.d]

oh ok thanks :) i would also love if you could make a factorial proof video too.. these videos help me so much. i really appreciate what you're doing for thousands of people

[flawns]

this made it more confusing .... fuuu. math in the summer suck

[DontReadThisAgain]

Thank you for explaining that. I love how the math is used