filmov
tv
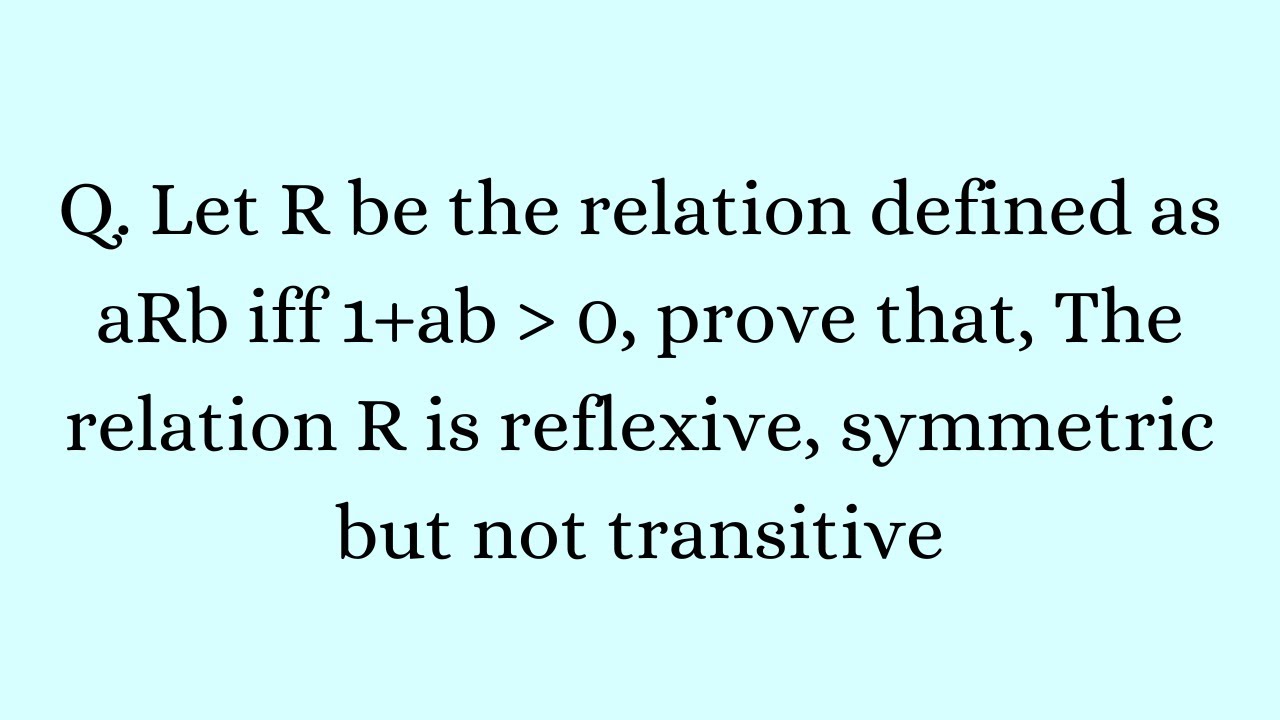
R is a relation: aRb iff 1+ab greater than 0, relation R is reflexive, symmetric but not transitive.
Показать описание
Let R be the relation defined as aRb iff 1+ab greater than 0, proving that the relation R is reflexive and symmetric but not transitive.
0:02:55
The relation aRb, If `|a| leqb, AA a,b in R` is
0:02:37
Given aRb iff ab is greater or equal to 0 explain why R is not an equivalence relation on set N
0:08:23
R is a relation: aRb iff 1+ab greater than 0, relation R is reflexive, symmetric but not transitive.
0:06:48
Given aRb a^3 =b^3 mod 5 prove R is an equivalence relation on the set N
0:04:40
Let us define a relation R in R as aRb if `a ge b`. Then, R is
0:06:54
Given aRb is a^2=b^2 mod 2 prove R is equivalence relation on set N. State the 2 equivalence classes
0:06:38
Let R be a relation defined on N as aRb if 2a+3b is a multiple of 5,a,b€N.Then R is
0:06:29
Equivalence Relation
0:05:01
What is an Equivalence Relation? | Reflexive, Symmetric, and Transitive Properties
0:00:57
ncert exemplar //relation R defined as aRb, if a is a brother of b, then R is//dhirendra sir/
0:05:06
Prove aRb iff a+b =2n is an equivalence relation on the set Z. State two equivalence classes
0:02:36
If R is a relation defined as aRb, `'iff'|a-b|gt0`, then the relation is
0:03:10
Let S be the set of R Then the relation R = {(a,b)/1+ab 0} on S is reflexive symmetric transitive
0:06:32
Check whether the Relation R in R defined by R={(a,b):a≤b³} is Reflexive, Symmetric or transitive|12...
0:08:53
Two Equivalence Classes [a] and [b] Are Equal If and Only If a is Related to b
0:03:20
Composition of relations | MISTAKE - explained RoS instead of SoR and vice versa | otherwise correct
0:15:36
RELATIONS - DISCRETE MATHEMATICS
0:05:18
Check whether the Relation R in the set {1,2,3,4,5,6} as R={(a,b):b=a+1} is Reflexive, Symmetric or
0:05:51
Representation of Relations
0:04:03
Relation R is reflexive and transitive but not symmetric, R defined as R = {(a, b) : a ≤ b}
0:08:06
The relation \( R \) defined in \( N \) as \( \mathrm{aRb} \Leftrightarrow \mathrm{b} \) is divi...
0:02:22
If relation R is defined as: aRb if ''a is the father of b''. Then, R is
0:06:36
Relation R in the set Z defined as R={(x, y):y=x-y is an integer} Reflexive, Symmetric, Transitive
0:03:45
If R be a relation for A = {1,2,3,4} to B = {1,3,5} such that (a,b) R iff a less than b then RoR-1
Комментарии