A awesome mathematics problem | Olympiad Question | can you solve this problem | x=?

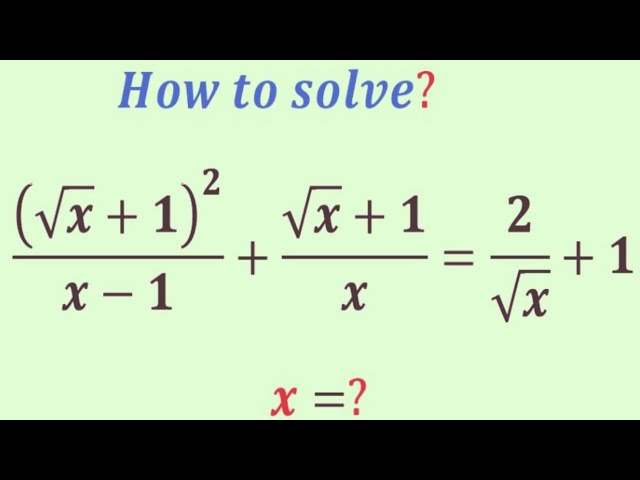
Показать описание

#quantativeaptitude #maths #olympiad #exponents #integral #awesome
france math olympiad question | can you solve this? | x=?, can you solve this math problem,can you solve this challenging problem?,can you solve this equation,can you solve this puzzle,learn how to solve this challenging problem,can you solve this maths puzzle,math olympiad algebra problem,how can solve this puzzle,math olympiad problem,olympiad mathematics,math olympiad problems,mathematics olympiad,mathematics

Рекомендации по теме

Комментарии

@tgx3529

But I tried to use substitution sqrtx +1= k
There Is the k^2=2
k= +- sqrt2
But k<0 Is not possible
For k=-sqrt2 Is x= 3+2sqrt2, Its not possible
Only 3-2sqrt2 Is possible

tgx

{2x^2/1x^2+2x^2/x^2}= 4x^4/1x^4 =4x^1 2^2x1^1 2^1^2x 1^1^2x 1^2x (x ➖ 2x+1). {2+2 ➖}/{x+x ➖ }{1+1 ➖}= {4/x^2+2 }= 6/x^2=3 (x ➖ 3x+3).

RealQinnMalloryu

_(√x + 1)²/(x - 1) + (√x + 1)/x = 2/√x + 1_
⇒ _(√x + 1)²/[(√x - 1)(√x + 1)] + (√x + 1)/x = 2/√x + 1_
⇒ _(√x + 1)/(√x - 1) + (√x + 1)/x - 2/√x - 1 = 0_
⇒ _((√x - 1)+ 2)/(√x - 1) + 1/√x + 1/x - 2/√x - 1 = 0_
⇒ _1 + 2/(√x - 1) + 1/√x + 1/x - 2/√x - 1 = 0_
⇒ _2/(√x - 1) - 1/√x + 1/x = 0_
Multiply through by _x(√x - 1)_ :
⇒ _2x - √x(√x - 1) + (√x - 1) = 0_
⇒ _x + 2√x - 1 = 0_
⇒ _(√x + 1)² - 2 = 0_
⇒ _√x + 1 = ±√2_
⇒ *_x = (±√2 - 1)² = 3 ∓ 2√2_*

guyhoghton

(√x + 1)²/(x - 1) + (√x + 1)/x = 2/√x + 1

find x ∈ ℝ
x > 0 ∧ x ≠ 1

x - 1 = (√x + 1)(√x - 1)

(√x + 1)/(√x - 1) + (√x + 1)/x = 2/√x + 1

(√x + 1)(1/(√x - 1) + 1/x) = (√x + 2)/√x

(√x + 1)(x + √x - 1)/[x(√x - 1)] = (√x + 2)/√x

(x + √x)(x + √x - 1) = x(√x - 1)(√x + 2)

(x + √x)(x + √x - 1) = x(x + √x - 2)

(x + √x)² - (x + √x) = x² + x√x - 2x
x² + x + 2x√x - x - √x = x² + x√x - 2x
x² + x√x = x² + x√x - 2x
x = 0 [ not valid ]

SidneiMV

A awesome mathematics problem | Olympiad Question | can you solve this problem | x=?