filmov
tv
Real Analysis Live - Problem Solving - Continuous Functions

Показать описание
Together with @fabiangabel
00:00 Intro
Real Analysis Live - Problem Solving - Continuous Functions
Real Analysis Live - Problem Solving ( check problem sheet here: https://tbsom.de/live )
Real Analysis Live - Problem Solving - Series and Convergent Criteria
Real Analysis Live - Problem Solving - Differentiable Functions
Real Analysis Live - Problem Solving - Series and Convergence Criteria (see tbsom.de/live)
Why greatest Mathematicians are not trying to prove Riemann Hypothesis? || #short #terencetao #maths
Real analysis practice: FIND A FIXED POINT
REAL ANALYSIS SOLVED PROBLEMS || mathematics solved problems // functions
Lec.(14) | Real Analysis | Diff., Imp. Ques. | IIT-JAM, NET | by IITian Parimal Sir (IIT Delhi)
6 Things I Wish I Knew Before Taking Real Analysis (Math Major)
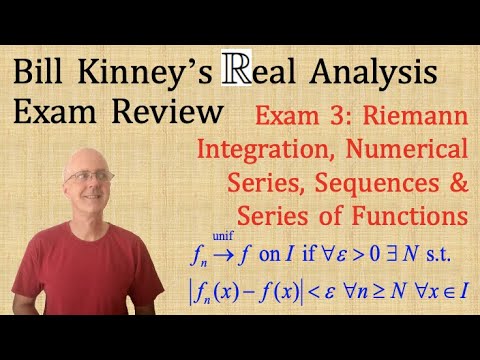
Real Analysis Exam 3 Review Problems and Solutions
Teaching myself an upper level pure math course (we almost died)
Learn Real Analysis With This Excellent Book
Daily Problems in Mathematics (1st May 2023) Real Analysis (English) (CSIR NET)
IQ TEST
BEST DEFENCE ACADEMY IN DEHRADUN | NDA FOUNDATION COURSE AFTER 10TH | NDA COACHING #shorts #nda #ssb
Why study real analysis?
Beauty of the Brain😍 IQ - IIT Bombay
Most💯 Important Step Before any Procedure 🔥
Jeff Bezos Quit Being A Physicist
11 years later ❤️ @shrads
Terence Tao Teaches Mathematical Thinking | Official Trailer | MasterClass
How to self study pure math - a step-by-step guide
1st yr. Vs Final yr. MBBS student 🔥🤯#shorts #neet
Комментарии
 2:13:26
2:13:26
 1:44:14
1:44:14
 1:29:49
1:29:49
 1:30:18
1:30:18
 1:30:03
1:30:03
 0:00:38
0:00:38
 0:23:29
0:23:29
 0:02:56
0:02:56
 1:44:31
1:44:31
 0:08:32
0:08:32
 1:35:00
1:35:00
 0:19:28
0:19:28
 0:10:40
0:10:40
 0:07:10
0:07:10
 0:00:29
0:00:29
 0:00:15
0:00:15
 0:04:30
0:04:30
 0:00:19
0:00:19
 0:00:16
0:00:16
 0:00:56
0:00:56
 0:00:11
0:00:11
 0:02:10
0:02:10
 0:09:53
0:09:53
 0:00:20
0:00:20