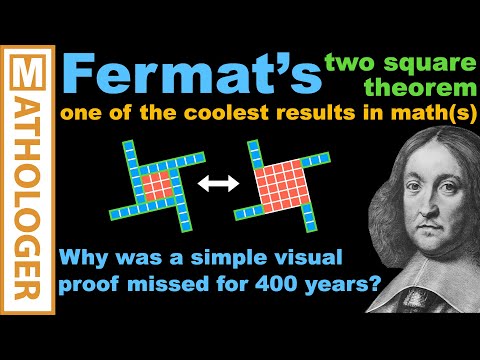
Detailed explanation of Zagier's one sentence proof

preview_player
Показать описание
A detailed explanation of Zagier's one-sentence proof

0:00 start
3:00 construct the involution from S to S and basic lemma
14:00 compute the fixed point
16:00 final prove that prime is 4k+1 is sum over two squares

Subscribe to my channel

Advanced mathematics list:

Real analysis:

Group representation for finite group

exact sequences

Ring theory lecture

group theory lecture

Category theory

Field theory in abstract algebra
  1. Math Geeks
  2. Detailed explanation of Zagier's one sentence proof
  3. Zagier's one sentence proof
  4. mathematics
  5. prime number
  6. prime numbers
Рекомендации по теме
Detailed explanation of

Detailed explanation of Zagier's one sentence proof

Is this the

Is this the most beautiful proof? (Fermat's Two Squares)

Don Zagier -

Don Zagier - Modular Forms and Differential Equations

Why was this

Why was this visual proof missed for 400 years? (Fermat's two square theorem)

Zagier's conjecture on

Zagier's conjecture on zeta(F,4) - Alexander Goncharov

8(b). Dedekind &

8(b). Dedekind & Zagier Reciprocity

Riemann Roch (Introduction)

Riemann Roch (Introduction)

The One Sentence

The One Sentence Proof (in multiple sentences) - Numberphile

What are the

What are the Betti numbers of a manifold? - Don Zagier - 2017

Ramanujan Yatra English

Ramanujan Yatra English Lecture Series - Prof Don Zagier

Marti Roset Julià,

Marti Roset Julià, The Gross--Kohnen--Zagier theorem via $p$-adic uniformization

A one sentence

A one sentence proof?! (Fermat's Two Squares)

Sergey Yurkevich -

Sergey Yurkevich - How to Conjecture and Prove that the Generating Function of the Yang-Zagier (...)

Ramanujan Yatra English

Ramanujan Yatra English Lecture Series - Prof Don Zagier

Don Zagier: Holomorphic

Don Zagier: Holomorphic quantum modular forms

Lennart Gehrmann, The

Lennart Gehrmann, The Gross-Kohnen-Zagier theorem via p-adic uniformization

Don Zagier -

Don Zagier - Mock Modularity and Applications

Alexander Goncharov Cluster:

Alexander Goncharov Cluster: polylogarithms and Zagier's conjecture

Wei Zhang -

Wei Zhang - 1/2 High-dimensional Gross–Zagier Formula

The Prime Problem

The Prime Problem with a One Sentence Proof - Numberphile

Modular forms: Introduction

Modular forms: Introduction

Shou-Wu Zhang. Neron–Tate

Shou-Wu Zhang. Neron–Tate heights and Gross‒Zagier formula

Zagier's Polylogarithm Conjecture

Zagier's Polylogarithm Conjecture revisited

Open Systems Theory

Open Systems Theory and BART

Комментарии
Автор

13:15
How do you know that |S| must be odd?
Given the condition you wrote down, it is possible that |A|=|C| and |B| is even, right?
I think the logic you present here has the wrong order.
We should do the following:
1. Study the fixed point condition for the set B as you did 15:15
2. Using the property the p is the prime and p=4k+1, there is only one solution of fixed point x=y=1 and z=k.
3. Conclude that |S| is odd and has exactly one fixed point.
4. Consider the other involution (x, y, z) -> (x, z, y), which must have some fixed point since we know that |S| is odd
5. This fixed point is equivalent to p = x^2 + (2y)^2, which shows that p is the sum of two integers.

YosiChen