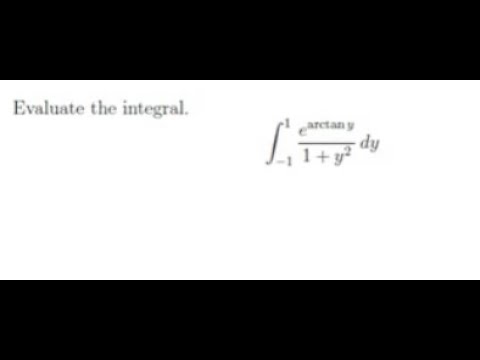
Evaluate the integral from -1 to 1 of [(e^(arctan(y))/(1 + (y^2))] dy

Transcript: Evaluate the definite integral from negative 1 to 1 of ((e to the arc tangent of y)/(1 + y squared)) with respect to y. Okay, looking at the integrand, I have a function arctangent of y, which is clearly inside function, and I have its derivative. So there's a clue. Let u be arctangent of y, and du = 1/(1 + y squared) dy, and the definite integral from negative 1 to 1 of ((e to the arctangent of y)/(1 + y squared)) dy is the definite integral, (e to the u). And then notice (1/(y squared)) dy is just du. Now what about the limits? Arctangent of 1, okay? What angle has tangent 1? That's pi/4 and arctangent of negative 1 = negative pi/4. So we have from pi/4 to negative pi/4. All right, well, the antiderivative of e to the u is e to the u, evaluated at the end points. So this is (e to the (pi/4)) - (e to the (negative pi/4)), and there is our definite integral.

Evaluate the integral. 1 4earctan(y) 1 + y2 dy −1

All Credits Go to Cengage