filmov
tv
Dividing polynomials using synthetic division
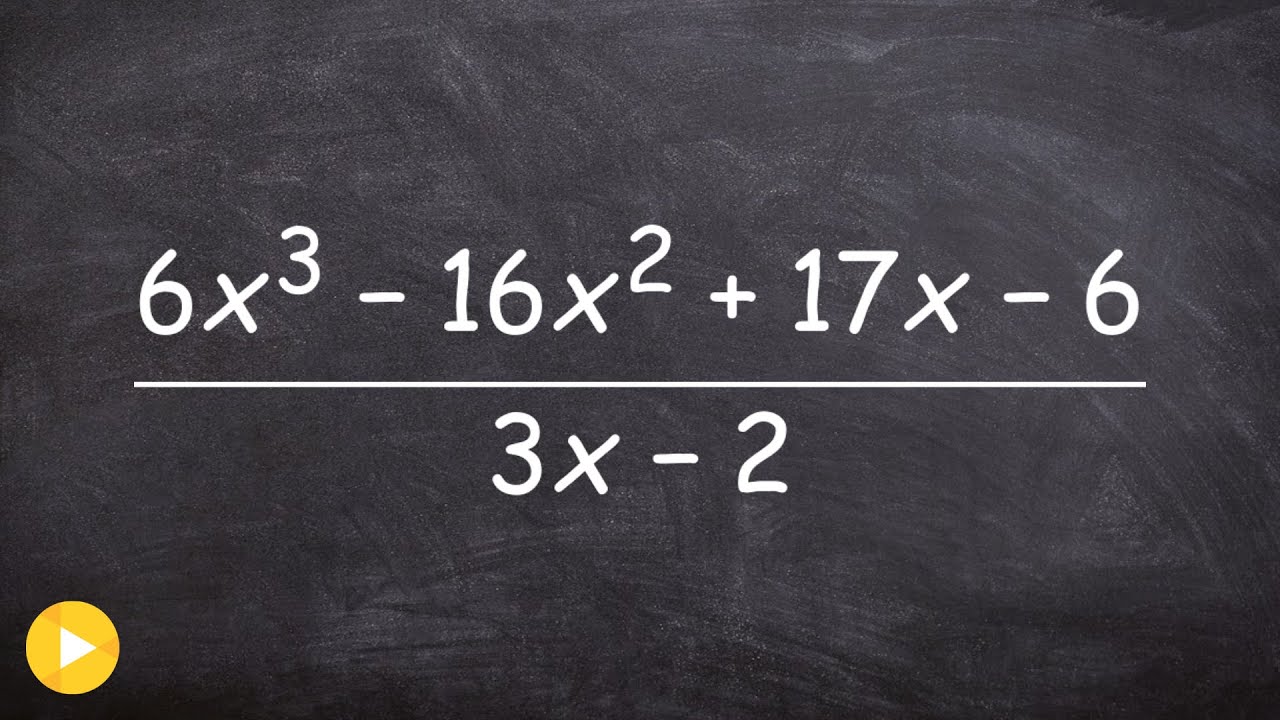
Показать описание
👉 Learn about dividing by synthetic division when the divisor is a fraction. Synthetic division is a method of dividing polynomials by linear expressions. To divide using synthetic division, we equate the divisor to 0 and then solve for the variable, the solution for the variable will be the synthetic divisor. In dividing with synthetic division, we set up the coefficients of the dividend polynomial against the synthetic divisor to get the coefficients of the quotient and the remainder. If there are any missing terms when applying synthetic division we must use a place holder zero in its place. It is also important to make sure your dividend polynomial is in standard form with descending powers.
Timestamps:
0:00 Intro
0:48 Start of Problem
Corrections:
3:06 Forgot to divide the solution with 3. Making the final quotient 2x^2 - 4x + 3.
Organized Videos:
✅Divide Polynomials using Synthetic Division
✅Divide Polynomials using Synthetic Division | Learn About
✅Divide Polynomials using Synthetic Division with missing terms
✅Divide Polynomials using Synthetic Division with fractions
✅Divide Polynomials using Synthetic Division with five terms
✅Divide Polynomials using Synthetic Division with three terms
✅Divide Polynomials using Synthetic Division with four terms
Connect with me:
#polynomials #brianmclogan
Timestamps:
0:00 Intro
0:48 Start of Problem
Corrections:
3:06 Forgot to divide the solution with 3. Making the final quotient 2x^2 - 4x + 3.
Organized Videos:
✅Divide Polynomials using Synthetic Division
✅Divide Polynomials using Synthetic Division | Learn About
✅Divide Polynomials using Synthetic Division with missing terms
✅Divide Polynomials using Synthetic Division with fractions
✅Divide Polynomials using Synthetic Division with five terms
✅Divide Polynomials using Synthetic Division with three terms
✅Divide Polynomials using Synthetic Division with four terms
Connect with me:
#polynomials #brianmclogan
Комментарии
 0:10:32
0:10:32
 0:04:33
0:04:33
 0:10:54
0:10:54
 0:06:13
0:06:13
 0:01:01
0:01:01
 0:11:55
0:11:55
 0:00:59
0:00:59
 0:03:00
0:03:00
 0:06:42
0:06:42
 0:10:07
0:10:07
 0:05:47
0:05:47
 0:05:21
0:05:21
 0:02:55
0:02:55
 0:04:52
0:04:52
 0:10:17
0:10:17
 0:00:59
0:00:59
 0:52:49
0:52:49
 0:02:35
0:02:35
 0:12:12
0:12:12
 0:03:08
0:03:08
![[TAGALOG] Grade 10](https://i.ytimg.com/vi/qclvLLhjOGQ/hqdefault.jpg) 0:20:37
0:20:37
 0:09:22
0:09:22
 0:08:57
0:08:57
 0:08:50
0:08:50