Addition Rule of Probability - Explained

The addition rule of probability can be used when you need to calculate the probability of A “or” B occurring:

P(A or B) = P(A) + P(B) - P(A and B)

VIDEO TRANSCRIPT
What’s the chance of drawing a heart or a face card? If some students play soccer, some play tennis, and some play both, what's the probability that a student selected plays at least one of the sports?

To answer these questions, we can use the addition rule of probability. It can be used when we’re interested in at least one of multiple criteria to be true, and also when we’re interested in the probability of A “or” B. Rolling a two OR a five. Drawing an 8 or a spade. Having an android phone Or an iphone.

We’ll start with two mutually exclusive events, which means they cannot both occur. For example, rolling a two OR a five. It’s not possible to roll a two and a five in a single roll. The addition rule of probability says that the probability of at least one of two mutually exclusive events occurring is simply the probability of the first event plus the probability of the second event. This is why it’s called the addition rule of probability. For rolling a two or a five, we would calculate the probability of rolling a two (1 out of 6) and the probability of rolling a five (1 out of 6) and add them together...2 out of 6. This works well as long as the events are mutually exclusive.

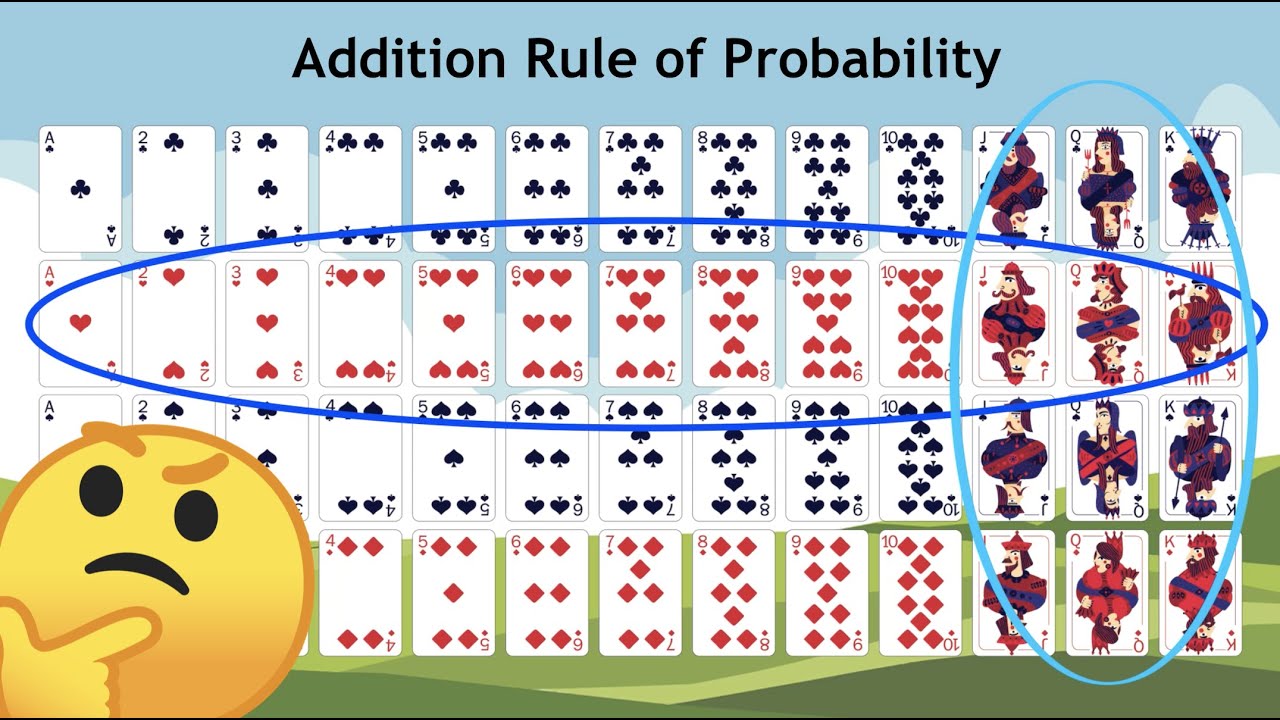
Let’s look at a second example. What’s the probability of drawing a heart or a face card? This is slightly more complicated because the events are not mutually exclusive—it’s possible to draw a queen of hearts which is both a heart and a face card. So we add one more element to our original formula. After adding the probability of event A and the probability of event B, we then must subtract the probability of event A and B to avoid double counting. If we count 13 hearts, and then 12 face cards, we’ve counted the jack, queen, and king of hearts twice. So we must subtract the number of cards that are both hearts and face cards in our formula.

You could also visualize it as a venn diagram of hearts and face cards. To calculate the total area, the easiest way is to add both circles together and then subtract the overlap.

As a final example, consider a group of students. 50% play soccer, 20% play tennis, and 10% play both soccer and tennis. What’s the probability that a student selected plays at least one of the sports? We can reword the question as what’s the probability that a student plays soccer OR tennis? This includes the students who play both. To answer this, we can use the formula. We add the probability of playing soccer to the probability of playing tennis and subtract the probability of playing both. The result is a 60% chance that a student selected at random plays at least one of the two sports.

In summary, if you want to know the likelihood of event A OR event B happening, or the probability that at least one of multiple events will occur, you can use the addition rule of probability.

Correction:
3:46 The formula should be P(A or B) = P(A) + P(B) - P(A and B).

[teodorminev]

Props for all the RUG IB studends here <3

[vilsa]

This video helped me so much, thank you! I’m doing online school and the way this program explains it is very confusing, but this video made it 100 times easier

[JimbobFaz]

The card explanation was brilliant, made perfect sense. Thanks!

[luisvelasquez]

Jeremy listen to me. I have been struggling soooo long to understand this subject; it was just not clicking for me, but it finally happened with your help.Your'e a damn legend

[shrustikulkarni]

THANK YOU A LOT SIR... IT HELPED ME IN DOING SEMINAR 🙏🙏

[jaggyjut]

what if there are more than two events. How can we apply the additional rule?

[Jaipur-random]

Any other topic on stats will do, cause these animations are very helpful

[amberbenton]

Thank you so much for yet again another wonderful tutorial about elem stats! This video really helped me connect what my professor was teaching in class.

[twobits]

Great Just lower the music, its distracting.
♥

[jimbomacgee]

At 3:46 shouldn't the formula be P(A or B) = P(A) + P(B) - P(A and B)?

[ZafarKhanatlantisimc]

Hi! Can you make a video on why golden ratio occurs more in nature as more probable than others. Also does golden ratio apply to stock markets rise and fall? Thanks in anticipation

[ferroumsamir]

Good explanation ! Nice. I understood your explanation rather than explanation on Linkedin

[johnsummers]

How about the probability of contracting a disease?

[MorolakeOBolarinwa]

This video is very helpful. Thank you so much.

[justStardust]

wonderful explanation. even better than crash course