filmov
tv
This Video will Trigger you and make you Incredibly Uncomfortable.
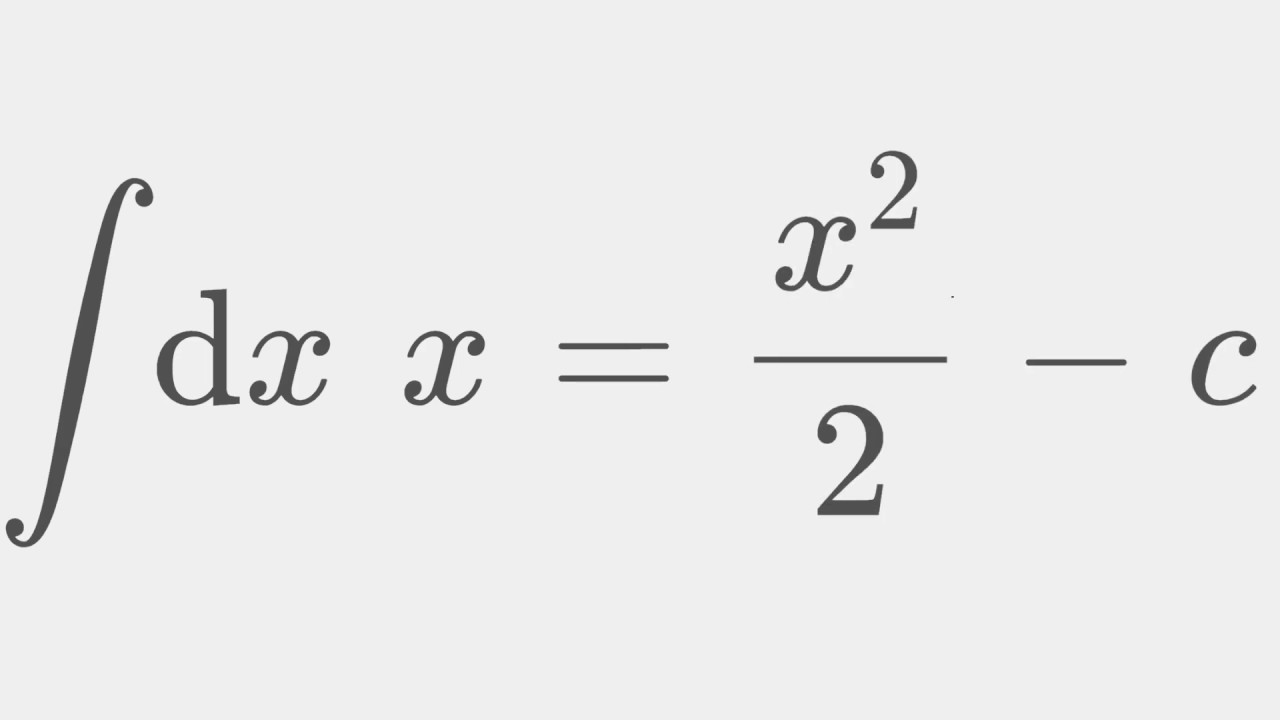
Показать описание
yes.
Help me create more free content! =)
--------------------------------------------------------------------------------
Wanna send me some stuff? lel:
Postfach 11 15
06731 Bitterfeld-Wolfen
Saxony-Anhalt
Germany
--------------------------------------------------------------------------------
Help me create more free content! =)
--------------------------------------------------------------------------------
Wanna send me some stuff? lel:
Postfach 11 15
06731 Bitterfeld-Wolfen
Saxony-Anhalt
Germany
--------------------------------------------------------------------------------
this cursed Blox Fruits video will trigger you..
this cursed Minecraft video will trigger you...
this minecraft video will tRIGGER you...
this cursed Minecraft house will trigger you...
The Most Unsatisfying Video Ever Made (OCD Trigger)
this cursed Minecraft lets play will trigger you...
this CURSED LEGO video will TRIGGER you
this cursed Minecraft video will make you cry...
Can you finish this video?
The Most Unsatisfying Video in the World ever made
This Minecraft Speedrun video will trigger you...
This Minecraft Video Will Annoy You
All your minecraft pain in one video...
this cursed Factorio video will trigger you...
This Video will Trigger you and make you Incredibly Uncomfortable.
this cursed minecraft video is painful to watch...
This minecraft video might trigger you
this will give you anxiety
this cursed minecraft video will trigger you...
This EU4 video will trigger you...
This Cursed Minecraft Video Will Trigger You | Dreams Cursed Minecraft World
This Cursed DST Video will Trigger you
Minecraft This Video will trigger you #shorts
This Game Will TRIGGER YOU..!
Комментарии
 0:15:53
0:15:53
 0:05:34
0:05:34
 0:00:59
0:00:59
 0:10:22
0:10:22
 0:05:55
0:05:55
 0:10:35
0:10:35
 0:08:10
0:08:10
 0:08:53
0:08:53
 0:07:45
0:07:45
 0:05:51
0:05:51
 0:02:50
0:02:50
 0:12:17
0:12:17
 0:01:25
0:01:25
 0:04:31
0:04:31
 0:01:42
0:01:42
 0:00:59
0:00:59
 0:04:16
0:04:16
 0:01:18
0:01:18
 0:11:03
0:11:03
 0:01:59
0:01:59
 0:04:54
0:04:54
 0:08:46
0:08:46
 0:00:26
0:00:26
 0:22:37
0:22:37