filmov
tv
Introduction to improper integrals | AP Calculus BC | Khan Academy

Показать описание
Improper integrals are definite integrals where one or both of the _bounderies is at infinity, or where the integrand has a vertical asymptote in the interval of integration. As crazy as it may sound, we can actually calculate some improper integrals using some clever methods that involve limits. Created by Sal Khan.
AP Calculus BC on Khan Academy: Learn AP Calculus BC - everything from AP Calculus AB plus a few extra goodies, such as Taylor series, to prepare you for the AP Test
For free. For everyone. Forever. #YouCanLearnAnything
Introduction to improper integrals | AP Calculus BC | Khan Academy
Improper Integrals - Convergence and Divergence - Calculus 2
Evaluating Improper Integrals
Calculus 2 Lecture 7.6: Improper Integrals
Improper Integrals Intro
Improper Integrals | Convergence & Divergence of Intigral | Maths 1 | Engineering Mathematics
Type 1 improper integrals! calculus 2
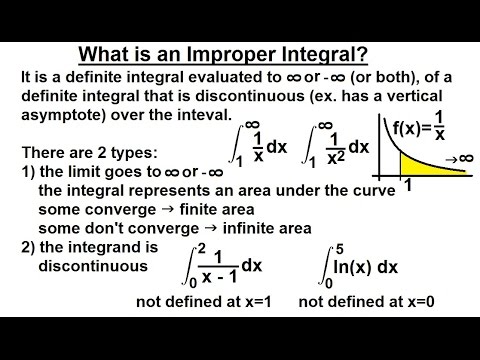
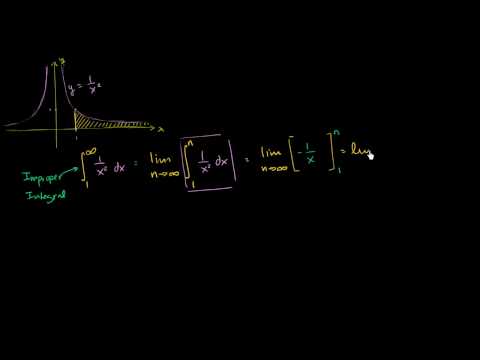
Calculus 2: Improper Integrals (1 of 16) What is an Improper Integral?
Introduction to Improper Integrals with Infinite Limits of Integration
Introduction to improper integrals
Simple & Straightforward Introduction to Improper Integrals (Type 1)
Introduction to improper integrals
Improper Integrals: Type 1: Infinite Intervals
Comparison Test for Improper Integrals
Introduction to Improper Integrals
1.Introduction to improper integral 1(Explanation : Hindi/ English)
Introduction to Improper Integrals
12.1 Improper integrals: Definition and Example 1
Improper Integrals: Introduction
Calculus II: Improper integrals
Calculus 2 -- Improper integrals -- Overview
Calculus 2: Improper Integrals (Video #7) | Math with Professor V
Improper Integrals: How to Integrate with Infinities, 2 ways!
Introduction to improper integrals
Комментарии
 0:03:52
0:03:52
 0:13:56
0:13:56
 0:12:24
0:12:24
 2:48:31
2:48:31
 0:05:47
0:05:47
 0:26:11
0:26:11
 0:27:48
0:27:48
 0:03:04
0:03:04
 0:07:22
0:07:22
 0:06:01
0:06:01
 0:14:48
0:14:48
 0:10:00
0:10:00
 0:13:25
0:13:25
 0:07:55
0:07:55
 0:28:56
0:28:56
 0:18:47
0:18:47
 0:10:38
0:10:38
 0:04:54
0:04:54
 0:05:04
0:05:04
 0:26:19
0:26:19
 0:18:54
0:18:54
 0:35:58
0:35:58
 0:08:51
0:08:51
 0:03:52
0:03:52