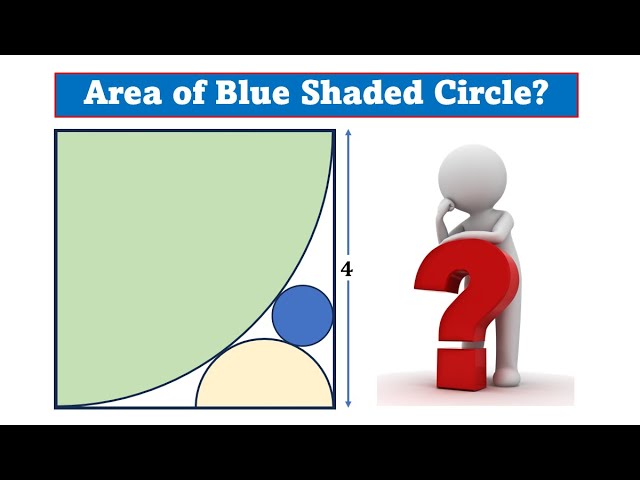
A hard Area of Circle Puzzle Lying Inside the Square and Outside a Quarter Circle and a Semicircle

#math #geometry #area #puzzles #problems
Welcome to our latest brain-teasing geometry puzzle! In this video, we present a perplexing challenge involving circles, semicircle, quarter circle and squares that will put your problem-solving skills to the test.

Imagine a square with a quarter circle inscribed on one side and a semicircle on another. The task? To determine the area of the circle confined within the square but lying outside both the quarter circle and the semicircle.

Join us as we dive into the intricacies of geometric shapes, exploring the relationships between circles, squares, and various segments. Through step-by-step analysis and clear explanations, we'll guide you towards unraveling the solution to this intriguing problem.

Whether you're a mathematics enthusiast, a puzzle aficionado, or simply looking for a mental workout, this video offers a stimulating challenge suitable for all levels of expertise. Get ready to stretch your mind and sharpen your geometric intuition!

Don't forget to hit the like button if you enjoy brain teasers like this one, and be sure to subscribe for more captivating puzzles and mathematical adventures. Let's embark on this geometric journey together!

A hard Area of Circle Puzzle Lying Inside the Square and Outside a Quarter Circle and a Semicircle
Geometry
Square,
Quarter Circle,
Semi-circle,
Semicircle,
Square Circle,
Area,
Construct Line Segment,
Diameter,
Radius,
Perpendicular,
Triangle,
Right Triangle,
Center of a Circle,
Pythagorean Theorem, #Geometry, Semicircle,
#QuarterCircle,
hypotenuse,
semi circle,
mathematics,
circle,
maths tricks,
semi circle and quarter circle,
mathematics semi circle,
semi circle animation,
learn shapes,
learn quarter circle,
how to learn math,
learn mathematics,
how to learn mathematics,
learn math

[gelbkehlchen]

Solution:
b = radius of the blue circle,
g = radius of the green quarter circle = 4,
y = radius of the yellow semicircle,
a = side of the square = 4,
d = distance of the center of the blue circle from the bottom of the square.

Pythagoras:
Hypotenuse: g+y
Vertical leg: a
Horizontal leg: a-y
(g+y)² = a²+(a-y)² ⟹
(4+y)² = 4²+(4-y)² ⟹
16+8y+y² = 16+16-8y+y² |-16-y²+8y ⟹
16y = 16 |/16 ⟹
y = 1

Pythagoras:
Hypotenuse: b+y
Vertical leg: d
Horizontal leg: y-b
(1) (b+y)² = d²+(y-b)² ⟹
(1a) (b+1)² = d²+(1-b)² ⟹
(1b) b²+2b+1 = d²+1-2b+b² |-b²-1+2b ⟹
(1c) 4b = d²

Pythagoras:
Hypotenuse: b+g
Vertical leg: a-d
Horizontal leg: a-b
(2) (b+g)² = (a-d)²+(a-b)² ⟹
(2a) (b+4)² = (4-d)²+(4-b)² ⟹
(2b) b²+8b+16 = 16-8d+d²+16-8b+b² |-b²-16+8b ⟹
(2c) 16b = 16-8d+d² |(2c)-(1c) = (3) 12b = 16-8d |+8d-12b ⟹
(3a) 8d = 16-12b |/8 ⟹
(3b) d = 2-3/2*b |into (1c) ⟹
(1d) 4b = (2-3/2*b)² = 4-6*b+9/4*b² |-4b ⟹
(1e) 9/4*b²-10*b+4 = 0 |*4/9 ⟹
(1f) b²-40/9*b+16/9 = 0 |p-q-formula ⟹
(1g) b1/2 = 20/9±√(400/81-16/9) = 20/9±√(400/81-144/81) = 20/9±√(256/81)
= 20/9±16/9 ⟹
(1h) b1 = 20/9+16/9 = 4 [too big as a solution in a square with the side of 4] and b2 = 20/9-16/9 = 4/9 that is the solution ⟹
Area of the blue circle = π*b² = 16/81*π

[rey-dqnx]

(4+r)²=(4-r)²+16
16+8r+ r²=16-8r+r²
16r=16
r=1
(1+x)²=(1-x)²+y²
1+2x+x²-(1-2x+x²)=y²
1+2x+x²-1+2x-x²=y²
y²=4x
y=2√x
(4+x)²-(4-x)².= z²
16+8x+x²-16+8x-x²=z²
16x=z²
z= 4√x
y+z=4
2√x+4√x=4
6√x=4
√x=2/3
x=4/9

Area=16π/81