Well Behaved Preferences of IC (Monotonicity, convexity and averages being preferred to extremes)

The assumption of monotonicity of preferences-
If (x1,x2) is a bundle of goods and (y1,y2) is a bundle of goods with at least as much of both goods and more of one, then (y1,y2) is preferred to (x1,x2).
This monotonicity assumption is the reason why IC’s have a negative slope.

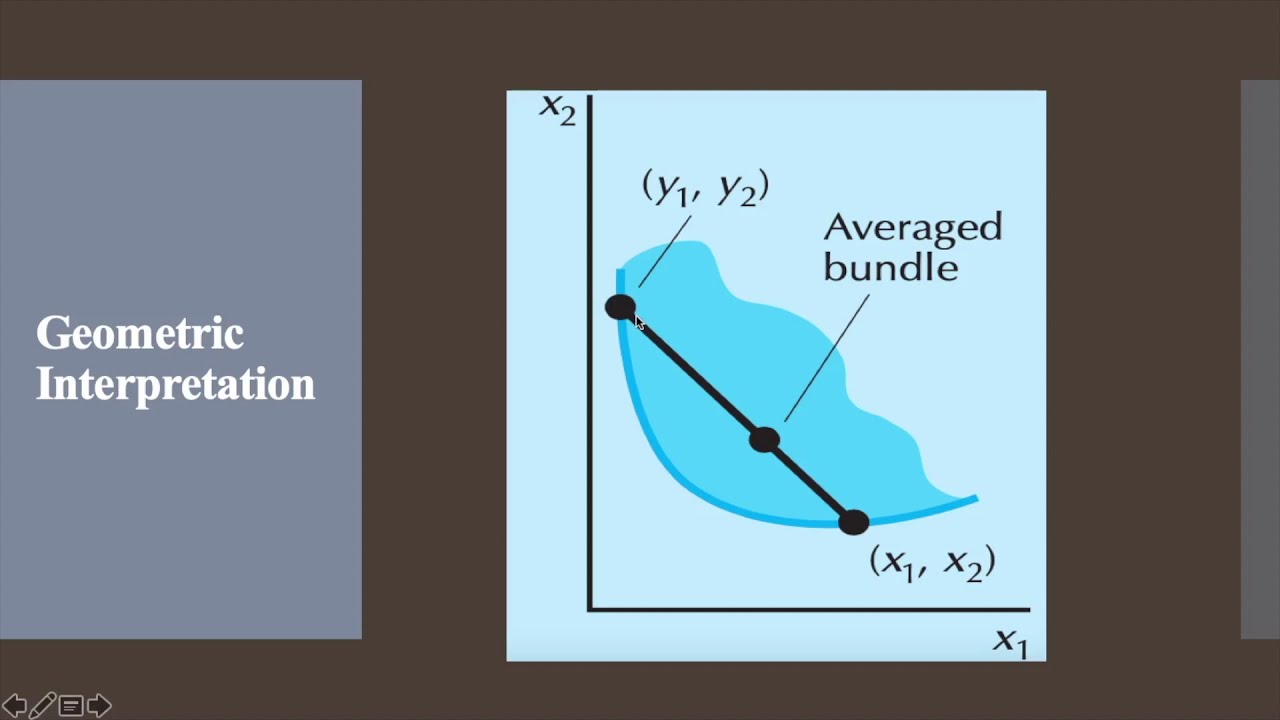
Secondly, it is assumed that the averages are preferred to extremes. That is, if we take two bundles of goods (x1,x2) and (y1,y2) on the same indifference curve and take a weighted average of the two bundles such as ½ x1 + ½ y1, ½ x2 +1/2y2 , then the average bundle will be at least as good as or is strictly preferred to each of the two extreme bundles.

A convex set has the property that if you take any two points in the set and draw the line segment connecting those two points, that line segment lies entirely in the set. Hence, usually the averages are preferred to the extremes.

[cushyacademy]

Its just a reading from the book of varian make it an explanation