filmov
tv
Algebraic Graph Theory: Extensions of EKR to 2-intersecting perfect matchings and permutations

Показать описание
Talk by Andriaherimanana Sarobidy Razafimahatratra & Mahsa Nasrollahi Shirazi.
The Erdős-Ko-Rado (EKR) theorem is a classical result in extremal combinatorics. It states that if n and k are such that $n$ is at least 2, then any intersecting family F of k-subsets of [n]={1,2,...,n} has size at most n-1 choose k-1. Moreover, if n is strictly greater than 2k, then equality holds if and only if F is a canonical intersecting family; that is, the intersection over all A in F is {i}, for some i in [n].
The EKR theorem can be extended to various combinatorial objects. In this presentation, we will talk about some variations of the EKR theorem for 2-intersecting perfect matchings and 2-intersecting permutations. In particular, we will first prove that any 2-intersecting family of perfect matchings of K_{2k} has size at most (2k-5)(2k-7)...(3)(1), for any k at least 3. Then, we will show that any 2-pointwise (resp. 2-setwise) intersecting family of Sym(n) has size at most (n-2)! (resp. 2(n-2)!) for n at least 5.
The Erdős-Ko-Rado (EKR) theorem is a classical result in extremal combinatorics. It states that if n and k are such that $n$ is at least 2, then any intersecting family F of k-subsets of [n]={1,2,...,n} has size at most n-1 choose k-1. Moreover, if n is strictly greater than 2k, then equality holds if and only if F is a canonical intersecting family; that is, the intersection over all A in F is {i}, for some i in [n].
The EKR theorem can be extended to various combinatorial objects. In this presentation, we will talk about some variations of the EKR theorem for 2-intersecting perfect matchings and 2-intersecting permutations. In particular, we will first prove that any 2-intersecting family of perfect matchings of K_{2k} has size at most (2k-5)(2k-7)...(3)(1), for any k at least 3. Then, we will show that any 2-pointwise (resp. 2-setwise) intersecting family of Sym(n) has size at most (n-2)! (resp. 2(n-2)!) for n at least 5.
Algebraic Graph Theory: Extensions of EKR to 2-intersecting perfect matchings and permutations
Algebraic Graph Theory: The Matching Polynomial
Algebraic Graph Theory: Various Maximum Nullities Associated with a Graph
Algebraic Graph Theory: Uniform mixing on oriented graphs
Paul Terwilliger: The alternating central extension of the $q$-Onsager algebra
Algebraic Graph Theory: Group Theory and the Erdős-Ko-Rado (EKR) Theorem
Daniel Spielman “Miracles of Algebraic Graph Theory”
Algebraic Graph Theory: State transfer and the size of the graph
Algebraic Graph Theory: Pretty Good State Transfer and Minimal Polynomials
Algebraic Graph Theory: Complexity Measures on the Symmetric Group and Beyond
Algebraic Graph Theory: Tight 2-designs in complex projective spaces
Algebraic Graph Theory: Leonard pairs, spin models, and distance-regular graphs
Algebraic Graph Theory: Feynman integrals as algebraic graph theory
Algebraic Graph Theory: Minimum eigenvalue of nonbipartite graphs
Algebraic Graph Theory: Laplacian Quantum Fractional Revival On Graphs
Lecture 13 | Algebraic Graph Theory and Quantum Computing
Lecture 14 | Algebraic Graph Theory and Quantum Computing
Lecture 24 | Algebraic Graph Theory and Quantum Computing
Algebraic Graph Theory: A covering graph perspective on Huang’s theorem
Algebraic Graph Theory: Quantum walks on Cayley graphs
Algebraic Graph Theory: Equiangular lines over finite fields
Lecture 29 | Algebraic Graph Theory and Quantum Computing
Algebraic Graph Theory: Continuous Quantum Walks on Graphs
Memorization Trick for Graphing Functions Part 1 | Algebra Math Hack #shorts #math #school
Комментарии
 0:59:43
0:59:43
 0:56:06
0:56:06
 0:51:23
0:51:23
 0:54:39
0:54:39
 1:13:01
1:13:01
 0:57:02
0:57:02
 0:52:41
0:52:41
 0:59:45
0:59:45
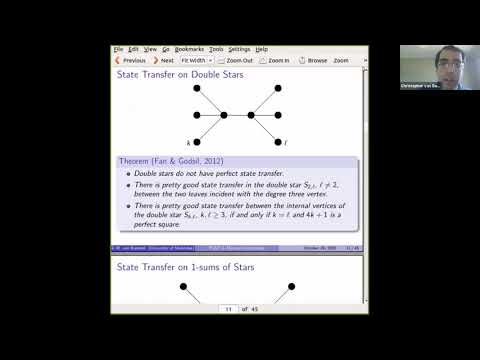 0:47:24
0:47:24
 1:12:06
1:12:06
 0:59:39
0:59:39
 1:03:17
1:03:17
 0:50:34
0:50:34
 0:50:22
0:50:22
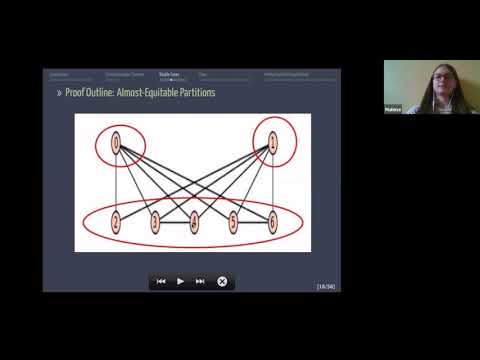 0:51:02
0:51:02
 0:49:29
0:49:29
 0:44:47
0:44:47
 0:49:45
0:49:45
 0:46:20
0:46:20
 0:45:30
0:45:30
 1:03:47
1:03:47
 0:47:30
0:47:30
 0:51:37
0:51:37
 0:00:15
0:00:15