Calculus 2: Parametric Equations (19 of 20) Find the Length of an Arch of a Cycloid

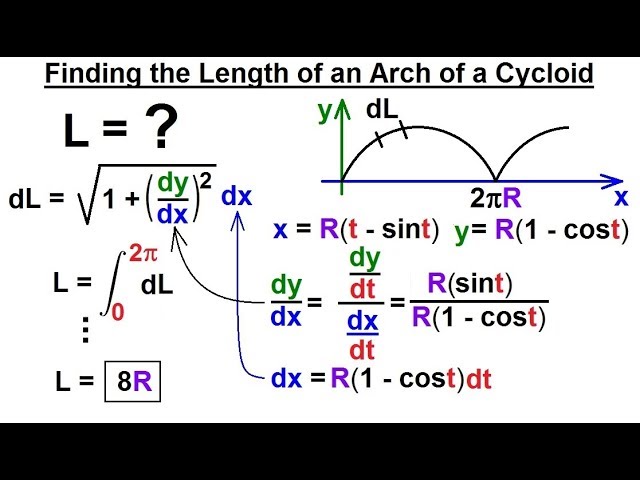
In this video I will find the length, L=? (dL=[1+(dy/dx)^2]^1/2dx), under a single arc of a cycloid using the equations y=R(1-cost) and x=R(t-sint).

Next video in the series can be seen at:

[acasaresm]

And what about the length of an Hipocycloid given its parametric equations x =
y =

[joeyborja]

1 video to finish for this series but at this time, I just would like to thank you for teaching us how to manipulate identities to get out of the messy line 4 inside the radical sign - that's one heluva skill that only a few could master. And oh those two (or three?) action figures to the bottom right kill it! Kudos!

[yumi-bvgf]

Sir if the question ask let s= arc length of the cycloid in the period of 0《t《B (B《2pi), how to show that s= 8 a sin²(B/4)

[heffrondrive]

Puede que quizá no lea esto, pero muchísimas gracias ❤️

[arashdeeppanesar]

Sir, how come the domain of integration is 0 => 2pi instead of 0 => 2piR ?