filmov
tv
Sampling Signals (3/13) - Fourier Transform of an Impulse Sampled Signal
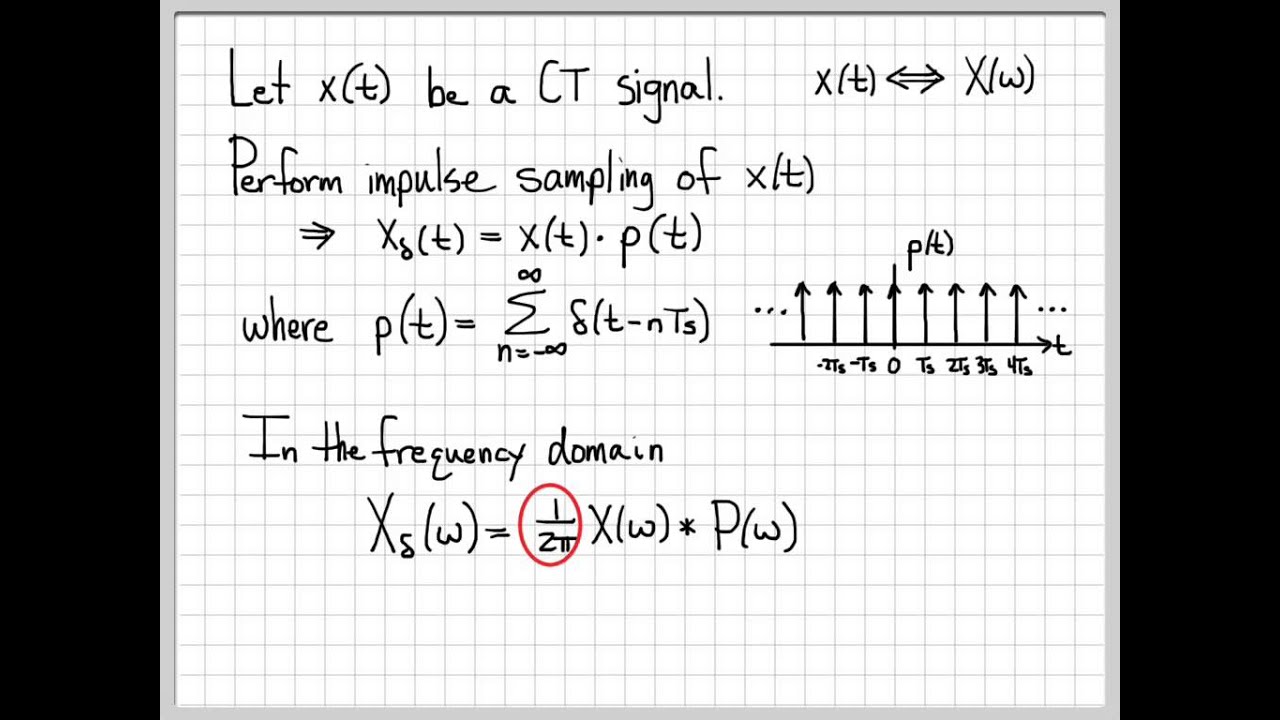
Показать описание
We investigate impulse sampling in the frequency domain, i.e. we derive an expression for the Fourier Transform (FT) of a signal that has been impulse sampled. If x(t) is the continuous-time signal with corresponding FT X(w), the impulse sampled version of x(t) has a FT that consists of an infinite collection of X(w) shifted up and down the frequency axis. Each shifted version of X(w) occurs at an integer multiple of the sampling frequency ws.
Sampling Signals (3/13) - Fourier Transform of an Impulse Sampled Signal
Example: Effect of Sampling on the Fourier Transform of a Bandlimited Signal
Sampling Example
Frequency Sampling and the Discrete Fourier Transform
Frequency Sampling and the Discrete Fourier Transform (Part 2)
Sampling Signals (12/13) - Practical Reconstruction
Sampled signals: Discrete Time Fourier Transform (#003)
Shannon Nyquist Sampling Theorem
Tomography - Sampling Theorems and Fourier Series
ME 360 04 02 fourier series
Lecture 13: Fourier examples, indexing/plotting, sampling
Fourier Transform of Basic Signals (Sampling Function)
Computing the Spectrum of Sampled Signals with the Discrete Fourier Transform
fourier transform of sampled signal
Sampling Signals (4/13) - Impulse Sampling
Nyquist Sampling Problem 02
2-Dimensional Sampling Theory
Sampled signals: exploring the Fourier transform (#006/000)
ENEL327 - Sampling - Discrete-Time Fourier Series
mod11lec74-Fourier Transform of Sampled Signals
036. Fourier Transform: Sampling
Sampled signals: The effect of finite length on the Fourier Transform
The Spectrum of Sampled Signals
Typical Exam Question on Sampling
Комментарии
 0:09:58
0:09:58
 0:05:53
0:05:53
 0:08:41
0:08:41
 0:21:33
0:21:33
 0:15:20
0:15:20
 0:04:14
0:04:14
 0:02:55
0:02:55
 0:17:19
0:17:19
 1:08:24
1:08:24
 0:11:04
0:11:04
 1:46:08
1:46:08
 0:06:02
0:06:02
 0:13:15
0:13:15
 0:03:09
0:03:09
 0:07:28
0:07:28
 0:01:33
0:01:33
 0:10:13
0:10:13
 0:04:30
0:04:30
 0:09:44
0:09:44
 0:27:30
0:27:30
 0:58:04
0:58:04
 0:19:08
0:19:08
 0:13:20
0:13:20
 0:09:49
0:09:49