filmov
tv
The Spectral Theorem
Показать описание
The Complex Spectral Theorem and the Real Spectral Theorem, with examples.
The Spectral Theorem
Oxford Linear Algebra: Spectral Theorem Proof
Spectral Theorem For Dummies - 3Blue1Brown Summer of Math Exposition #SoME1
Example of Spectral Theorem (3x3 Symmetric Matrix)
Linear Algebra - Spectral Decomposition
Visualize Spectral Decomposition | SEE Matrix, Chapter 2
What is...the spectral theorem?
Lecture - 12.4 Spectral Theorem
Math of QM: 5.4. Motivation of Spectral Theorem via the Finite-Dimensional Case
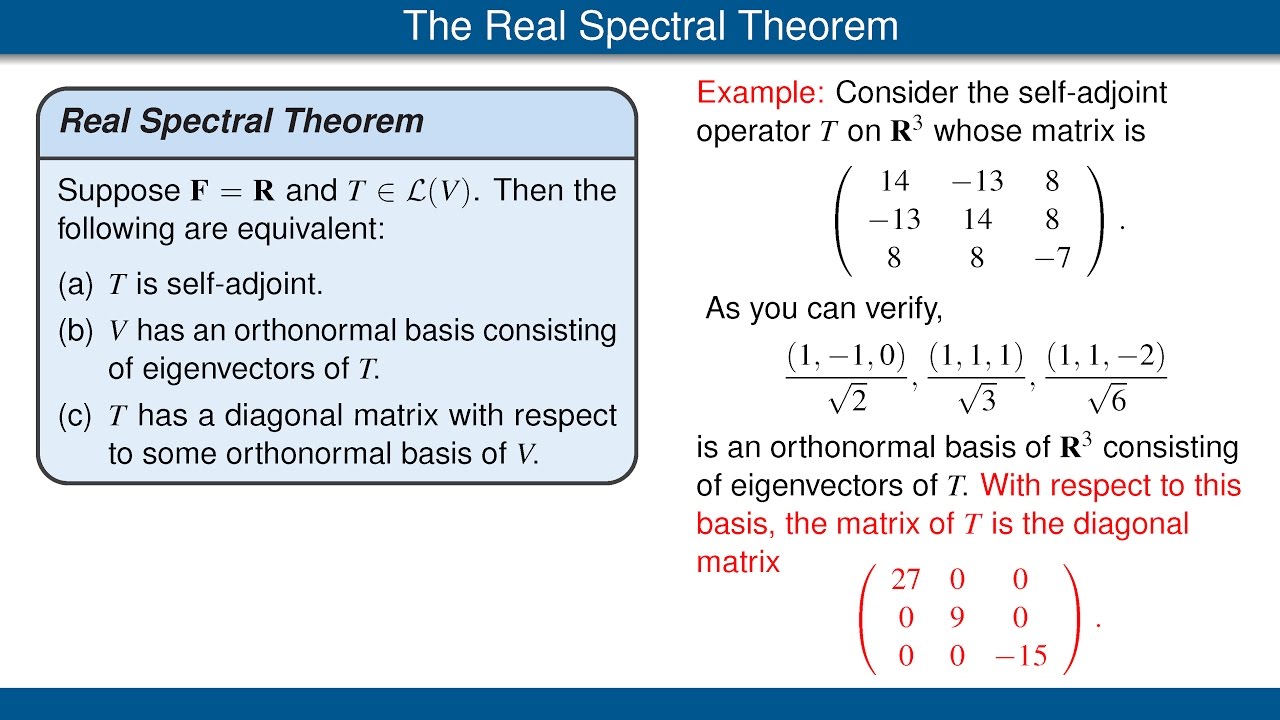
Real Spectral Theorem
Spectral Theorem for Compact Operators, and Coming back to my office after 2 years.
Symmetric Matrix
Functional Analysis 34 | Spectral Theorem for Compact Operators [dark version]
The Spectral Theorem #shorts
Lecture 22: The Spectral Theorem for a Compact Self-Adjoint Operator
Functional Analysis 28 | Spectrum of Bounded Operators
Shifrin Math 3510 Day53: Spectral Theorem
Positive Semi-Definite Matrix 2: Spectral Theorem
Spectral Theorem - L11 - Frederic Schuller
Understanding the Spectral Theorem: A Simple Guide
The “Spectral Theorem”
Advanced Linear Algebra, Lecture 4.5: The spectral theorem
Spectral Theorem for Real Matrices: General nxn Case
22b: Why the Spectral Theorem works
Комментарии
 0:11:18
0:11:18
 0:35:00
0:35:00
 0:07:06
0:07:06
 0:07:54
0:07:54
 0:10:54
0:10:54
 0:15:55
0:15:55
 0:11:49
0:11:49
 0:41:20
0:41:20
 0:15:47
0:15:47
 0:26:27
0:26:27
 0:21:58
0:21:58
 0:25:51
0:25:51
 0:09:21
0:09:21
 0:00:59
0:00:59
 1:24:07
1:24:07
 0:07:45
0:07:45
 0:46:51
0:46:51
 0:12:39
0:12:39
 1:59:41
1:59:41
 0:03:19
0:03:19
 0:06:16
0:06:16
 0:32:59
0:32:59
 0:15:34
0:15:34
 0:16:49
0:16:49