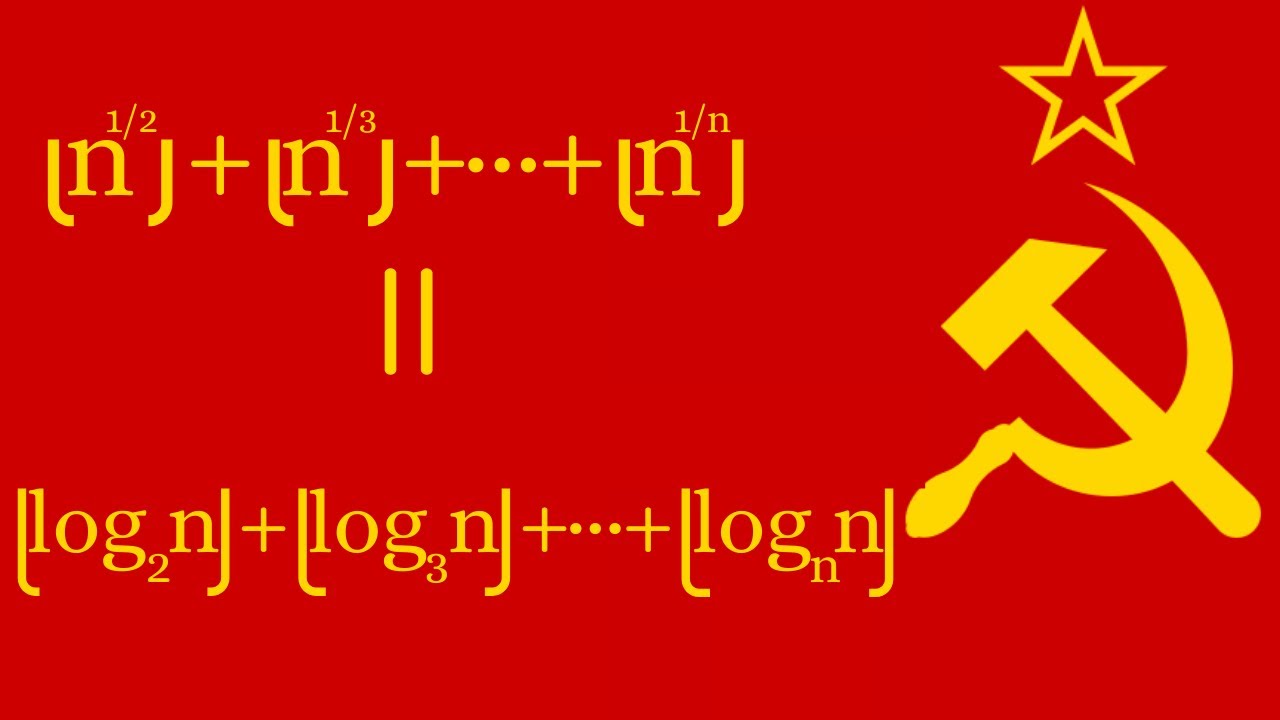
A classic problem from the 1982 Soviet Mathematical Olympiad

We present a solution to a classic problem with first(?) appeared on the 1982 Soviet Mathematical Olympiad.

[michel_dutch]

In Soviet Russia, expression takes logarithm of you

[arpitdas]

This is the first ever Olympiad problem I've seen that requires such a rigorous use of Calculus. Great stuff

[RobsMiscellania]

One of my most loved books is a now decades-old copy of The USSR Olympiad Problem Book: Selected Problems and Theorems of Elementary Mathematics. I have come to peace with the fact that I may never solve all of the book's 320 very difficult but rewarding problems. To me, it was an enigma, all problems my 15 year old mind could comprehend but could scarcely approach only the lowest hanging fruit. Decades later, it is clear to me that this book is part of those responsible for setting alight my passion for exploratory mathematics, and doing math for its own sake, as a means of exploring the creative and analytical methods behind the concepts.

[mstarsup]

there's a "-1" missing in the integral for the computation of the area A with the first version of the computation. The area should stop at 1 on the vertical axis, not at 0. It doesn't change much about the intuition part though.

[bhoenix]

This is interesting because it seems to also prove the same thing for:


floor(f(1)) + floor(f(2)) + ... + floor(f(n)) = floor(f^-1(1)) + floor(f^-1(2)) + ... + floor(f^-1(n))


Where f is an invertible positive function (where 1 <= x <= n) such that n <= f(0), and n <= f^-1(0) (or the limit coming from the positive side if not possible)


I probably made a mistake lmao, I haven't tested this with any functions that satisfy this.


EDIT: Changed continuous to invertible

[lt]

another great solution is to consider A = { x, y integers such that, x>1, y >0 and x^y< or = n} and count it in two ways

[yueyangzhang]

Thank you for putting such great education material on board. I am thrilled to watch.

[willyh.r.]

As a math teacher, I'm very impressed with your ability to solve strange math problems. Can you share with us your method? Thank you.

[dominikstepien]

I see no point in doing these integrals, just counting the points does the job. Very hard problem and interesting solution nevertheless.

[EquuleusPictor]

I'm very surprised by this equation, as it's known that the log increases much slower than the root function, yet when floored and averaged from 2 to n it yields the same result ! That's fantastic really !

[senco]

Such an interesting solution, thank you for this!

[mehdisi]

Excellent intuitive proof. Thank you so much.

[taopaille-paille]

Man thank you for all these videos. You have amazing presentation skills and great maths skills. Your channel is an extremely useful source for doing great maths

[peterdecupis]

Cool example! And we can easy build a generalized theorem on this method in order to state the equality between the discrete summation of lowerint[f(k)] and the discrete summation of lowerint[inversef[(h)], when f (and its inverse) is a monotonic continuous function, provided that the summation ranges are suitably matched

[mili]

Dude your channel is incredible, please don’t stop making videos.

[disguisedhell]

There seems something incorrect in the integral 1 to n of (n^1/x)dx. It also calculates the additional area of n-1( the rectangular box) that you missed shading in area

[darkseid]

You chose your shirt very cleverly . 🤣👌

[pawebielinski]

What a great problem. I like it a lot, thank you for your work!

[PaulHobbs]

Very intuitive solution! With my first look at the problem, it seemed like the problem statement was wrong, because n^1/2 grows so much faster than log_2(n), so to have a very simple and obvious solution to the problem was nice to see.

[MrAhYo]

Great approach to solving this problem. This video has inspired me to ask you for more combinatorics problems 😀