filmov
tv
Find the radius of the circle | Geometry Question | Important Geometry And Algebra Skills Explained

Показать описание
Find the radius of the circle | Geometry Question | Important Geometry And Algebra Skills Explained
Join this channel to get access to perks:
MEMBERS OF THIS CHANNEL
••••••••••••••••••••••••••••••••••••••••
Mr. Gnome
Sambasivam Sathyamoorthy
ஆத்தங்கரை சண்முகம்
Εκπαιδευτήρια Καντά
AR Knowledge
堤修一
Sidnei Medeiros Vicente
Mark Ludington
Saunak Swar
Grace Cooper
Nicholas Garcia
Ben Stone
Karlo Karbs
Join this channel to get access to perks:
MEMBERS OF THIS CHANNEL
••••••••••••••••••••••••••••••••••••••••
Mr. Gnome
Sambasivam Sathyamoorthy
ஆத்தங்கரை சண்முகம்
Εκπαιδευτήρια Καντά
AR Knowledge
堤修一
Sidnei Medeiros Vicente
Mark Ludington
Saunak Swar
Grace Cooper
Nicholas Garcia
Ben Stone
Karlo Karbs
Find the Radius
Finding the Radius of a Circle Given the Diameter | Math with Mr. J
How to Find Radius of Circle from Area | Geometry
Finding the radius when given the circumference
Finding the radius of a circle
Can you find the Radius of the circle? | (Triangle inscribed in a circle) | #math #maths
How to calculate the RADIUS, DIAMETER and the CIRCUMFERENCE of a circle
How To Find The Center and Radius of a Circle
Finding the radius by using A=pi.r^2 #solvingmaths #mathdad #MathsWithYohannes
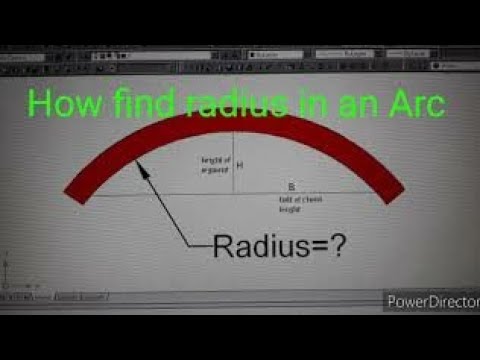
HOW TO FIND A RADIUS IN A CIRCLE / ARC
Find the radius of the circle whose diameter is 40 cm
What is the Radius of a Circle? | What is the Diameter of a Circle? | Radius and Diameter Explained
How to Find the Radius of a Circle (Circumference Given)
Is this even solvable? What is the radius?
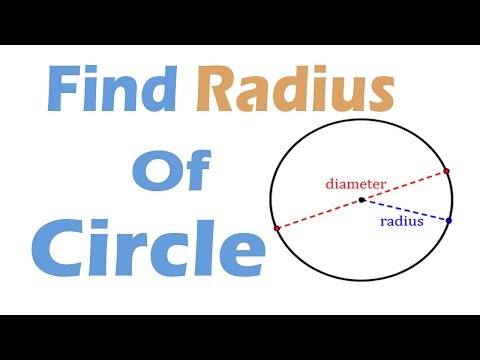
How to Find The Radius Of Circle
How to calculate an arch radius!
How to find the center and radius of a circle in standard form
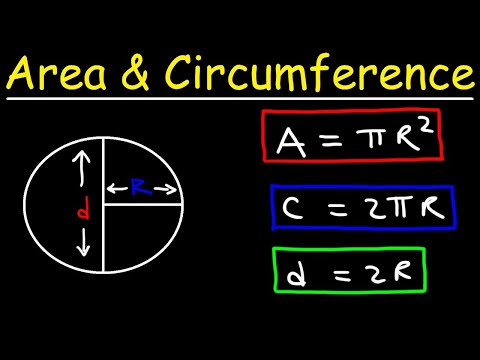
Circles - Area, Circumference, Radius & Diameter Explained!
Find Radius of a Cylinder from Volume and Height | Geometry
Finding the radius of a sphere given the volume
Find the Radius and Centre of Circle
Can You Find the Radius of the Circle? | Quick & Simple Tutorial
Finding the Diameter of a Circle Given the Radius | Math with Mr. J
TIP: Easy Arc Radius (Intersecting Chords Theorem)
Комментарии
 0:03:25
0:03:25
 0:02:56
0:02:56
 0:05:03
0:05:03
 0:01:51
0:01:51
 0:01:40
0:01:40
 0:10:25
0:10:25
 0:08:14
0:08:14
 0:06:37
0:06:37
 0:01:01
0:01:01
 0:02:08
0:02:08
 0:01:33
0:01:33
 0:03:52
0:03:52
 0:01:02
0:01:02
 0:12:21
0:12:21
 0:02:05
0:02:05
 0:01:01
0:01:01
 0:05:06
0:05:06
 0:17:20
0:17:20
 0:07:55
0:07:55
 0:03:28
0:03:28
 0:05:20
0:05:20
 0:09:15
0:09:15
 0:03:08
0:03:08
 0:05:03
0:05:03