Find Radius of a Cylinder from Volume and Height | Geometry

How do we find the radius of a cylinder given its volume and height? We'll go over that in today's video geometry lesson with examples and explanations!

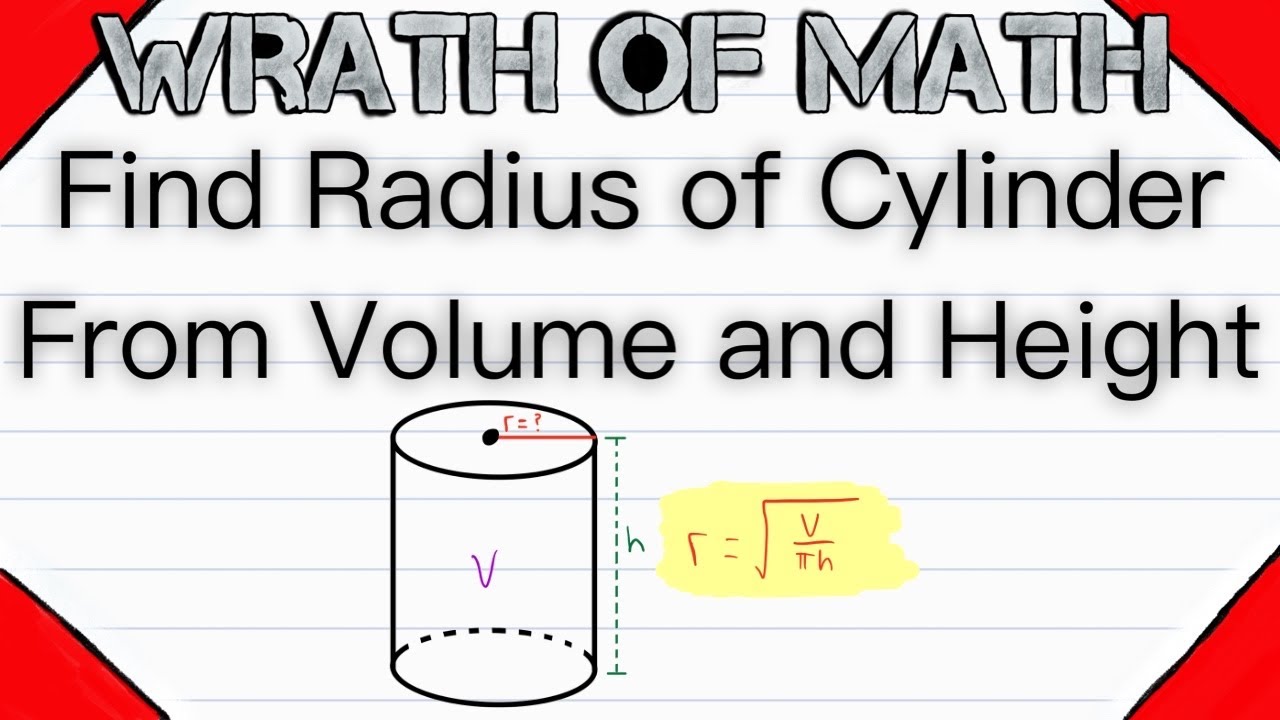
Let V be the volume of the cylinder, r the radius of the base, and h the height. Then, recall that the volume is equal to pi*r^2*h. That is: V = pi*(r^2)*h. We can solve this equation for r in order to calculate radius when given volume and height. We'll go over that process in the video, but what we end up with is r = sqrt(V/(pi*h)). We can then double the radius to find the diameter of the cylinder!

SOLUTION TO PRACTICE PROBLEM:

We are given a volume of 12 m^3 and a height of 5 m. Plugging these into the equation for volume ( V = pi*(r^2)*h ), we have that 12 = pi*(r^2)*5. Notice we are not including the units because we know the final number will be in our base unit - meters in this case.

Then we divide both sides of the equation by pi*5 to get r^2 by itself. So 12/(pi*5) = r^2. Take the square root of both sides and we have r = sqrt( 12/(pi*5) ), which is about 0.874 meters. The diameter is double the radius, so d = 2*r = 2*sqrt( 12/(pi*5) ), which is about 1.748 meters.

I hope you find this video helpful, and be sure to ask any questions down in the comments!

********************************************************************
The outro music is by a favorite musician of mine named Vallow, who, upon my request, kindly gave me permission to use his music in my outros. I usually put my own music in the outros, but I love Vallow's music, and wanted to share it with those of you watching. Please check out all of his wonderful work.

********************************************************************

+WRATH OF MATH+


Follow Wrath of Math on...

[perkinsat]

I put 5/2pie in my calculator and got a completely different answer. Even when I added the square error at the beginning I got a whole different number. How did you get 0.89?

[raminamiri]

How do you find the approximate of the square root. Ex: 5/2pi equals 0.89 meters how did you find the 0.89

[averytaylor]

This video was a whole lotta steps and u still left me confused

[sarahbjj]

I want to know how to get those numbers on the calculator. I do not understand how you get those numbers. I have got a lot of different numbers after doing it like 10 times and getting 10 different

[Freelcccprisoners]

What if I am give. The volume and I need to find the height when the height is equal to its diameter

[Dvsqt]

omg I love you . Thank you for this video.

[eqyusgg]

Sorry to say but you are not to the point...You mixed up soo many techniques in one equation. And extend it for no reason. The video shouldn't be long more then 5 minutes. You took about 8 minutes just to solve only one equation.

[ZuwairaUsman-pirx]

You can't be asked to find radius with only height given

[nicorequina]

It's not 0.89 it's 0.79 I guess

[charlenemayolmido]

What if the height is longer than its radius.