filmov
tv
Lecture 6(B): Logic 2 (Strategy for proofs)

Показать описание
Vacuously true statements and their use in proofs.
There's an issue in Parts B and C of Lecture 6 due to the sequencing of the lectures. These two parts of Lecture 6 use open sets in some examples -- and that's a problem, because open sets aren't introduced until Lecture 10, which I had recorded *before* the Logic lectures. Until I can fix this, I suggest that before doing Parts B and C of Lecture 6 you look ahead to Lecture 10 on open sets
There's an issue in Parts B and C of Lecture 6 due to the sequencing of the lectures. These two parts of Lecture 6 use open sets in some examples -- and that's a problem, because open sets aren't introduced until Lecture 10, which I had recorded *before* the Logic lectures. Until I can fix this, I suggest that before doing Parts B and C of Lecture 6 you look ahead to Lecture 10 on open sets
Lecture 6(B): Logic 2 (Strategy for proofs)
Lecture 6(C): Logic 2 (Strategy for proofs)
How to Answer Any Question on a Test
Logical Reasoning???#viral #vidumzn
Lecture 6(A): Logic 2 (Implication issues)
LSAT PrepTest 20 Logic Game 2 Full Tutorial (Questions 6-12)
The Simple Solution to Traffic
Dan Harmon Story Circle: 8 Proven Steps to Better Stories
Discrete Maths for Engineering - Lecture 5th ( operations on sets part 1 ) | SPPU 2nd Year CO \ IT
6 Logical reasoning questions to trick your brain
Logarithms, Explained - Steve Kelly
Supply and Demand Explained in One Minute
How To Solve Absolute Value Equations, Basic Introduction, Algebra
Digital Logic - implementing a logic circuit from a Boolean expression.
Propositional Logic: Proof Strategy and Reasoning
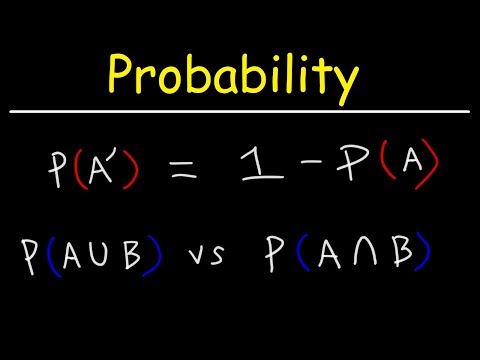
Probability of Complementary Events & Sample Space
Lecture 6: Time Evolution and the Schrödinger Equation
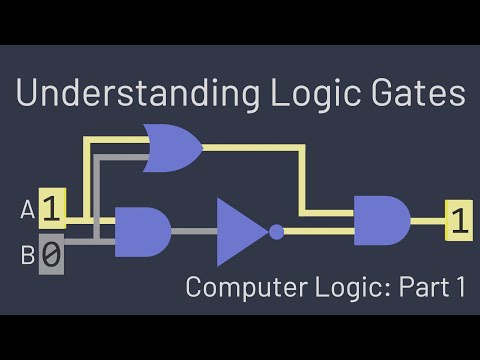
Understanding Logic Gates
Claims, Evidence, and Reasoning.
How to use BODMAS (Order of Operations) #2
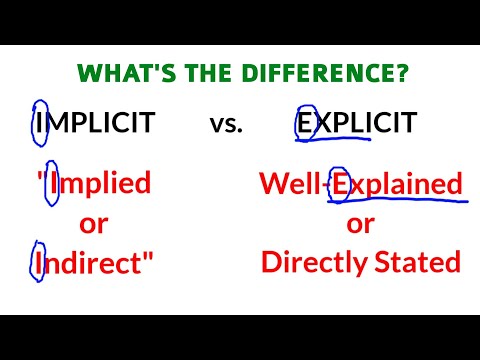
IMPLICIT vs EXPLICIT STATEMENT | Logical Reasoning
AFPSAT | ABSTRACT REASONING | Logical Test
This tool will help improve your critical thinking - Erick Wilberding
The Schwa - English In A Minute
Комментарии
 0:14:34
0:14:34
 0:31:19
0:31:19
 0:00:27
0:00:27
 0:00:11
0:00:11
 0:24:45
0:24:45
 0:15:53
0:15:53
 0:05:14
0:05:14
 0:08:57
0:08:57
 0:08:41
0:08:41
 0:02:36
0:02:36
 0:03:34
0:03:34
 0:00:54
0:00:54
 0:04:21
0:04:21
 0:08:03
0:08:03
 0:20:03
0:20:03
 0:05:48
0:05:48
 1:22:19
1:22:19
 0:07:28
0:07:28
 0:02:06
0:02:06
 0:06:20
0:06:20
 0:04:24
0:04:24
 0:11:35
0:11:35
 0:05:20
0:05:20
 0:01:02
0:01:02