filmov
tv
Given One Complex Zero Find the Remaining Zeros of a Polynomial

Показать описание
👉 Learn how to find all the zeros of a polynomial given one complex zero. A polynomial is an expression of the form ax^n + bx^(n-1) + . . . + k, where a, b, and k are constants and the exponents are positive integers. The zeros of a polynomial are the values of x for which the value of the polynomial is zero. Note that when a complex number is a factor of a polynomial, that the conjugate of the complex number is also a factor of the polynomial.
To find the zeros of a polynomial when one of the zeros is known, we use synthetic division to divide the polynomial with the given zero or we use long division to divide the polynomial with the given zero expressed in factor form. This gives the other factor of the polynomial, which can be further factored by dividing with the conjugate of the complex number and then further factored (if need be) using any of the techniques for factoring polynomials.
After we have factored the polynomial, we can then use the zero product property to evaluate the factored polynomial and hence obtain the remaining zeros of the polynomial. Recall that the zero-product property states that when the product of two or more terms is zero, then either of the terms is equal to 0.
Organized Videos:
✅Find all the Remaining Zeros Given a Factor or Zero
✅Find all the Remaining Zeros Given one irrational Zero
✅Find all the remaining zeros given one complex zero
✅Find all the remaining zeros given one rational zero
Connect with me:
#polynomials #brianmclogan
To find the zeros of a polynomial when one of the zeros is known, we use synthetic division to divide the polynomial with the given zero or we use long division to divide the polynomial with the given zero expressed in factor form. This gives the other factor of the polynomial, which can be further factored by dividing with the conjugate of the complex number and then further factored (if need be) using any of the techniques for factoring polynomials.
After we have factored the polynomial, we can then use the zero product property to evaluate the factored polynomial and hence obtain the remaining zeros of the polynomial. Recall that the zero-product property states that when the product of two or more terms is zero, then either of the terms is equal to 0.
Organized Videos:
✅Find all the Remaining Zeros Given a Factor or Zero
✅Find all the Remaining Zeros Given one irrational Zero
✅Find all the remaining zeros given one complex zero
✅Find all the remaining zeros given one rational zero
Connect with me:
#polynomials #brianmclogan
Комментарии
 0:04:08
0:04:08
 0:04:42
0:04:42
 0:04:56
0:04:56
 0:04:29
0:04:29
 0:07:13
0:07:13
 0:05:53
0:05:53
 0:07:48
0:07:48
 0:04:53
0:04:53
 0:31:26
0:31:26
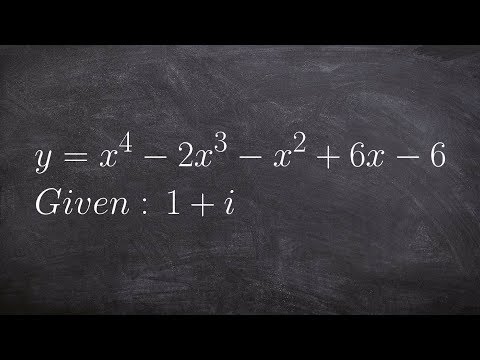 0:03:36
0:03:36
 0:04:18
0:04:18
 0:06:31
0:06:31
 0:05:22
0:05:22
 0:03:24
0:03:24
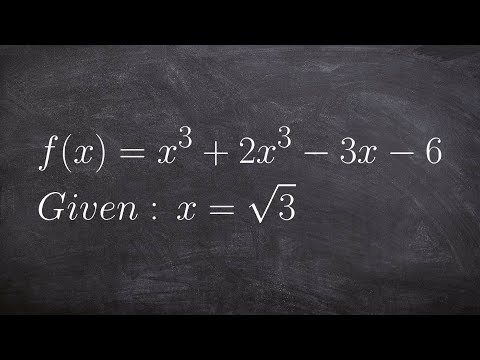 0:05:07
0:05:07
 0:04:28
0:04:28
 0:07:12
0:07:12
 0:03:50
0:03:50
 0:08:12
0:08:12
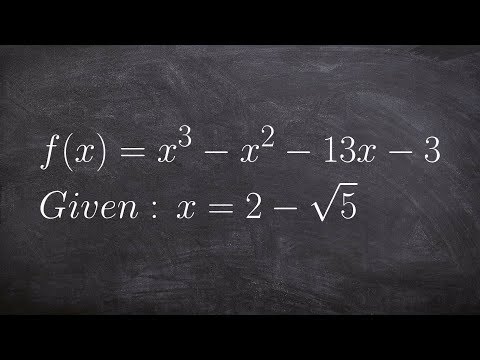 0:06:04
0:06:04
 0:03:56
0:03:56
 0:03:08
0:03:08
 0:04:06
0:04:06
 0:07:55
0:07:55