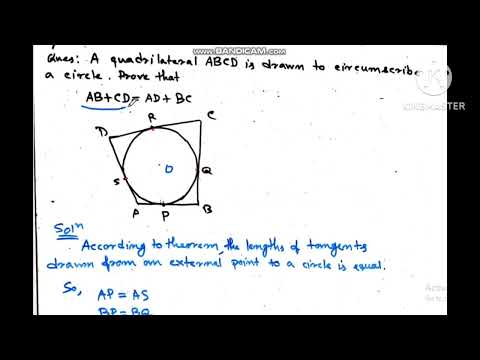
A quadrilateral ABCD is drawn to circumscribe a circle | Exercise 10.2 [Q8]

Exercise 10.2 Question 8 Class 10
A quadrilateral ABCD is drawn to circumscribe a circle (see Fig. 10.12). Prove that AB + CD = AD + BC

HINT :
Theorem 10.1 :
The tangent at any point of a circle is perpendicular to the radius through the point of contact

Theorem 10.2 :
The lengths of tangents drawn from an external point to a circle are equal.

Read it :
INTRODUCTION
A circle is a collection of all points in a plane which are at a constant distance (radius) from a fixed point (centre).
Different situations that can arise when a circle and a line are given in a plane.
(i) The line PQ and the circle have no common point. In this case, PQ is called a non-intersecting line with respect to the circle.
(ii) There are two common points A and B that the line PQ and the circle have. PQ a secant of the circle.
(iii) There is only one point A which is common to the line PQ and the circle line is called a tangent to the circle.

Tangent to a Circle
A tangent to a circle is a line that intersects the circle at only one point.
There is only one tangent at a point of the circle.
The tangent to a circle is a special case of the secant, when the two end points of its corresponding chord coincide.

The common point of the tangent and the circle is called the point of contact and the tangent is said to touch the circle at the common point

Note📝
The above question is taken from NCERT class 10 chapter Circles Exercise 10.2 Question 8.

Practice :
Two concentric circles are of radii 5 cm and 3 cm. Find the length of the chord of the larger circle which touches the smaller circle.

Introduction to circles and Exercise 10.1

Revise✍🏻:
Real Numbers:

Polynomials

Quadratic Equation

Trigonometry

Connect with us 🤩:

#circles #class10maths #ncert