filmov
tv
Cauchy sequences and complete metric spaces, Real Analysis II
Показать описание
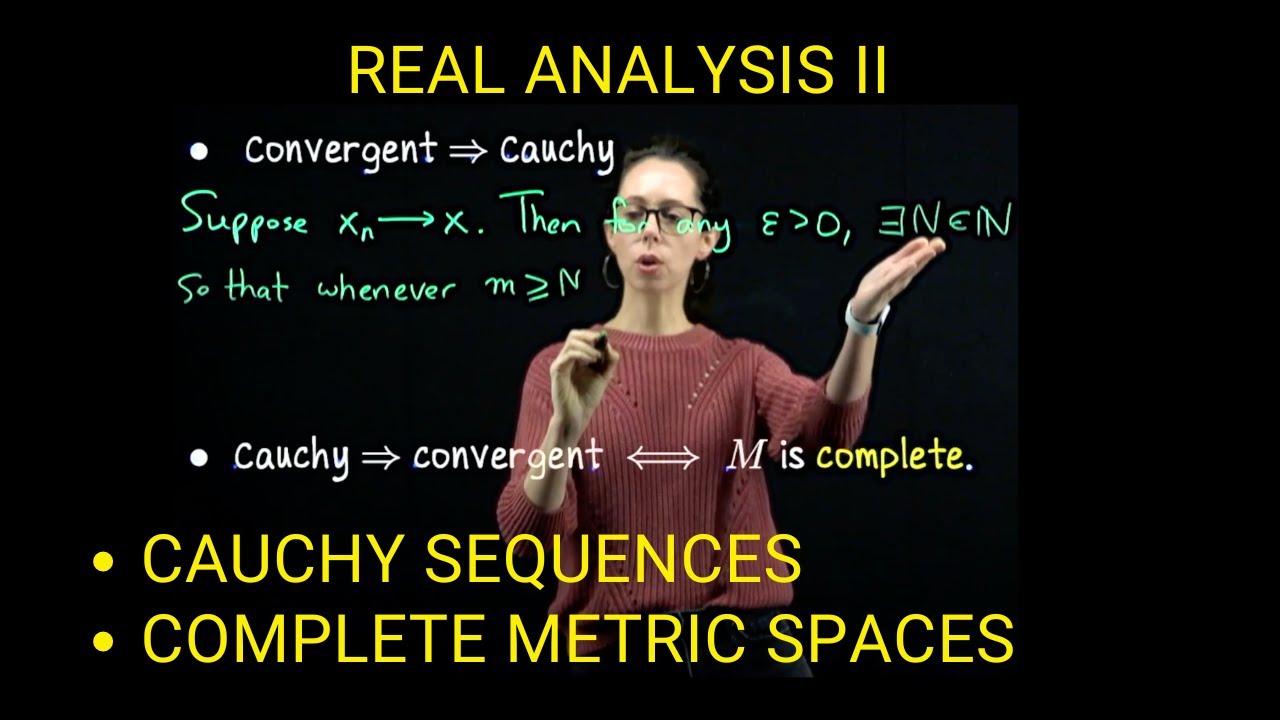
In this lecture, I introduce Cauchy sequences in metric spaces and explain how they help us define and understand complete metric spaces. A sequence is called Cauchy if, for any small distance epsilon, there is a point in the sequence beyond which all subsequent terms are within that distance from each other. This is similar to the idea of convergence, but instead of terms getting close to a limit, they are getting close to each other. I then show that all convergent sequences are Cauchy, but not all Cauchy sequences converge unless the space is complete.
Next, I illustrate these concepts with examples. In the rational numbers, which are not complete, I construct sequences that are Cauchy but fail to converge to a rational number, instead converging to an irrational number. I also examine the open unit ball in Euclidean space, which is not complete, as sequences can converge to a point outside the ball. Finally, I show that discrete metric spaces are always complete since any Cauchy sequence in such spaces must eventually become constant.
The lecture concludes with two key results. First, I prove that all Cauchy sequences are bounded, adapting the proof that convergent sequences are bounded by choosing an anchor point in the sequence. Second, I prove that any closed subset of a complete metric space is complete, showing that if a sequence converges in the larger space, its limit must lie within the closed subset.
#Mathematics #math #MetricSpaces #CauchySequences #advancedcalculus #RealAnalysis #Topology #MathLecture #AdvancedMath #MathTutorial #settheory
Next, I illustrate these concepts with examples. In the rational numbers, which are not complete, I construct sequences that are Cauchy but fail to converge to a rational number, instead converging to an irrational number. I also examine the open unit ball in Euclidean space, which is not complete, as sequences can converge to a point outside the ball. Finally, I show that discrete metric spaces are always complete since any Cauchy sequence in such spaces must eventually become constant.
The lecture concludes with two key results. First, I prove that all Cauchy sequences are bounded, adapting the proof that convergent sequences are bounded by choosing an anchor point in the sequence. Second, I prove that any closed subset of a complete metric space is complete, showing that if a sequence converges in the larger space, its limit must lie within the closed subset.
#Mathematics #math #MetricSpaces #CauchySequences #advancedcalculus #RealAnalysis #Topology #MathLecture #AdvancedMath #MathTutorial #settheory
Комментарии
 0:07:24
0:07:24
 0:32:01
0:32:01
 0:09:14
0:09:14
 0:07:29
0:07:29
 0:05:51
0:05:51
 0:03:39
0:03:39
 0:50:26
0:50:26
 0:10:51
0:10:51
 0:15:17
0:15:17
 0:22:46
0:22:46
 0:01:01
0:01:01
 0:07:38
0:07:38
 0:56:58
0:56:58
 0:13:47
0:13:47
 0:21:59
0:21:59
 0:17:43
0:17:43
 0:19:44
0:19:44
 0:00:10
0:00:10
 0:05:53
0:05:53
 0:19:13
0:19:13
 0:11:22
0:11:22
 0:01:53
0:01:53
 0:05:25
0:05:25
 0:21:52
0:21:52