Quadratic Reciprocity Law | Examples | Mersenne Number | Lecture-14

1. If p is prime of the form 8k+7 then M(p-1)/2 is congruent to 0 (mod p) where M(p-1)/2 is Mersenne number
2. If n is greater than 3 is prime of the form 4k+3 and p = 2n+1 is also prime then Mersenne number is composite
3. Find all prime p for which x^2 is congruent to 13 (mod p) is solvable
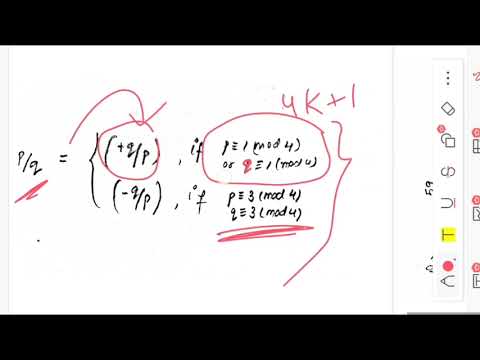
4. If p is prime and p = a^2 + n b^2 where a, b and n are integers , then prove that (a,b)=1 and (-n/p) =1
5. Let p and q are primes such that p is congruent to 3 (mod 4) , q = 2p+1 then show that q | 2^p -1
6. If a is a quadratic non residue of each odd prime p and q. Is x^2 is congruent to a (mod pq) solvable?