Axiomatics and the least upper bound property (I1) | Real numbers and limits Math Foundations 121

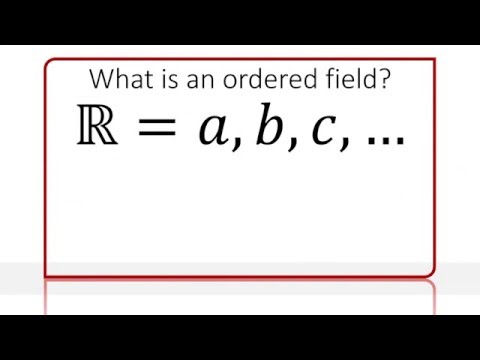
Here we continue explaining why the current use of `axiomatics' to try to formulate a theory of `real numbers' is fundamentally flawed. We also clarify the layered structure of the rational numbers: we have seen these several times already in prior discussion of the Stern- Brocot tree, here we view rationals in [0,1] in terms of increasing denominators. This allows us to explain why, if you believe the usual sad story of being able to `do an infinite number of operations', we can `create' nested interval sequences (or equivalently Cauchy sequences) with no rational limit.

This paves the way for introducing the much vaunted ``least upper bound property'' of `real numbers', which is a mainstay of classical analysis, and props up the modern theories of areas, integrals, infinite sums, transcendental functions, infinite products, lengths of curves and much more.

The reality is that much of modern mathematics, sadly, is an elaborate dream system. Here we are slowly, slowly....waking up. Feel free to join us, it's actually quite invigorating!

************************

Here are the Insights into Mathematics Playlists:

[pickeyberry]

Video Content
00:00 Introduction
4:59 Piggy backing
6:56 Nested intervals
9:22 Archimedean nested sequence of intervals
13:47 ?
16:00 Wished for properties of real numbers
18:42 If M is an upper bound
21:24 Least upper bound properties of real numbers

[alfonsoyfreddy]

Thank you that was very informative