filmov
tv
Griffiths QM Problem 4.9 (3rd ed.) Solving the FINITE Spherical Well for l=0
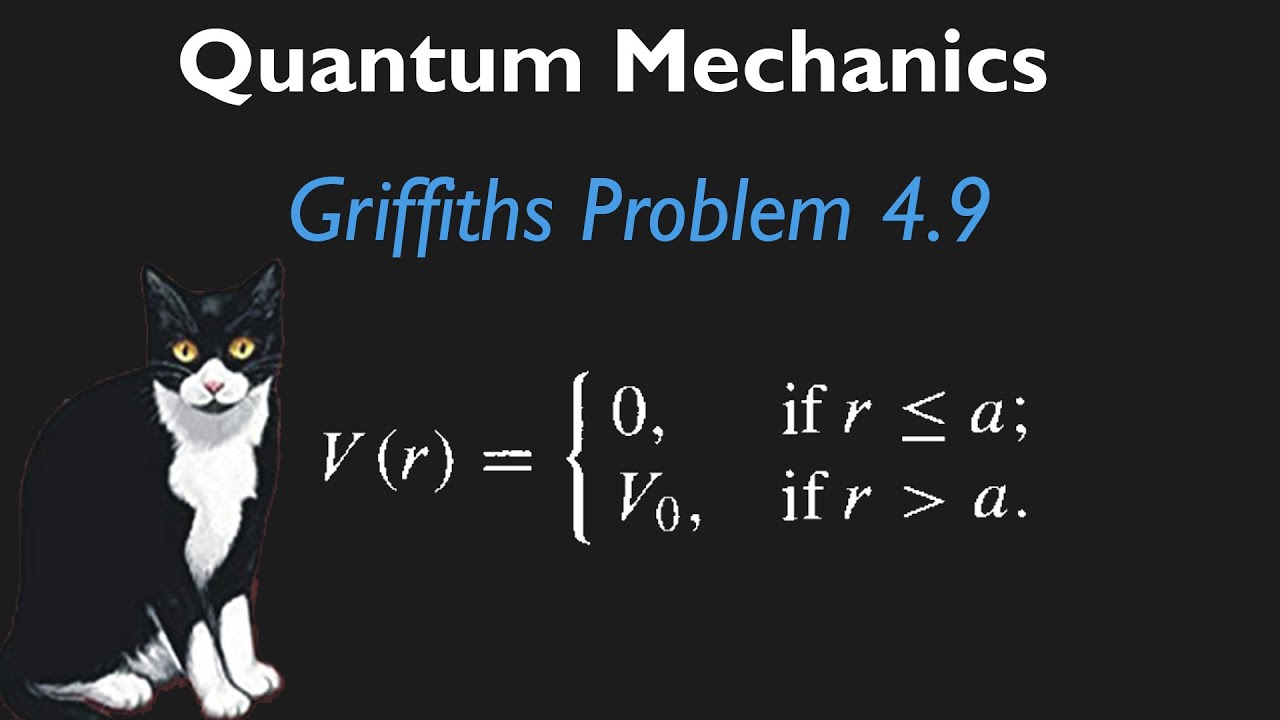
Показать описание
In this video I will solve problem 4.9 as it appears in the 3rd edition of griffiths introduction to quantum mechanics. The problem states:
a particle of mass m is placed in a finite spherical well.
find the ground state by solving the radial equation with l=0. Show that there is no bound state at all if ...
If you enjoy my content, please consider checking out my Patreon!
Also, consider subscribing and following me on my socials!
My name is Nick Heumann, I am a recently graduated physicist. I love to teach physics, so I decided to give YouTube a try. English is not my first language, but I hope that you can understand me well enough regardless.
▬ Contents of this video ▬▬▬▬▬▬▬▬▬▬
00:00 Introducing the problem
01:00 Finding the wavefunction in the inner region (V=0)
08:03 Finding the wavefunction in the outer region (V=V0)
11:37 Applying boundary conditions
13:00 Finding the transcendental equation
17:10 Graphing the equations
22:00 Finding the minimum value for V0 and a
a particle of mass m is placed in a finite spherical well.
find the ground state by solving the radial equation with l=0. Show that there is no bound state at all if ...
If you enjoy my content, please consider checking out my Patreon!
Also, consider subscribing and following me on my socials!
My name is Nick Heumann, I am a recently graduated physicist. I love to teach physics, so I decided to give YouTube a try. English is not my first language, but I hope that you can understand me well enough regardless.
▬ Contents of this video ▬▬▬▬▬▬▬▬▬▬
00:00 Introducing the problem
01:00 Finding the wavefunction in the inner region (V=0)
08:03 Finding the wavefunction in the outer region (V=V0)
11:37 Applying boundary conditions
13:00 Finding the transcendental equation
17:10 Graphing the equations
22:00 Finding the minimum value for V0 and a
Griffiths QM Problem 4.9 (3rd ed.) Solving the FINITE Spherical Well for l=0
Griffiths QM Problem 4.3: Constructing Spherical Harmonics for l=m=0 and l=2, m=1
Problem 4.3 (Part 1) | Introduction to Quantum Mechanics (Griffiths)
Griffiths QM Problem 3.4
Griffith Quantum Mechanics Solution 4.30 Spin Example
Griffiths QM Problem 3.9 and 3.10
Solving the Infinite Cubical Well: Griffiths QM Problem 4.2 (3rd edition) Solution FULLY EXPLAINED
Griffiths, Quantum Mechanics, Problems 1.1-1.4
Griffiths QM 1.14 Solution (HARD PROBLEM) - Expectation Values for Gaussian wavefunction
GS 2.37 Griffiths 3rd edition, quantum mechanics, Linear combination, expectation value
Griffiths QM problem 8.3 (3rd edition): Transmission Coefficient for Finite Square Well (WKB)
Griffiths Intro to QM Problem 9.1: Hydrogen Atom in Time dependent Electric field
GS 4.2 Griffiths 3rd edition Chapter 4 Problem 4.2, related to cubical infinite potential
Griffiths Quantum Mechanics 3rd Ed. | Problem 2.5(d)
Solution to Griffiths Introduction to QM Problem 6.31 (3rd edition) 6.36 (2nd edition) Stark effect
Griffiths QM Problem 1.10
3.12 , 3.13 solution | particle physics | Griffith | Chp 3 notes | particle physics solved problems
Problem 1.10 | Griffiths' Introduction to Quantum Mechanics | 3rd Edition
Griffiths QM Problem 3.2
Griffiths David J. - Solutions 4.19 - 4.20 - Introduction to Quantum Mechanics
Griffiths QM Problem 1.2: More Probability Practice
Finding Expectation Values - Griffiths Quantum Mechanic Problem 1.5 Part B
GS 2.5 Griffiths 3rd ed., quantum, Problem 2.5, square well, time dependent wave, linear combination
Quantum Mechanics - Problem 1.4 a | Griffiths QM
Комментарии
 0:25:16
0:25:16
 0:18:49
0:18:49
 0:09:21
0:09:21
 0:17:51
0:17:51
 0:14:28
0:14:28
 0:11:16
0:11:16
 0:37:30
0:37:30
 0:10:54
0:10:54
 0:19:34
0:19:34
 0:23:44
0:23:44
 0:09:56
0:09:56
 0:26:48
0:26:48
 0:21:16
0:21:16
 0:02:02
0:02:02
 1:13:00
1:13:00
 0:04:47
0:04:47
 0:00:53
0:00:53
 0:08:09
0:08:09
 0:06:38
0:06:38
 1:01:17
1:01:17
 0:12:11
0:12:11
 0:07:02
0:07:02
 0:26:32
0:26:32
 0:09:45
0:09:45