'Groupoid-Equivariant Index Theory' by Zachary Garvey

GroupoidFest 2021

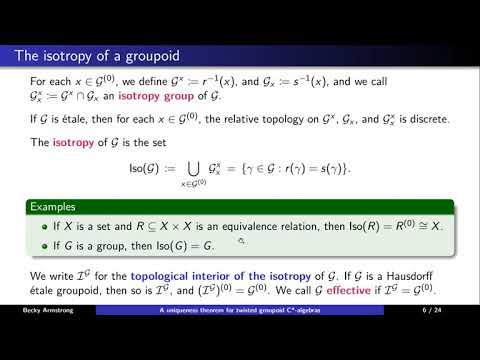
ABSTRACT: Index theory, from a K-homological point of view, is a factorization of the (analytic) K-homology class of a Fredholm operator, [P], into the KK-product of "topologically-defined" classes. For an elliptic pseudodifferential operator, P, on a compact manifold X , there is a factorization: [P ] = [σ_P ] ⊗ [D_{TX} ], where σ_P is determined by the principal symbol of P , and D_{TX} is a Dirac operator on the spin^c manifold TX. Given a groupoid with some conditions, G, we consider analogues of this factorization for G-equivariant elliptic pseudodifferential operators on a proper, cocompact G-manifold.