filmov
tv
Find the Volume of Spheres using Integral Calculus

Показать описание
Hi friends! This video is about deriving and finding the volume of a sphere by using integral calculus!
The formula for the volume of any shape is the definite integral from one end of the shape (let's call it "a") to the other end of the shape (let's call this "b"). So the integral is from a to b of the area of the cross-section (If you cut or slice the shape, you find the area of the open surface) and then dx.
Here, the area of the cross-section of the sphere is the circle! The area of a circle is pi times the radius squared. From the figure shown, the radius is y. Using the Pythagorean theorem, we get y = (r^2-x^2)^0.5. Plugging this into the area of the circle, we get A = pi(r^2-x^2). Now, we substitute this into the integral from -r to r and solve for it. Done.
In the next video, I will show you how to find the volume of a cone using integration.
LIKE & SUBSCRIBE:
MY EQUIPMENT:
✅Camera: Samsung Galaxy J7
✅Editing Software: Shotcut
The formula for the volume of any shape is the definite integral from one end of the shape (let's call it "a") to the other end of the shape (let's call this "b"). So the integral is from a to b of the area of the cross-section (If you cut or slice the shape, you find the area of the open surface) and then dx.
Here, the area of the cross-section of the sphere is the circle! The area of a circle is pi times the radius squared. From the figure shown, the radius is y. Using the Pythagorean theorem, we get y = (r^2-x^2)^0.5. Plugging this into the area of the circle, we get A = pi(r^2-x^2). Now, we substitute this into the integral from -r to r and solve for it. Done.
In the next video, I will show you how to find the volume of a cone using integration.
LIKE & SUBSCRIBE:
MY EQUIPMENT:
✅Camera: Samsung Galaxy J7
✅Editing Software: Shotcut
Volume of a Sphere | MathHelp.com
Volume of a Sphere
Visualizing the Volume of a Sphere Formula | Deriving the Algebraic Formula With Animations
The Volume of a Sphere - Numberphile
GCSE Maths - Calculate Volume of Spheres and Hemispheres #111
Volume of a sphere | Perimeter, area, and volume | Geometry | Khan Academy
How do you find the volume of a sphere
Volume of a Sphere
Want to Ace Surface Area and Volume?Exercise 12.1 (Q8, Q9) & Exercise 12.2 (Q1 to Q3) Class 10 M...
Let’s Find The Volume of a Sphere….Step-by-Step….
HOW TO FIND THE VOLUME OF A SPHERE
volume of spheres (KristaKingMath)
Volume of a Hypersphere
How to Figure Out the Radius of a Sphere Without Knowing the Volume : Science & Math
Find the Volume of a Sphere
How to Find the Volume of a Sphere
Math Antics - Volume
Find the Volume of Spheres using Integral Calculus
Volume of a Sphere - Corbettmaths
Cylinder, Cone, and Sphere Volume
Sphere Volume Formula | Math Animation
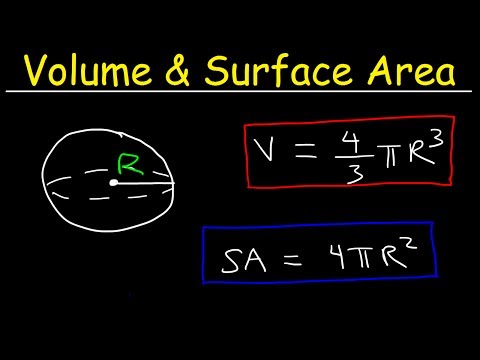
Volume and Surface Area of a Sphere Formula, Examples, Word Problems, Geometry
How to Find the Volume of a Sphere with Diameter
Volume of Spheres - Solid Figures in Geometry @MathTeacherGon
Комментарии
 0:01:57
0:01:57
 0:01:27
0:01:27
 0:03:12
0:03:12
 0:04:14
0:04:14
 0:03:55
0:03:55
 0:02:35
0:02:35
 0:03:22
0:03:22
 0:04:55
0:04:55
 0:41:16
0:41:16
 0:13:29
0:13:29
 0:02:21
0:02:21
 0:05:03
0:05:03
 0:01:00
0:01:00
 0:02:36
0:02:36
 0:01:49
0:01:49
 0:03:30
0:03:30
 0:12:36
0:12:36
 0:06:24
0:06:24
 0:07:07
0:07:07
 0:02:32
0:02:32
 0:01:27
0:01:27
 0:11:09
0:11:09
 0:03:27
0:03:27
 0:10:30
0:10:30