filmov
tv
Limit of absolute value functions [ lim |x^3 - x|/(x^3 - |x|) as x goes to 0 ]
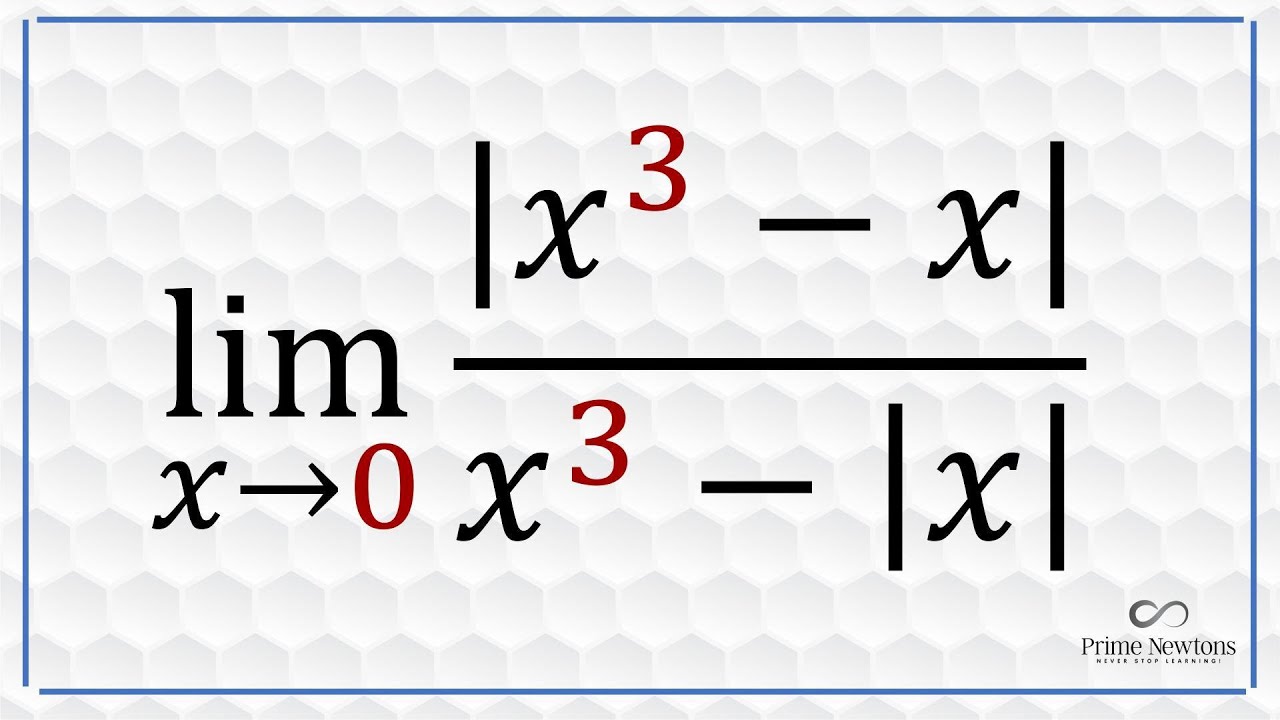
Показать описание
In this video , I showed how to compute the limit of all absolute value functions
Limits and Absolute Value
Limit of Absolute Value Function
Limit of Absolute Value Function (II)
Limit of absolute value functions [ lim |x^3 - x|/(x^3 - |x|) as x goes to 0 ]
Learn to evaluate the limit of the absolute value function
Limits of Absolute Value Functions
Algebraic Limits: Absolute Value
Limit of Function with Absolute Values Involved TIPS
Prove using epsilon-delta: Limit of (2x^2 - 3x + 1)/(x^3 + 4) = 1/4 as x approaches 2
Limit of Absolute value Functions
Limits Involving Absolute Value (AP Calc)
Find the One-Sided Limit with Absolute Value: |x|/x as x approaches zero from the Left #shorts
Limits involving Absolute Value | Limits and Absolute Value | Calculus
How to Compute a One Sided Limit with an Absolute Value
Calculus I: Limit with Absolute Value (Hard Example)
Finding One-Sided Limits of Piecewise and Absolute Value Functions | Calculus
Finding Limits of Functions with Absolute Value | Calculus 1 | Math with Professor V
Limit of Expression with Absolute Function and Graph
Limits in Absolute Value Functions - (Limits - 3)
Finding Limits of Functions with Absolute Value | Calculus 1 | Math with Professor V
Limits Involving Absolute Value Functions Calculus 1 AB
Absolute Value Limit: x/|x| as x approaches zero from the left #shorts
Determine Limit Involving Absolute Function
Limits with Absolute Value Functions
Комментарии
 0:17:44
0:17:44
 0:07:37
0:07:37
 0:05:05
0:05:05
 0:11:13
0:11:13
 0:02:19
0:02:19
 0:12:32
0:12:32
 0:10:45
0:10:45
 0:07:57
0:07:57
 0:11:11
0:11:11
 0:05:31
0:05:31
 0:07:44
0:07:44
 0:00:45
0:00:45
 0:19:01
0:19:01
 0:05:16
0:05:16
 0:08:17
0:08:17
 0:08:19
0:08:19
 0:18:06
0:18:06
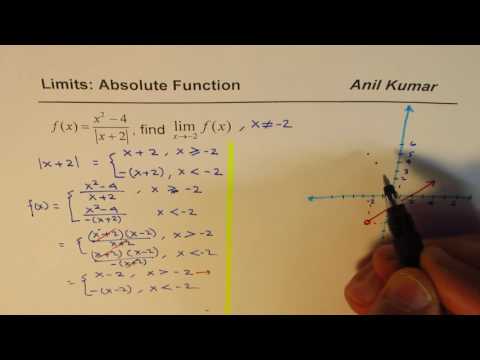 0:09:15
0:09:15
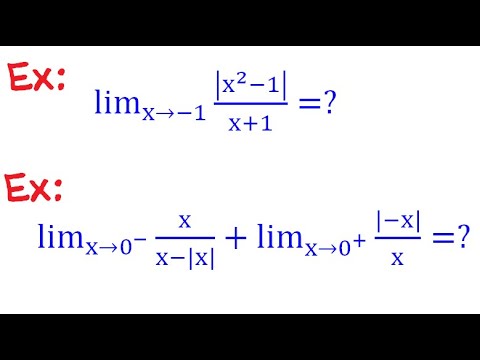 0:12:58
0:12:58
 0:20:33
0:20:33
 0:16:19
0:16:19
 0:00:49
0:00:49
 0:09:13
0:09:13
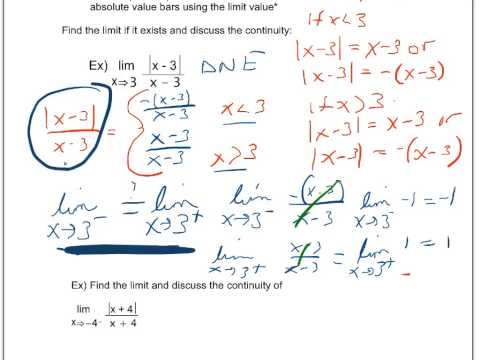 0:09:18
0:09:18