filmov
tv
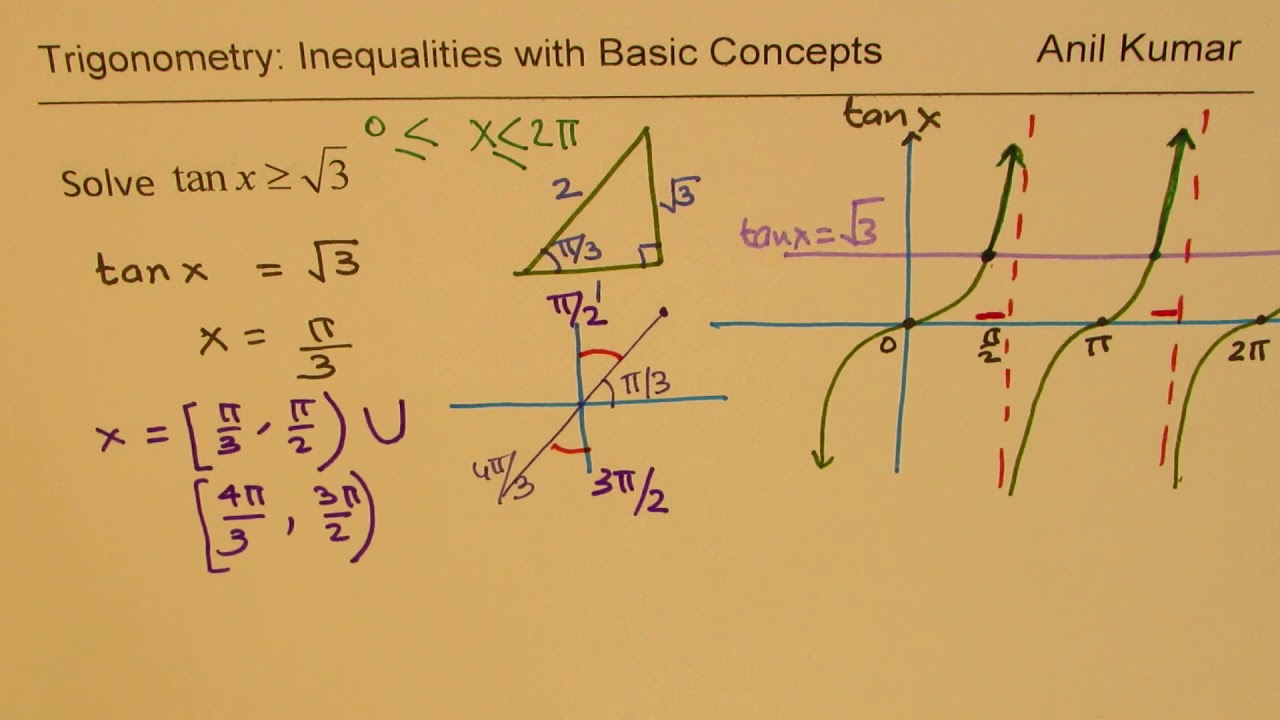
Solve Trigonometric Inequalities with Help of Graph and Basic Concepts
Показать описание
How to Solve Trigonometric Inequalities
Solve Trigonometric Inequalities with Help of Graph and Basic Concepts
5_3 Lesson Solving Trigonometric inequalities
Trigonometric Inequalities
Trigonometric Inequality | Using Calculus to Develop Trigonometric Inequalities
Trigonometric Inequality cos(3x - pi/4) greater than 0
sinx greater than tanx solution of Trigonometric Inequality
solving trigonometric inequalities
Topic 3 Review (3) for Solving Trig Inequalities
Solving Trig Inequalities
Trigonometric Inequality 2cos^2x+sinx less than 2 IIT JEE
Can You Solve These Trigonometric Inequalities? | Class 11, 12 | General Mathematics | #jee #maths
Solving Trig Inequalities
Topic 3 Review (4) Solving Trig Inequalities
Trigonometric Inequality Absolute value of cosine x less than half
Trigonometric Inequality cos x less than minus half
Trig Inequalities
Trigonometric Inequalities
JEE Mathematics | Trigonometric Inequalities | Theory | In English | By Misostudy
Tricks to solve trigonometric inequalities.
Trigonometric Inequalities
Solving Trig. Inequalities
Trigonometric inequalities | JEE, ISI, CMI | Simplification | Substitution | Polynomial | Induction
Calculus - Section 7.C (Trig Inequalities)
Комментарии
 0:06:44
0:06:44
 0:33:10
0:33:10
 0:09:49
0:09:49
 0:00:28
0:00:28
 0:06:24
0:06:24
 0:07:53
0:07:53
 0:09:56
0:09:56
 0:05:50
0:05:50
 0:15:01
0:15:01
 0:10:05
0:10:05
 0:08:16
0:08:16
 0:04:10
0:04:10
 0:07:36
0:07:36
 0:14:55
0:14:55
 0:08:52
0:08:52
 0:03:53
0:03:53
 0:18:06
0:18:06
 0:06:50
0:06:50
 0:43:32
0:43:32
 0:05:25
0:05:25
 0:04:11
0:04:11
 0:10:54
0:10:54
 1:17:36
1:17:36
 0:04:10
0:04:10