Can You Solve this Geometry Puzzle?

preview_player
Показать описание
There are 5 semicircles and the three below have a diameter of 4. Can you find the total red area?

This geometry puzzle was asked by Twitter user @Cshearer41. Please give her a follow if you enjoy geometry puzzles.

Subscribe!
  1. Dr. Weselcouch
  2. Geometry Puzzle
  3. Competition
  4. Math
  5. Geometry
Рекомендации по теме
Can you solve 0:06:09

Can you solve this Geometry Puzzle?

Can you solve 0:09:46

Can you solve these geometry problems?

Geometry Problem | 0:00:44

Geometry Problem | Finding the Missing Angle | SAT Prep | Math Problem

Geometry everyone should 0:00:15

Geometry everyone should learn

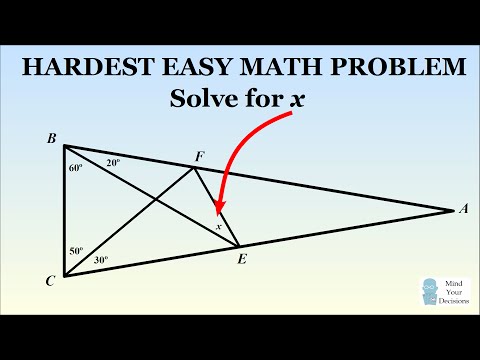
How To Solve 0:08:05

How To Solve The Hardest Easy Geometry Problem

Missing Angles Geometry 0:00:37

Missing Angles Geometry Problem | Tricky Math Question | JusticeTheTutor #maths #math #shorts

Can you find 0:06:27

Can you find the red area? – Geometry puzzle

Solving a geometry 0:03:42

Solving a geometry question that no one can figure out

Geometry: Can You 0:04:19

Geometry: Can You Solve for x Using Angle Rules? - SAT Math

Geometry | Find 0:00:16

Geometry | Find the angle #math #tutor #mathtrick #learning #geometry #angles #x

Can you solve 0:10:12

Can you solve this interesting geometry challenge?

■ Can you 0:09:43

■ Can you solve this Geometry question? | Out the box thinking 🧠 Geometry Q2 📐

Incredible trick to 0:05:39

Incredible trick to solve this geometry problem

Can You Find 0:08:44

Can You Find Angle X? | Geometry Challenge!

Can You Solve 0:04:13

Can You Solve This Grade 12 Geometry Problem From Australia? The Marshmallow Chocolate Puzzle

Geometry you need 0:00:55

Geometry you need to know for college

VERY HARD South 0:12:13

VERY HARD South Korean Geometry Problem (CSAT Exam)

Clever method to 0:03:15

Clever method to solve a tricky geometry problem!

Find Missing Side 0:00:48

Find Missing Side Length Using SohCahToa Trig in Geometry

Can you find 0:09:06

Can you find the diagonal length X? | (Semicircle) | #math #maths | #geometry

If you can 0:05:58

If you can solve this 3d geometry problem, you are a genius

Can you solve 0:04:12

Can you solve these viral geometry problems?

Want to PASS 0:14:08

Want to PASS Geometry? You better know this…

That's How You 0:00:54

That's How You Solve a Geometry Problem!

Комментарии
Автор

Will definitely be using the phrase "the messes cancel" in future.

elijahjoel
Автор

Interesting how the area is independent of the point taken on the middle semicircle, not an intuitive fact. I also like how you don't go too slow like other youtubers who explain every step, your pace is just perfect. Excellent solution, excellent problem.

enejidjsi
Автор

Very nice, great video!
What I did is I chose a point on the middle semicircle that was also on the line, so I could see the area would be two semi circles with diameters on the line. Since one diameter was two segments of 4 and the other was 1 segment, I knew that one had a diameter of 8 and the other had a diameter of 4. Then I just used the formula for a circle on both added them and divided by 2, (4^2+2^2)PI/2. However this doesn't tell you if the area is the same for any point you choose on the circle, but I just assumed that was true because we were asked to find the area and not a range of areas.

adrienyts
Автор

Keep doing your interesting videos!👍

francisconavarromolina
Автор

Great explanation
i thought the 2 point of 2 semicircle met at the center of the middle semicircle, but it just no matter because the answer will be the same for all point in the middle semicircle

phjvrsh
Автор

I cheated a bit. I chose the point where the middle semicircle intersects the line. In that specific case, it's pretty easy to see that the two semicircles have diameters of 8 and 4, which give a combined area of 10pi. Since you didn't give a specific point on the middle semicircle, I assumed it was true in general.

RunstarHomer
Автор

First one here again, I like that problem BTW








Damn u r so awesome...

HolyG-sus
Автор

I was about to ask where you got the pi over 8 from, but then I realized that you were talking about diameter and not radius! Stupid formulas that get stuck in your head!

oliverhb